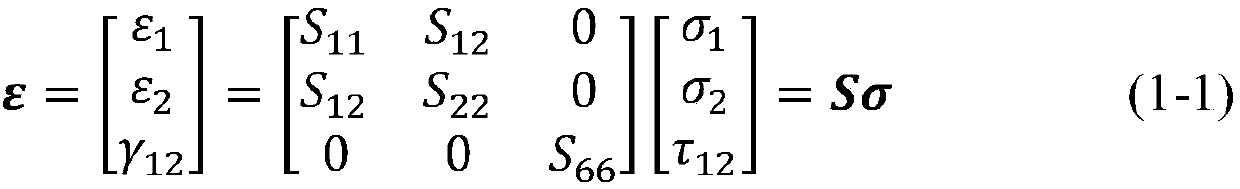Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

Calculation of equivalent modulus of composite laminates and strength verification under given load

A composite material layer and equivalent modulus technology, applied in calculation, design optimization/simulation, special data processing applications, etc., can solve the problem that the finite element analysis results cannot correctly and intuitively reflect the stress of the laminated board

Active Publication Date: 2018-12-11
WEIHAI GUANGWEI COMPOSITES
View PDF2 Cites 9 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

This concentrated stress makes the finite element analysis results unable to reflect the force of the laminated plate directly and correctly, and according to Saint-Venant’s principle, this force is only a local effect and has no effect on the force of the overall structure

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Calculation of equivalent modulus of composite laminates and strength verification under given load
  • Calculation of equivalent modulus of composite laminates and strength verification under given load
  • Calculation of equivalent modulus of composite laminates and strength verification under given load

Examples

Experimental program
Comparison scheme
Effect test

Embodiment 1

[0060] Embodiment 1: It includes an information input module, an operation module and an information output module. After the data is input and run, the calculation program will first calculate the stiffness matrix of each single layer according to the data input in the information input module, and then integrate the laminate stiffness matrix according to the ply information. From the laminate stiffness matrix, the equivalent modulus and equivalent stiffness matrix of the laminate under pure in-plane load and pure bending torsional load can be calculated, and the overall strain can be obtained by combining the load information, and further combined with the ply information to obtain the According to the maximum stress criterion, the Tsai-Hill failure criterion and the Tsai-Wu failure criterion, it is judged whether the laminate fails under the specified load.

[0061] In the equivalent modulus and strength check calculation program, the information included in the information...

Embodiment 2

[0114] Embodiment 2: on the basis described in embodiment 1, in order to satisfy the situation that part has requirement to structural rigidity, increase output structural rigidity data, include:

[0115] a. Tensile stiffness EA:

[0116]

[0117] Among them, EA is the tensile stiffness, E is the modulus of elasticity, A is the cross-sectional area; F is the tensile force, l is the length of the rod, and ΔL is the elongation of the rod.

[0118] b. Bending stiffness EI:

[0119]

[0120] Where EI is the bending stiffness, E is the modulus of elasticity, I is the moment of inertia of the section about the rotation axis; F is the concentrated force at the rod end of the cantilever, l is the length of the rod, and ω is the deflection of the rod end.

[0121] c. Torsional stiffness GI p :

[0122]

[0123] where GI p is the torsional stiffness, G is the shear modulus, I p is the polar moment of inertia; T is the torque, l is the length of the member, is the relativ...

Embodiment 3

[0125] Embodiment 3: On the basis described in Embodiment 2, in order to facilitate professional computing personnel to check the calculation details, increase the output A, D, B matrix information, the stress-strain information σ under the x-y coordinates of each layup and the 1-2 coordinates x , σ y , τ xy , σ 1 , σ 2 , τ 12 . However, it should be noted that due to the data type in the calculation process, only 4 digits after the decimal point are reserved for the short type, so the corresponding calculation results will produce data deviations, and the data 0 will often be calculated as 10 -10 Numbers below the order of magnitude.

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention relates to the field of composite material design, in particular to calculation of equivalent modulus of composite laminates and strength verification under a given load. The invention comprises an information input module, an operation module and an information output module. Material property information, laminating information and load data information are input into the information input module. Three stiffness matrices A, D, B of composite laminates are calculated according to the input data by a computing program, and the equivalent modulus and stiffness matrices of composite laminates under pure in-plane load and pure bending-torsion load are calculated according to the matrix A and matrix D. At the same time, according to the stiffness matrix and load information, theoverall strain and deflection rate of the laminate can be calculated, and the stress and strain information of each single layer can be obtained. Through adoption of three composite failure criteria,whether the laminate meets the strength requirements under the specified load can be checked. The invention greatly improves the calculation speed, and the accurate numerical solution of stress and strain can be directly and quickly obtained for the analysis of the laminated plate under the simple stress condition, and the design efficiency is improved.

Description

technical field [0001] The invention relates to the field of structural design of composite materials, in particular to a program for calculating the equivalent modulus of a composite material laminate and checking the strength under a given load. Background technique [0002] As we all know, in the process of finite element analysis of composite materials, due to the complexity of the structure of composite materials and the anisotropy of materials, it is often necessary to input cumbersome ply information when defining material properties, especially for those with many plies or complex When analyzing structural composite parts, it takes a lot of time and cost to assign material properties. Since there is no tension-bending and tension-torsion coupling in the symmetrically balanced ply composite laminate, and the tension-shear coupling coefficient is small, the process of assigning material properties is simplified by the equivalent modulus method, which saves a lot of tim...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
Patent Type & Authority Applications(China)
IPC IPC(8): G06F17/50
CPCG06F30/20Y02T90/00
Inventor 丛庆曾秋云倪亭隋显航殷飞
Owner WEIHAI GUANGWEI COMPOSITES
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com