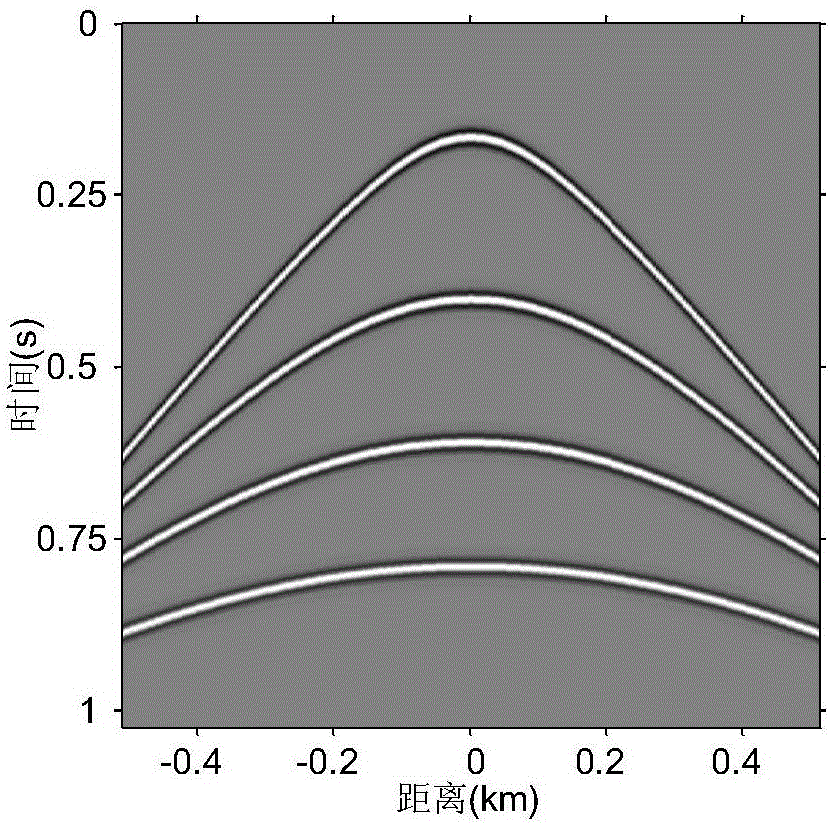
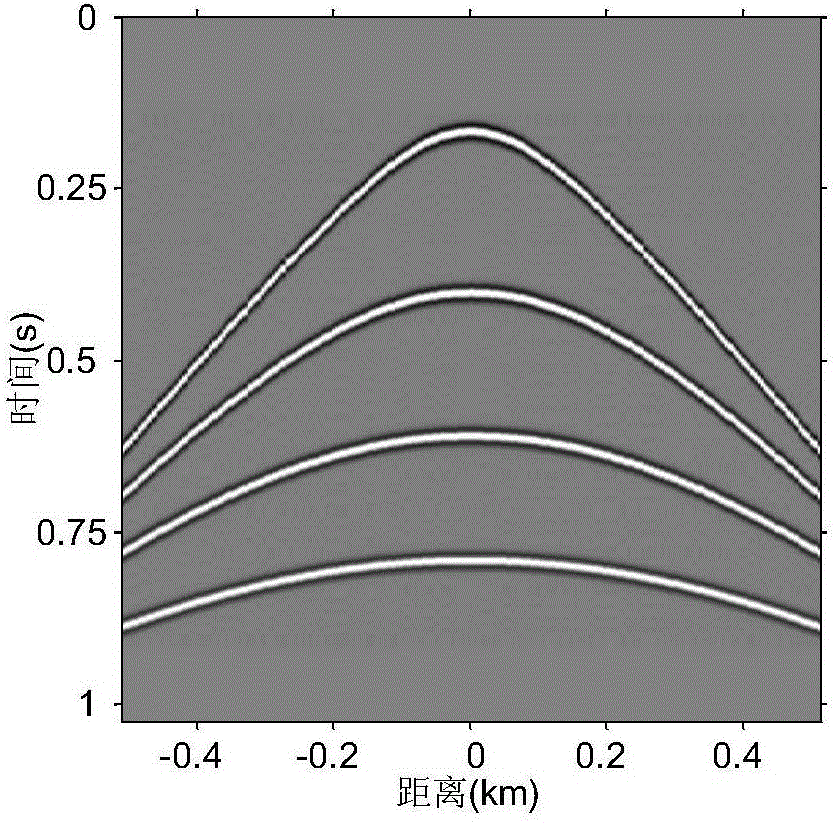
High-precision seismic data reconstruction method based on two-dimensional non-uniform curvelet transform
A technology of seismic data and curvelet transformation, applied in seismology, seismic signal processing, geophysical measurement, etc., can solve the problems of limited application, seismic data cannot be directly reconstructed, seismic trace missing reconstruction, etc.
- Summary
- Abstract
- Description
- Claims
- Application Information
AI Technical Summary
Problems solved by technology
Method used
Image
Examples
Embodiment 1
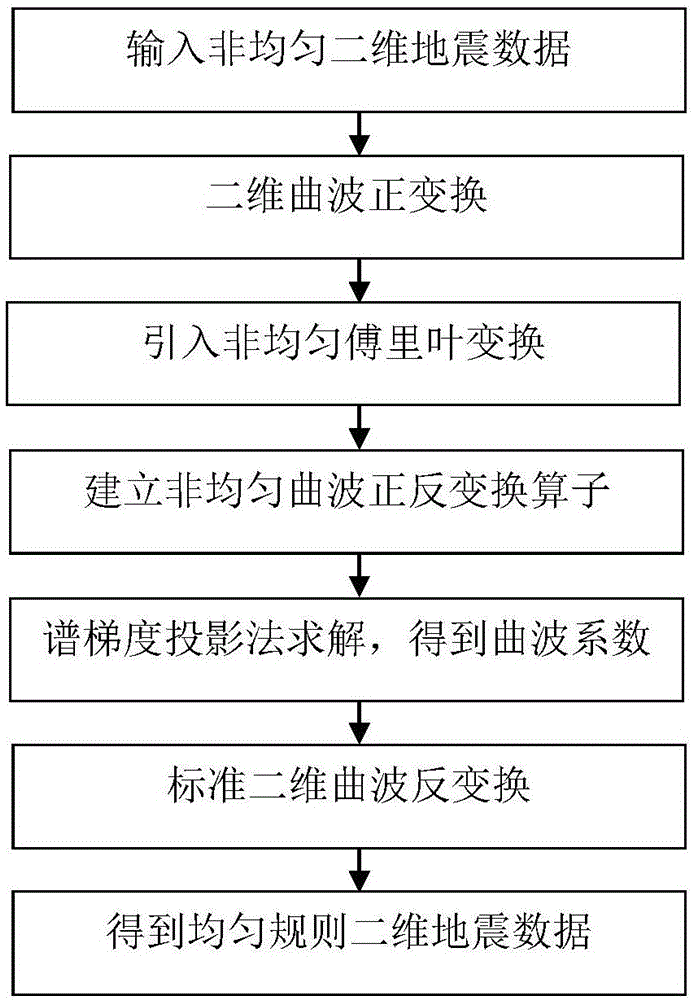
[0066] The steps to implement this method mainly include conventional two-dimensional curvelet forward and inverse transformation, non-uniform Fourier transform, establishment of non-uniform curvelet forward and inverse transformation operator, spectral gradient projection method, etc. Specific steps are as follows:
[0067] Step 1: Two-dimensional curve wave positive and negative transformation. In order to obtain high-precision seismic data reconstruction results, it is necessary to perform data reconstruction on the basis of two-dimensional curvelet transform. The two-dimensional curvelet positive transform is defined as:
[0068] c ( j , l , k ) = d , ψ j , l , k > = ∫ R 2 d ( x ) ψ j , l , k ( x ) ‾ d x
[0069] Where: ψ j,l,k Represents the curvelet function, c(j,l,k) is the curvelet coefficient, j,l,k represent the scale, direction and location parameters respectively, d(x...
PUM
 Login to View More
Login to View More Abstract
Description
Claims
Application Information
 Login to View More
Login to View More - R&D
- Intellectual Property
- Life Sciences
- Materials
- Tech Scout
- Unparalleled Data Quality
- Higher Quality Content
- 60% Fewer Hallucinations
Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.
© 2025 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com