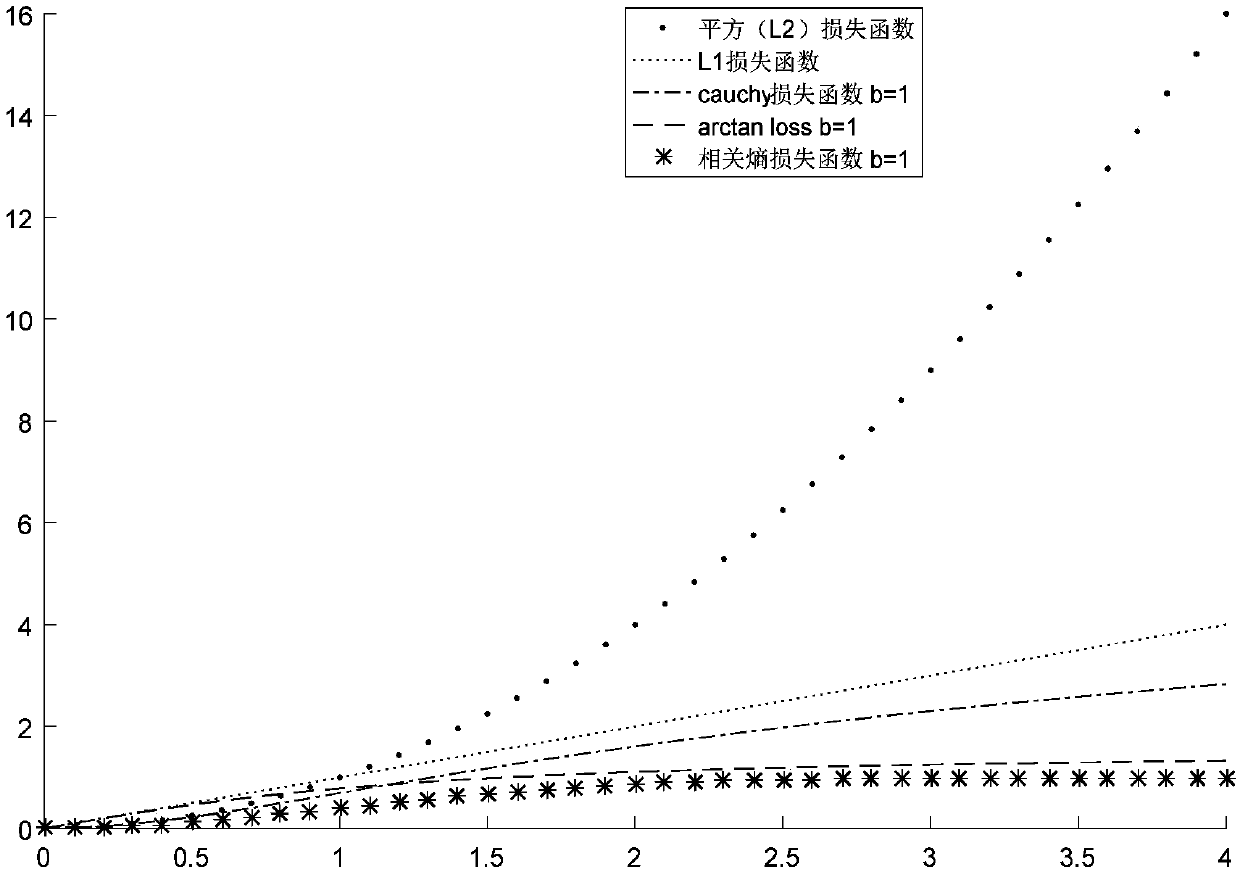
Robust Estimation of Fundamental Matrix of Torr-m-estimators Based on Correlation Entropy
A basic matrix and robust estimation technology, applied in computing, computer components, image analysis, etc., can solve problems such as low accuracy, long computing time, and poor results, and achieve improved accuracy and robustness. Effect
- Summary
- Abstract
- Description
- Claims
- Application Information
AI Technical Summary
Problems solved by technology
Method used
Image
Examples
Embodiment Construction
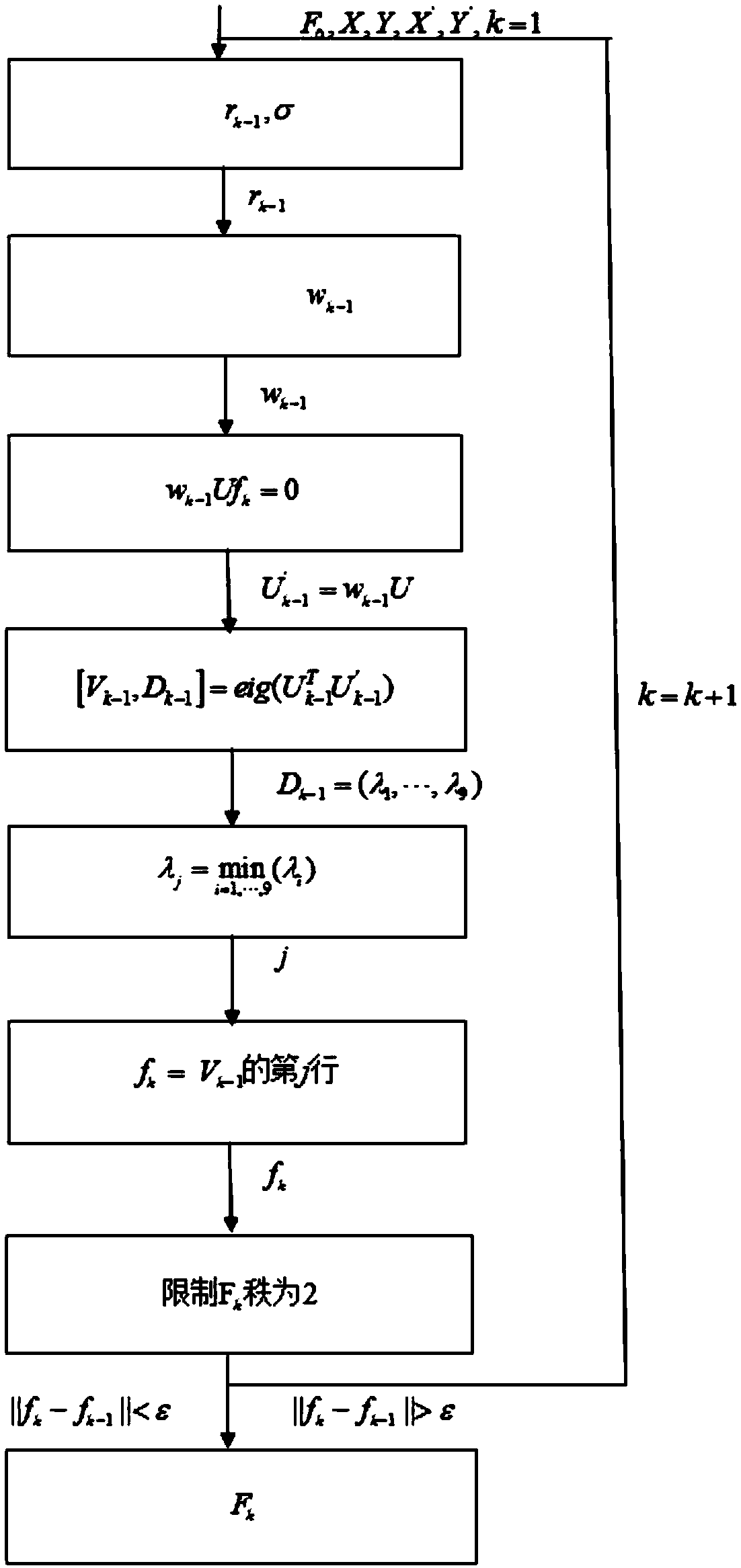
[0032] Details in each step of the present invention will be described in detail below in conjunction with the accompanying drawings.
[0033] The present invention proposes a Torr-M-Estimators fundamental matrix robust estimation method based on correlation entropy, such as figure 1 As shown, it specifically includes the following steps:
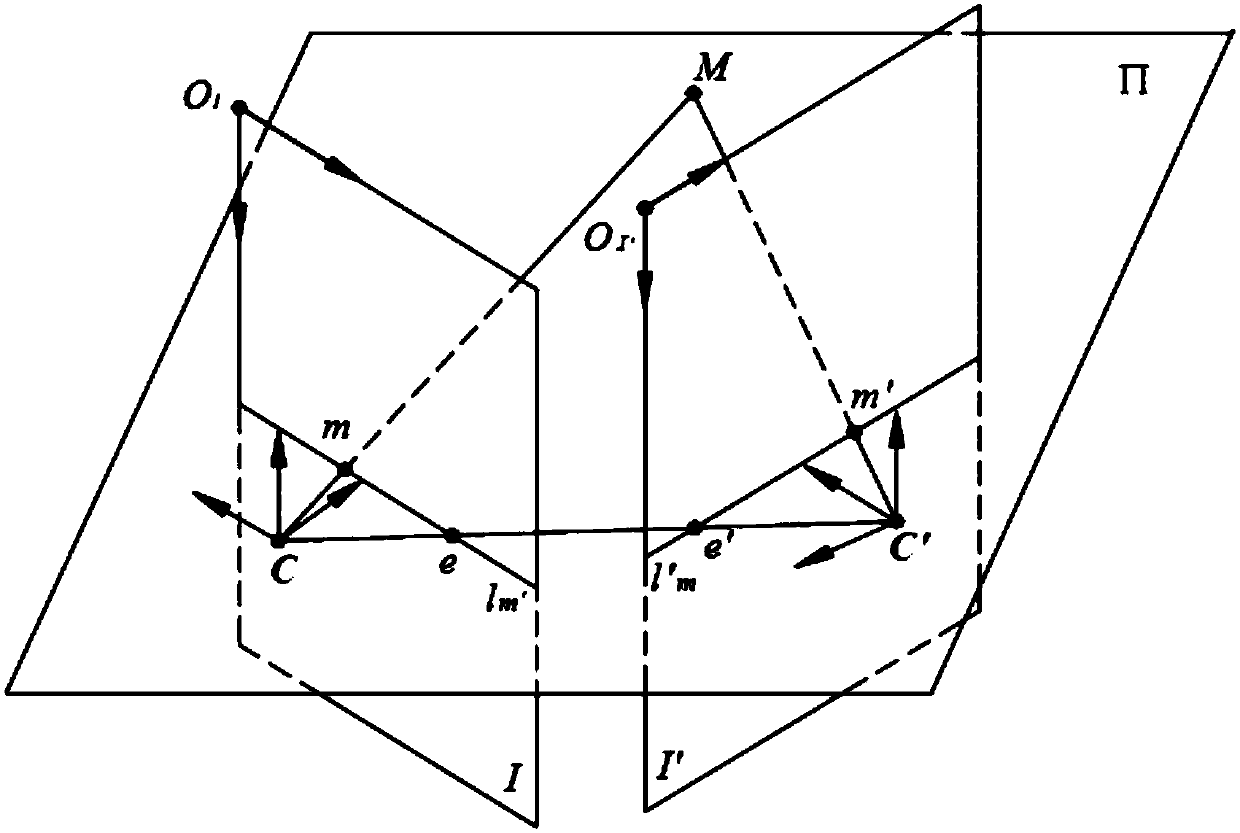
[0034] Step 1: For input such as Figure 4 Two images from different perspectives of the same scene shown, Figure 4 -a is the image of the first perspective, Figure 4 -b is the image corresponding to the second viewing angle. The present invention extracts SIFT feature points as a feature point description operator, and the result is as follows Figure 5 as shown, Figure 5 -a, 5-b represent the SIFT feature points corresponding to the two perspectives. Using the RANSAC method for feature point matching, the results are as follows Image 6 As shown, at this time, according to the geometric relationship, the corresponding relations...
PUM
 Login to View More
Login to View More Abstract
Description
Claims
Application Information
 Login to View More
Login to View More - R&D
- Intellectual Property
- Life Sciences
- Materials
- Tech Scout
- Unparalleled Data Quality
- Higher Quality Content
- 60% Fewer Hallucinations
Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.
© 2025 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com