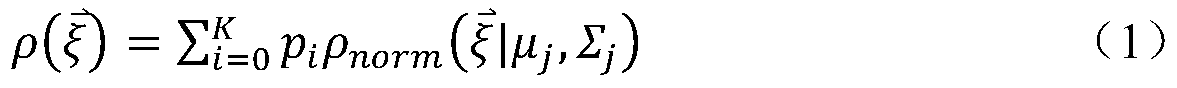MEMS device uncertainty analysis method based on Nataf transformation
A technology of uncertainty and analysis methods, applied in the field of computer simulation of micro-electromechanical systems, it can solve the problems of not satisfying the Gaussian distribution, difficult to express the distribution form in analytical form, lack of research, etc., achieving easy implementation, simple form, easy to obtain Effect
- Summary
- Abstract
- Description
- Claims
- Application Information
AI Technical Summary
Problems solved by technology
Method used
Image
Examples
Embodiment Construction
[0021] The present invention will be further explained below in conjunction with the accompanying drawings and specific embodiments.
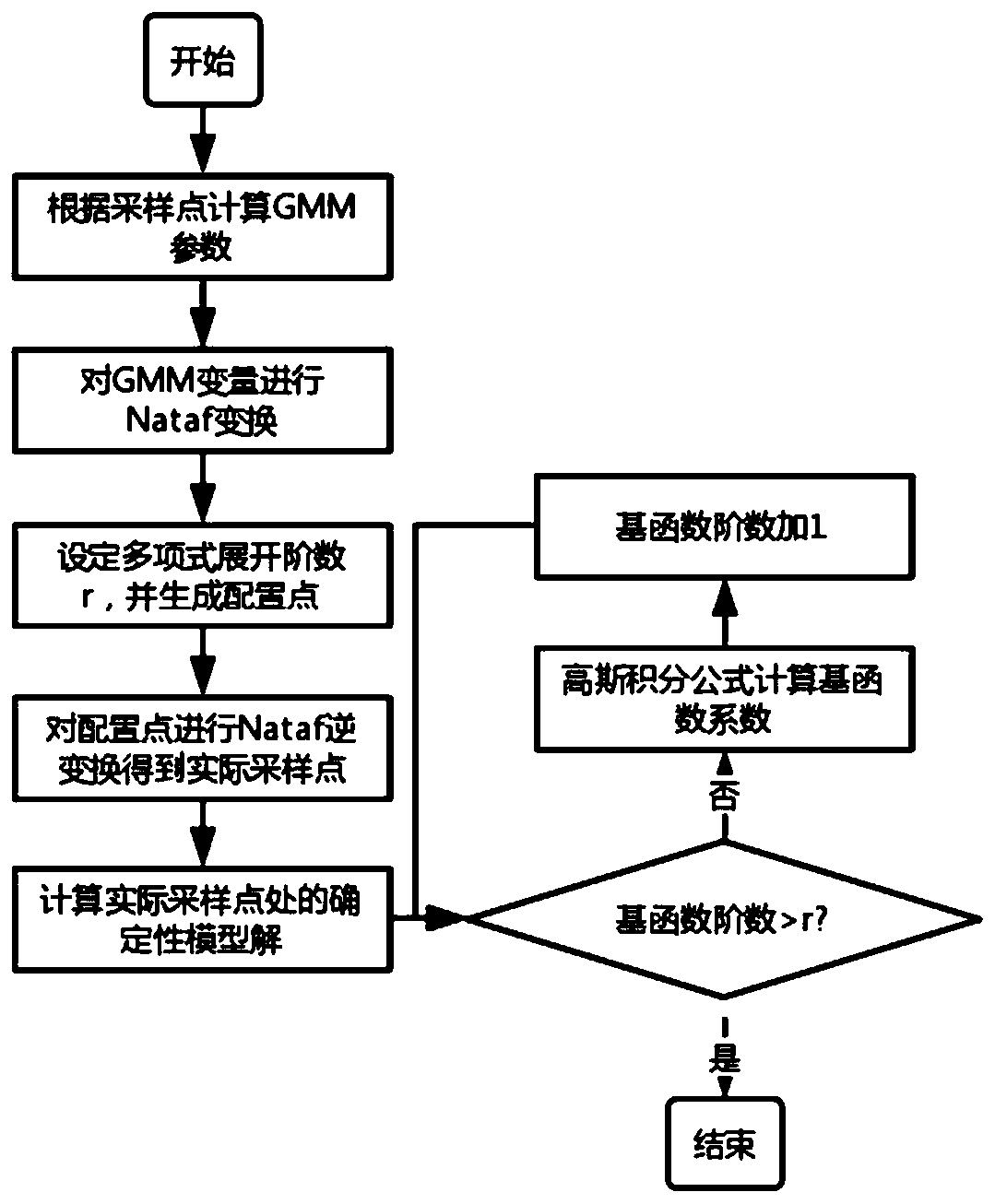
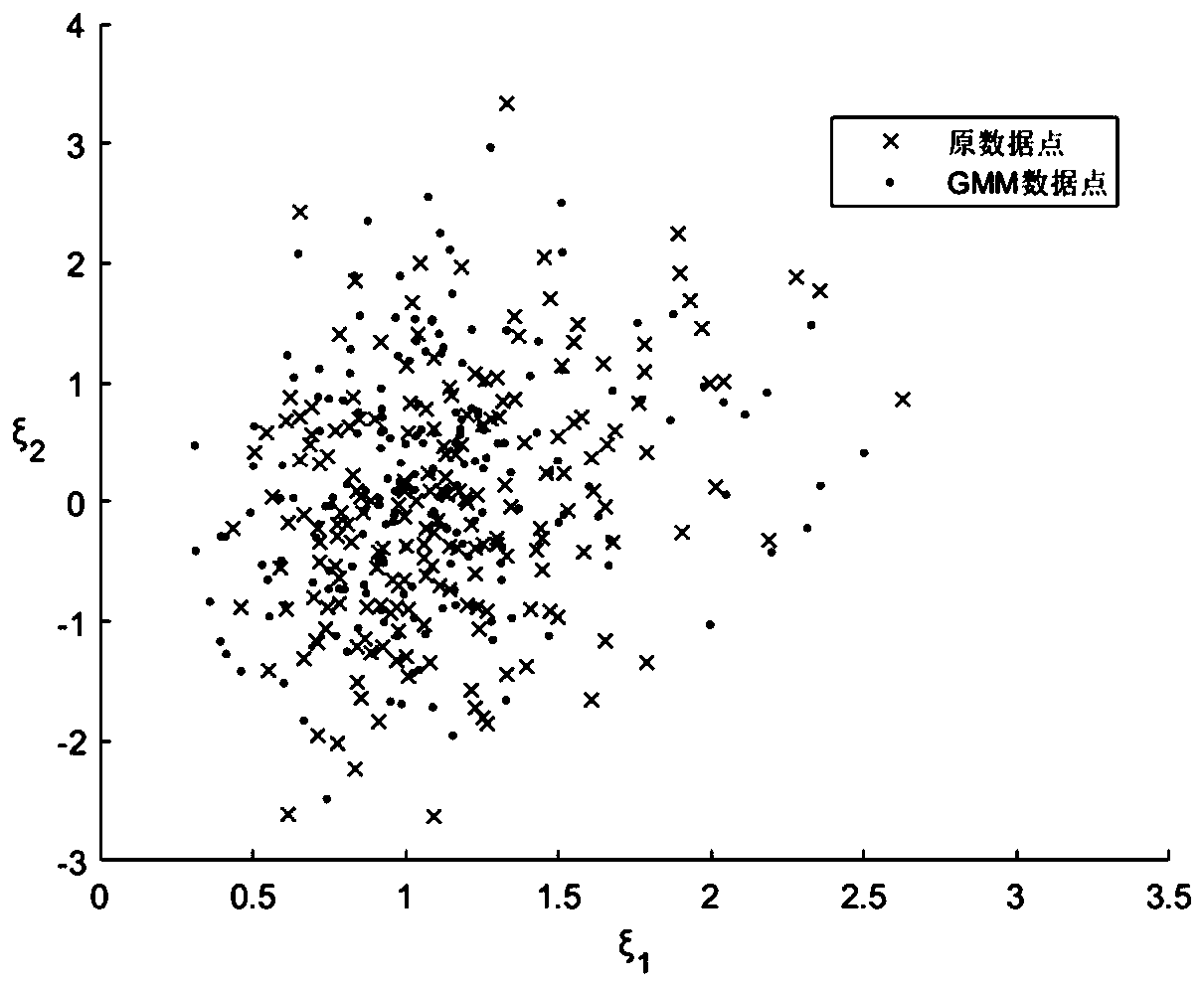
[0022] This embodiment uses the Gaussian mixture model to model the distribution of the associated process deviation, and then uses the improved Nataf transformation combined with the random collocation method to analyze the uncertainty of the MEMS device, as shown in figure 1 As shown, the specific steps are as follows:
[0023] 1) According to the multi-dimensional discrete data of the process deviation obtained through experiments or process simulation, a Gaussian mixture model (GMM) is established, which can decompose the density function of any distribution into the sum of a series of Gaussian distribution density functions:
[0024]
[0025] Among them, ρ norm (·|μ j , ∑ j ) is a Gaussian distribution density function with mean μ j , the covariance matrix is ∑ j ,p i is the weighting coefficient, satisfying Obviously, The m...
PUM
 Login to View More
Login to View More Abstract
Description
Claims
Application Information
 Login to View More
Login to View More - R&D
- Intellectual Property
- Life Sciences
- Materials
- Tech Scout
- Unparalleled Data Quality
- Higher Quality Content
- 60% Fewer Hallucinations
Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.
© 2025 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com