Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

Direction-of-arrival estimation method based on sparse representation

A direction of arrival, sparse representation technology, applied in the field of signal processing, can solve the problems of slow response speed of target reconnaissance and passive positioning, unsatisfactory angular resolution, large estimation error, etc., to avoid angle search or angle matching process. , Improve the probability and accuracy of discovery, and improve the effect of angle measurement accuracy

Inactive Publication Date: 2014-09-03
XIDIAN UNIV
View PDF3 Cites 20 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

However, this method has a large amount of calculation, and in the case of low signal-to-noise ratio, the angular resolution is not ideal
In practical applications, since both target reconnaissance and passive positioning need to be carried out on the basis of angle estimation, the use of the above methods will cause the problems of slow response speed and large estimation error of target reconnaissance and passive positioning

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
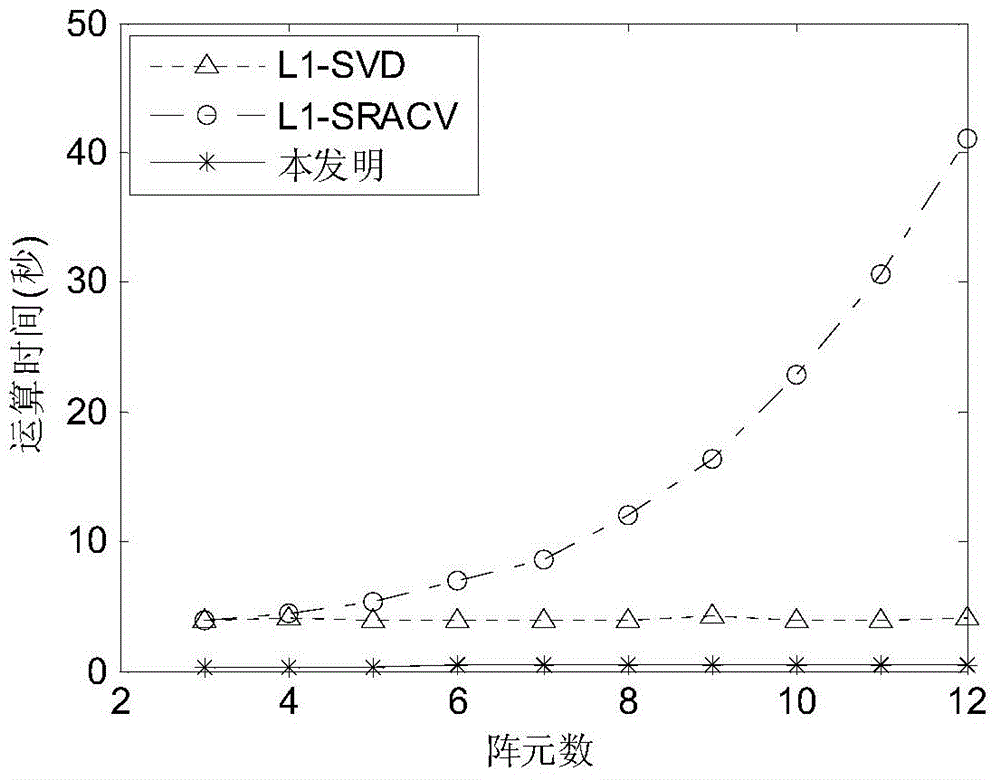
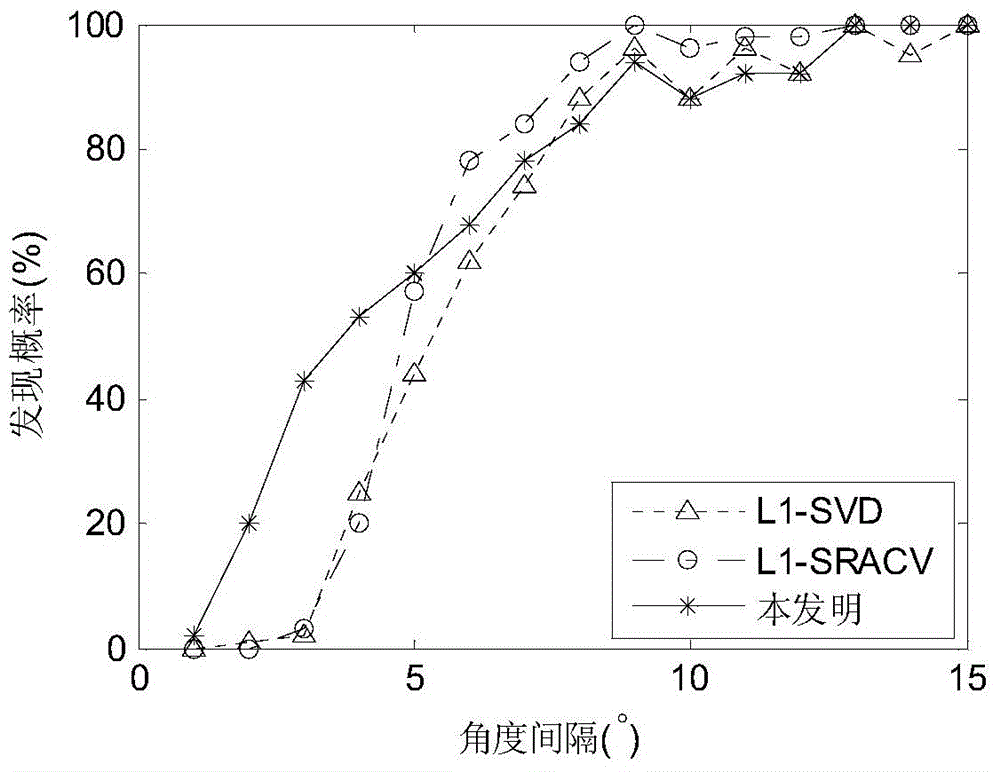
  • Direction-of-arrival estimation method based on sparse representation
  • Direction-of-arrival estimation method based on sparse representation
  • Direction-of-arrival estimation method based on sparse representation

Examples

Experimental program
Comparison scheme
Effect test

Embodiment Construction

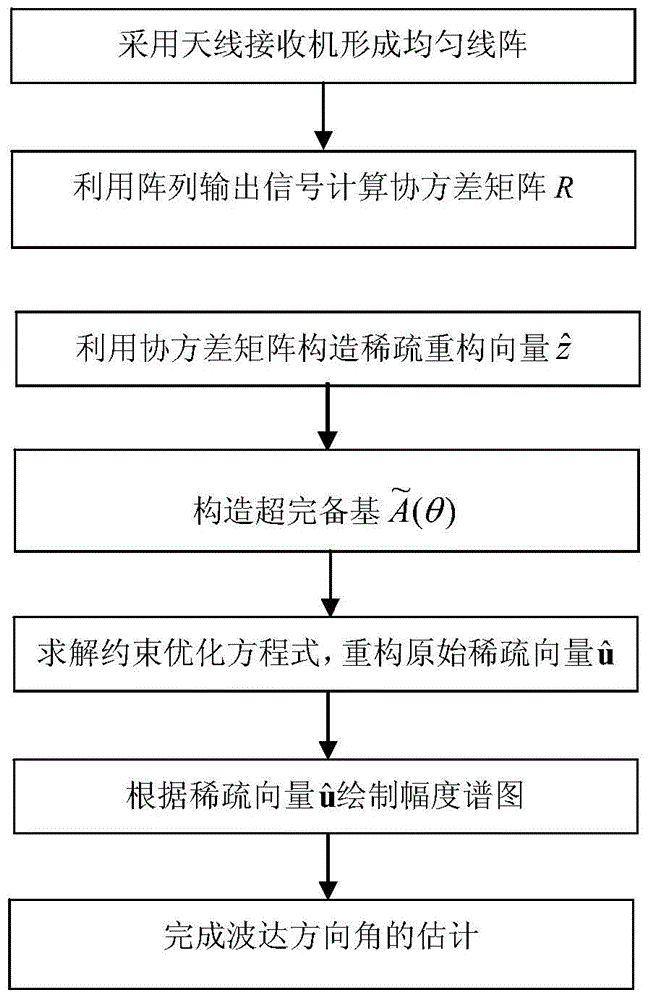
[0034] refer to figure 1 , the implementation steps of the present invention are as follows:

[0035] Step 1: Use the antenna receiver to form a uniform line array.

[0036] Antenna receivers are placed every interval d, and a total of M are placed to form a uniform linear antenna array. Assume that there are K signal sources incident on the antenna array, where M≥2, K≥1, 0<d≤λ / 2, λ is the wavelength of the incident narrowband signal.

[0037] Step 2: According to the output signal X(t) of the array antenna, calculate its covariance matrix R:

[0038] R=E{X(t)X H (t)},

[0039] Among them, E( ) means seeking mathematical expectation, ( ) H Represents the matrix conjugate transpose operation.

[0040] Step 3: Construct a sparse reconstruction vector using the covariance matrix R

[0041] The element R(p,q) of the pth row and qth column of the covariance matrix R is expressed as:

[0042] R ( p , q ...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention discloses a direction-of-arrival estimation method based on sparse representation. The method mainly solves the problems that because a similar technology is large in calculation amount and low in angular resolution, the estimation speed of target reconnaissance and passive location is low and estimation errors in target reconnaissance and passive location are large. The method comprises the implementation steps: (1) acquiring output signals of an array and calculating a covariance matrix R of the signals, (2) constructing reconstructed sparse vectors through lower triangular elements of the covariance matrix R, (3) carrying out mesh generation on a spatial domain to construct a perfect base, (4) converting the direction-of-arrival estimation issue into the issue of solving a constrained optimization equation according to the sparse representation relation between the reconstructed sparse vectors and the perfect base, (5) solving the constrained optimization equation according to the convex optimization method to obtain the optimal estimation vectors, (6) drawing a magnitude spectrum according to the one-to-one correspondence of the optimal estimation vectors and spatial domain angles to obtain the direction-of-arrival value. By means of the method, the calculation speed of target reconnaissance and passive location is decreased, and the estimation errors in target reconnaissance and passive location are reduced. The method can be applied to target reconnaissance and passive location.

Description

technical field [0001] The invention belongs to the technical field of signal processing, in particular to a direction-of-arrival angle estimation method, which can be used for target reconnaissance and passive positioning. Background technique [0002] Direction of Arrival Angle of Arrival (DOA) estimation is to use antenna arrays in different positions in space to receive signals from multiple signal sources in different directions, and use modern signal processing methods to quickly and accurately obtain the direction of signal sources. field has important application value. Among the models constructed for this problem, the subspace-based model appears earlier and is widely used. Most of the previous DOA estimation methods are generated using this model. In recent years, the theory of compressed sensing proposed by Donoho et al. provides a new way of thinking for DOA estimation, resulting in a class of DOA estimation methods based on sparse representation models. [00...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
Patent Type & Authority Applications(China)
IPC IPC(8): G01S3/00
CPCG01S3/14
Inventor 蔡晶晶李鹏鲍丹武斌秦国栋刘高高冯小平张银平
Owner XIDIAN UNIV
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com