Liquid refractive index sensing method and liquid refractive index sensing device
A liquid refractive index and refractive index technology, which is applied in the measurement of phase influence characteristics, etc., can solve the problems of small dynamic measurement range, variation, and different output characteristics of refractive index sensors, achieve high sensitivity, improve linearity, and expand linearity. The effect of the working area
- Summary
- Abstract
- Description
- Claims
- Application Information
AI Technical Summary
Problems solved by technology
Method used
Image
Examples
Embodiment 1
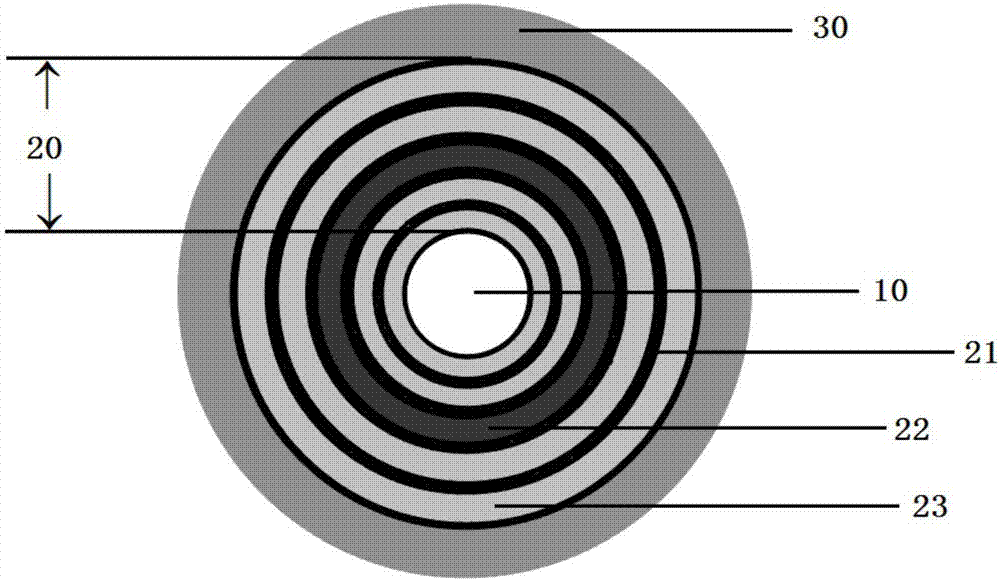
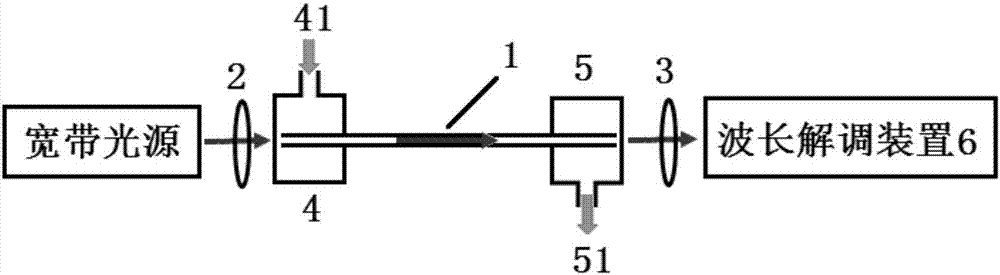
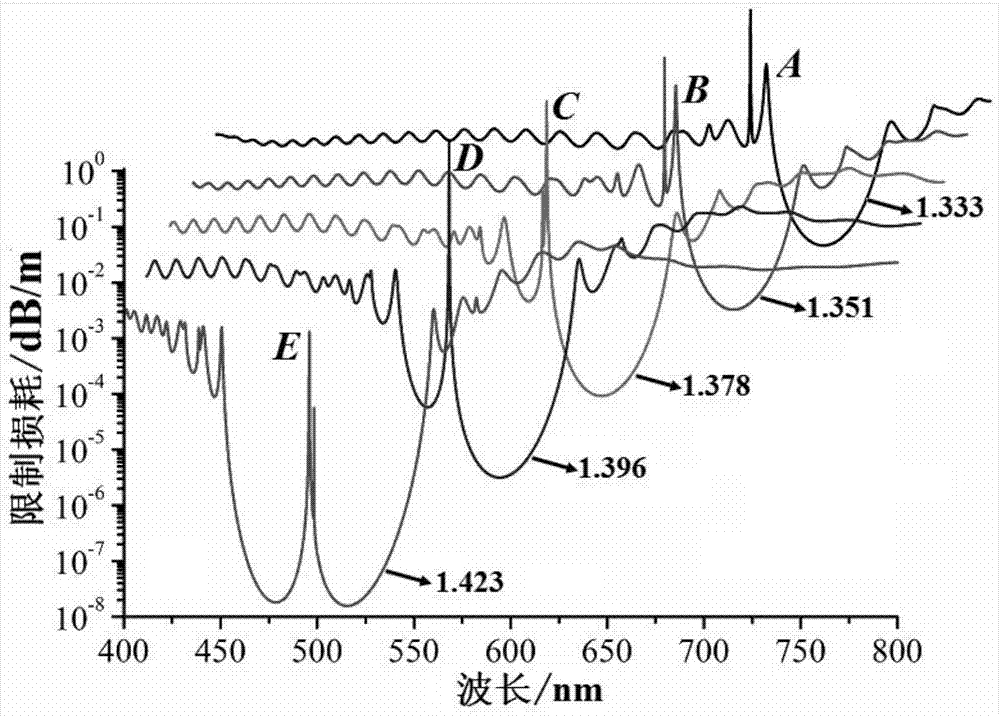
[0044] image 3 Shown is the structural parameter of the hollow Bragg optical fiber with a defect layer in the refractive index sensing device in Example 1 of the present invention is d c =800μm, N=25, n h =1.581,n l =1.487,n d =1.581,t h =130nm, t l =370nm,t d =1.2t l ,N d = 3, the refractive index of the core filling liquid n a HE at different values 11 Mode confinement loss versus wavelength. Due to the mutual coupling between the defect mode and the core mode, the HE 11 Two loss peaks, one wide and one narrow, appear in the loss spectrum of the mode, and their central wavelengths are respectively denoted by λ RW and lambda RN Indicates that below we will use λ RW (Such as image 3 A, B, C, D and E points in the center) is the characteristic wavelength λ S , to study and analyze its refractive index sensing performance. In Figure 3, A, B, C, D and E correspond to n a The characteristic wavelength λ at 1.333, 1.351, 1.378, 1.396 and 1.423 S . Depend on im...
Embodiment 2
[0045] Embodiment 2: Since the resonance wavelength in the hollow Bragg fiber containing the defect layer depends on the structural parameters of the defect layer, such as the refractive index, thickness and position of the defect layer in the cladding. Therefore, by optimizing the structural parameters of the defect layer, the λ in the liquid-filled hollow Bragg fiber sensor with a defect layer can be further improved S with n a linear correlation between them. Figure 5 The structure parameter of the hollow Bragg fiber with defect layer shown is d c =800μm, N=25, n h =1.581, n l =1.487,n d =1.68,t h =130nm,t l =370nm,t d = t l ,N d= 2, the liquid fills the λ in the hollow Bragg fiber sensor with defect layer S with n a In the figure, the abscissa x represents the liquid refractive index, and the ordinate y represents the characteristic wavelength. to lambda S with n a Linear fitting found that the linear correlation between the two was further improved (R 2 =0...
PUM
| Property | Measurement | Unit |
|---|---|---|
| diameter | aaaaa | aaaaa |
| refractive index | aaaaa | aaaaa |
Abstract
Description
Claims
Application Information
 Login to View More
Login to View More - R&D
- Intellectual Property
- Life Sciences
- Materials
- Tech Scout
- Unparalleled Data Quality
- Higher Quality Content
- 60% Fewer Hallucinations
Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.
© 2025 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com