Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

Variational integral-discretization Lagrange model-based Buck-Boost converter modeling and nonlinear analysis method

A nonlinear analysis and converter technology, applied in instruments, special data processing applications, electrical digital data processing, etc., can solve the problems of inaccurate discrete iterative models, inaccurate nonlinear dynamic behaviors, and complex discrete iterative model operations. , to achieve the effect of reducing computational complexity and computing time

Inactive Publication Date: 2015-09-16
HARBIN INST OF TECH
View PDF3 Cites 18 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

[0003] The purpose of the present invention is to solve the problem that the inaccurate establishment of the approximate discrete iterative model leads to inaccurate nonlinear dynamic behavior and the large amount of calculation of the stroboscopic mapping model makes the calculation of the discrete iterative model complicated. Modeling and Nonlinear Analysis Method of Buck-Boost Converter Based on Integral Discrete Lagrangian Model

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Variational integral-discretization Lagrange model-based Buck-Boost converter modeling and nonlinear analysis method
  • Variational integral-discretization Lagrange model-based Buck-Boost converter modeling and nonlinear analysis method
  • Variational integral-discretization Lagrange model-based Buck-Boost converter modeling and nonlinear analysis method

Examples

Experimental program
Comparison scheme
Effect test

specific Embodiment approach 1

[0027] Specific embodiment one: a kind of Buck-Boost converter modeling and nonlinear analysis method based on the variational integral discrete Lagrangian model of the present embodiment is specifically prepared according to the following steps:

[0028] Step 1, constructing the Euler-Lagrange function of the Buck-Boost converter;

[0029] Step 2, after discretizing the Buck-Boost Euler-Lagrangian function, constructing the Hamiltonian system equation;

[0030] Step 3. Through the Hamiltonian system equation, derive the position-momentum p of the variational integral k and p k+1 ;

[0031] Step 4, according to p k and p k+1 Establish a discrete Lagrangian model of the Buck-Boost converter;

[0032] Step 5, using the discrete Lagrangian model of the Buck-Boost converter to derive the Jacobian matrix eigenvalues ​​of the system;

[0033] Step 6. Use the eigenvalues ​​of the Jacobian matrix to find the nonlinear behavior of the Buck-Boost converter's stable region and the ...

specific Embodiment approach 2

[0038] Specific embodiment two: the difference between this embodiment and specific embodiment one is: the Euler-Lagrangian function of constructing the Buck-Boost converter in step one is specifically:

[0039] (1) Definition is the kinetic energy of the circuit, ν u (q C) is the potential energy of the circuit, is the dissipation function of the circuit, ν u,nc (q) is the external force function of the system, u is the control signal; when u=0, the switch is off, and when u=1, the switch is on, the specific form is as follows:

[0040] T u ( q · L ) = 1 2 L q · L 2 , v u ( q C ) ...

specific Embodiment approach 3

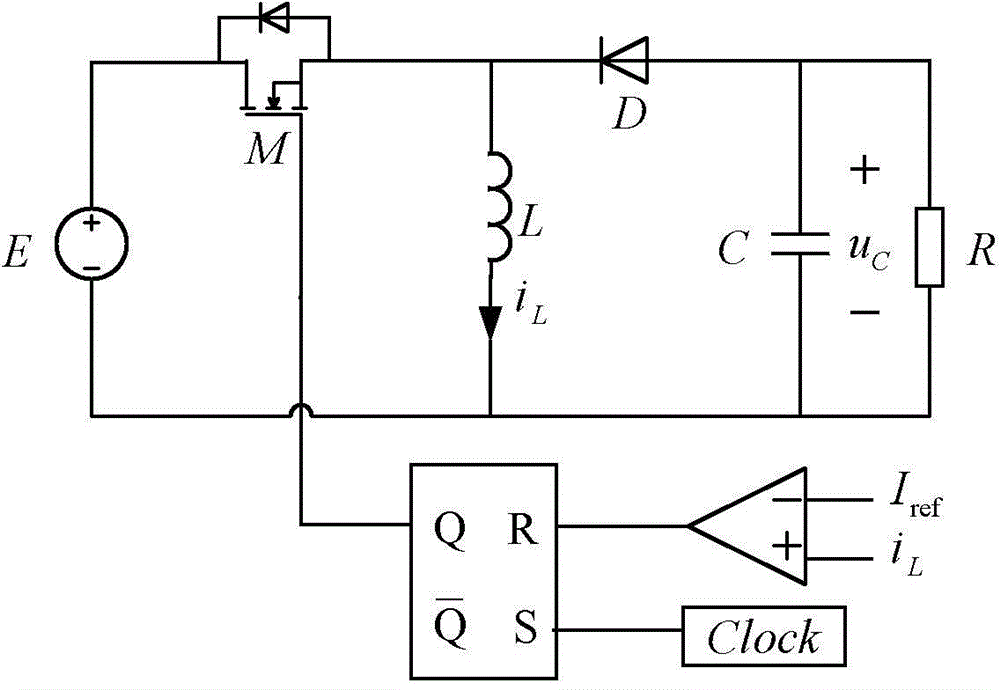
[0047] Embodiment 3: The difference between this embodiment and Embodiment 1 or 2 is that the schematic diagram of the Buck-Boost converter current mode control in Step 1 is as follows: figure 1 As shown, the current mode control of the Buck-Boost converter is specifically:

[0048] (1) All the components in the circuit are regarded as ideal devices; among them, the components in the circuit include inductors, capacitors, resistors, switching tubes, input power supplies, comparators and RS flip-flops; ideal devices have a single constant relationship Components affected by other factors such as material temperature;

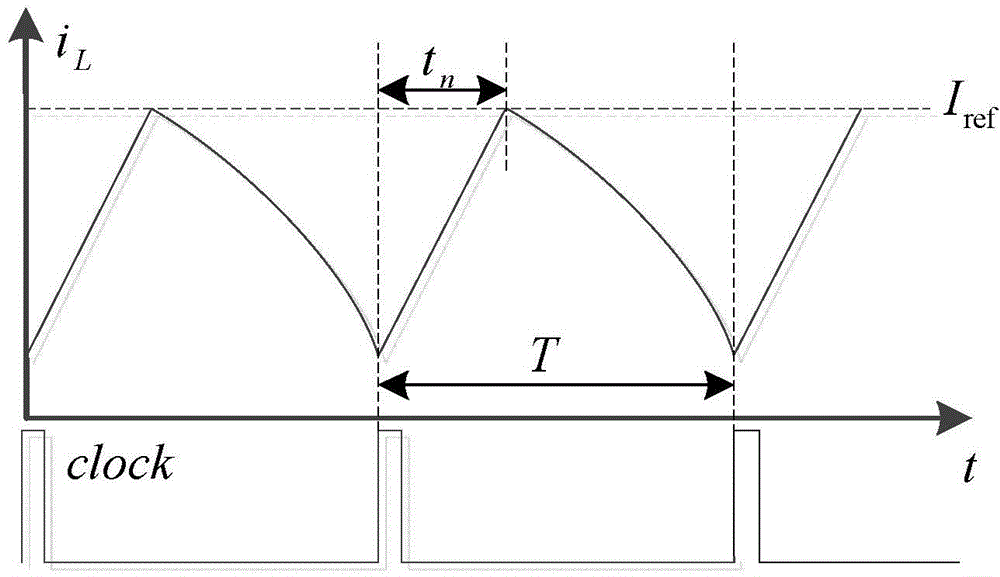
[0049] (2) Divert the inductor current i L with reference current I ref For comparison, if i L greater than I ref , then the input of the R terminal of the RS flip-flop is 1; if i L less than I ref , then the input of the R terminal of the RS flip-flop is 0;

[0050] (3) The clock signal is input through the S terminal of the RS flip-flop. If the input of ...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

The invention provides a variational integral-discretization Lagrange model-based Buck-Boost converter modeling and nonlinear analysis method. The invention relates to a Buck-Boost converter modeling and nonlinear analysis method. The method of the invention is used for solving the problem that operation of a discrete iterative model is complex because the calculated quantity of an established stroboscopic mapping model is large. The method of the invention is realized by the steps as follows: 1) establishing an Euler-Lagrange function; 2) establishing a Hamiltonian system equation; 3) exporting location momentum of a variational integral; 4) establishing a discrete Lagrange model; 5) exporting a Jacobian matrix characteristic value; 6) calculating non-linear behaviors of a stability region of the Buck-Boost converter and a first branch point of the Buck-Boost converter. The method of the invention is applied to the field of the Buck-Boost converter modeling and nonlinear analysis.

Description

technical field [0001] The invention relates to Buck-Boost converter modeling and nonlinear analysis, in particular to a Buck-Boost converter modeling and nonlinear analysis method based on the variational integral discrete Lagrangian model. Background technique [0002] Power electronic converters are strongly nonlinear systems, which contain rich nonlinear dynamic behaviors. In the past two decades, people have extensively and deeply studied the complex nonlinear behavior of DC-DC converters, and have formed a complete set of methods for analyzing the nonlinear behavior of the system. When analyzing the slow-scale and fast-scale dynamic behaviors of DC-DC converters, the mathematical model of the system must be deduced first. In modeling, the state-space averaging method, equivalent circuit method, and energy conservation method are widely used. Due to the complex topology and rich nonlinear dynamic behavior of DC-DC converters, the discrete-time mapping modeling method c...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
IPC IPC(8): G06F17/50
Inventor 刘洪臣周祺堃
Owner HARBIN INST OF TECH
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com