Looking for breakthrough ideas for innovation challenges? Try Patsnap Eureka!

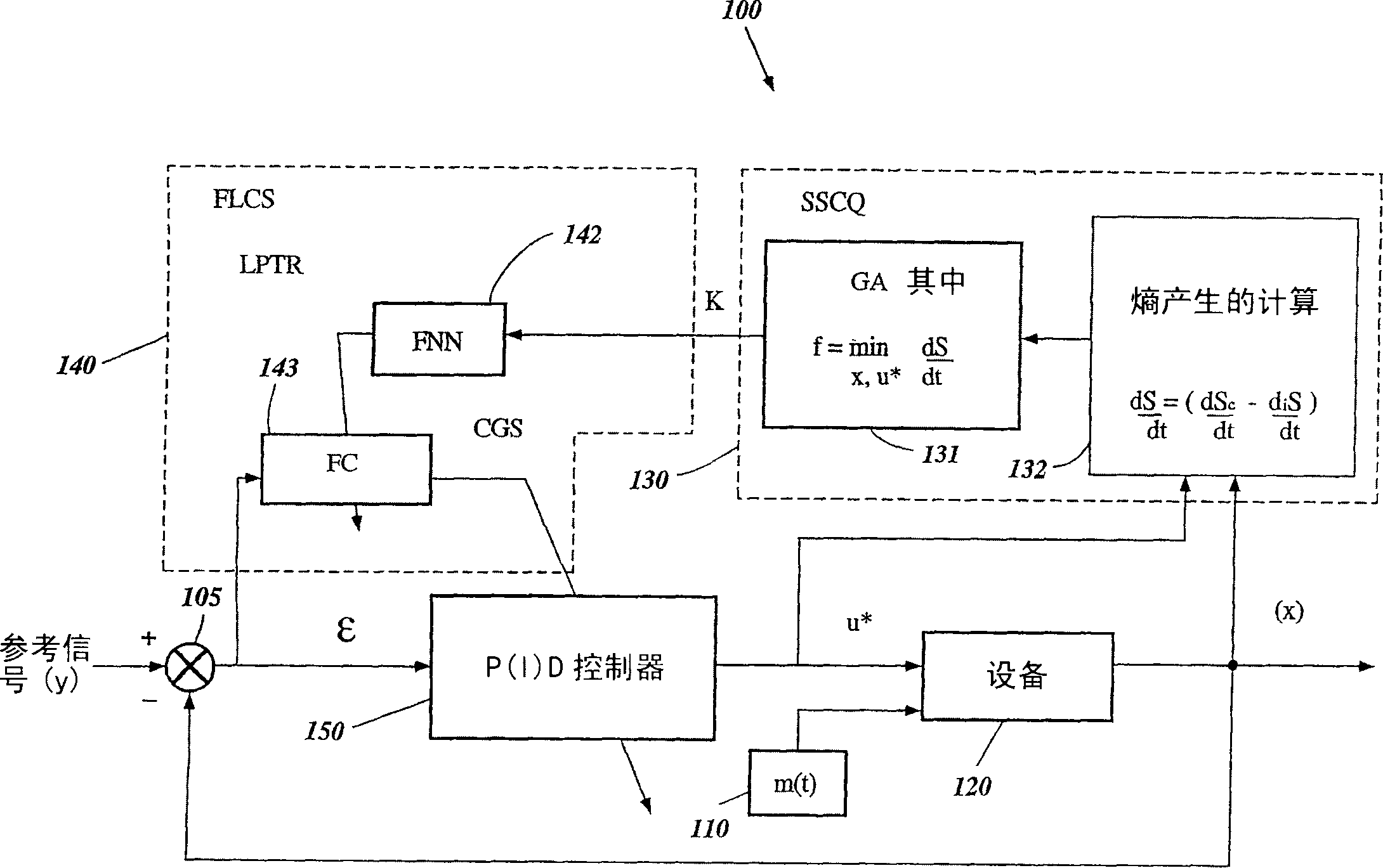
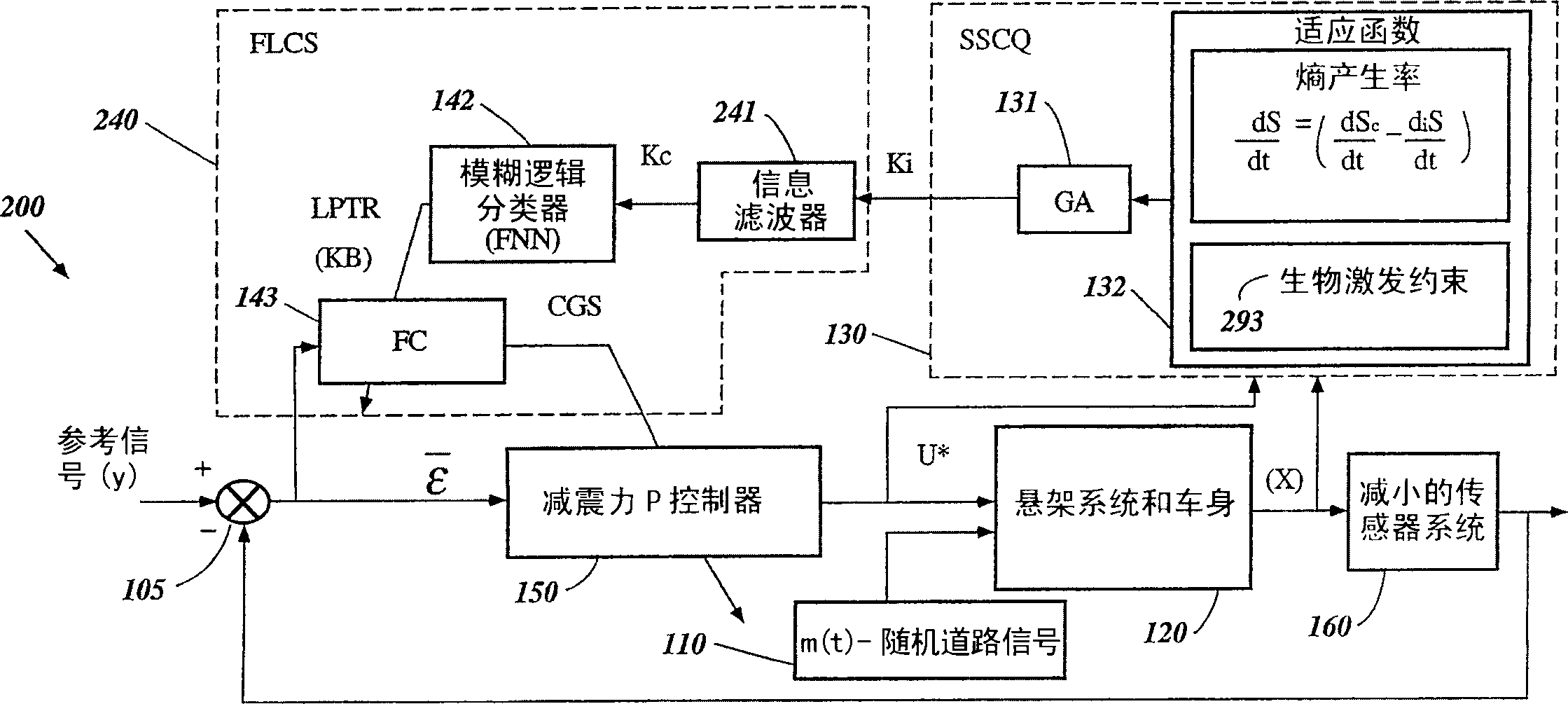
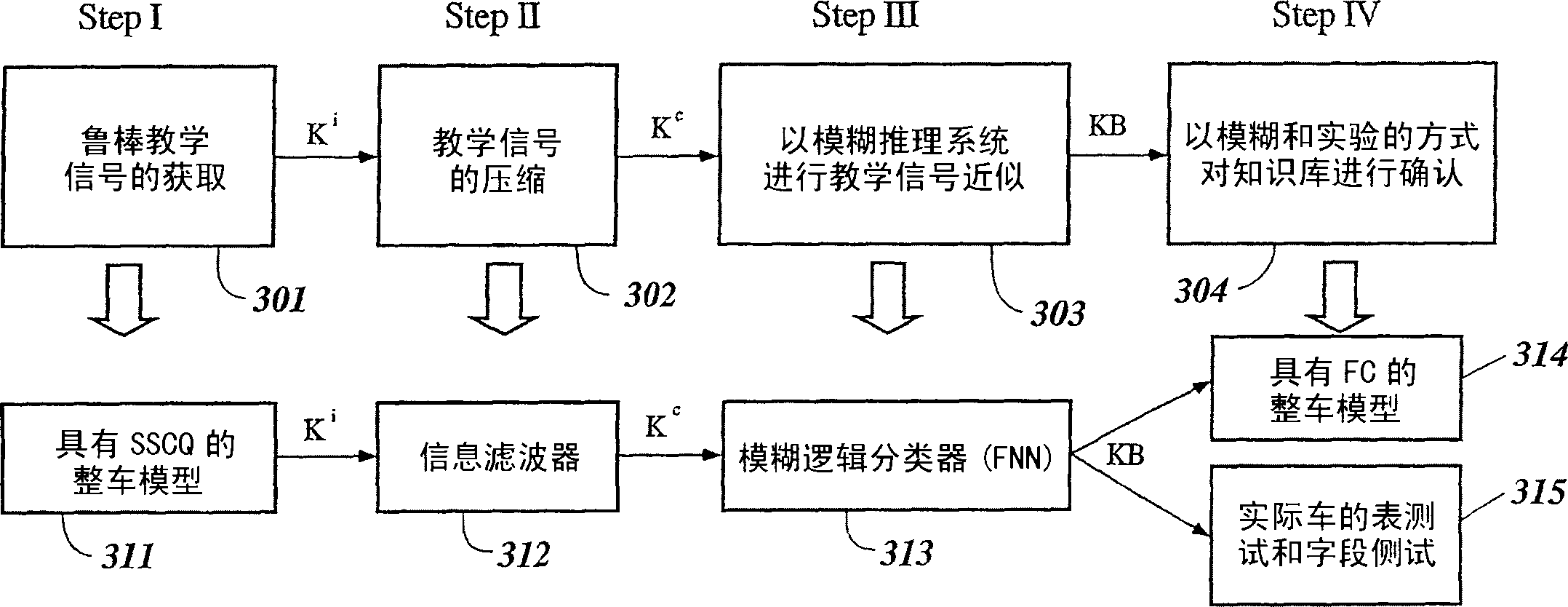
Intelligent mechatronic control suspension system based on quantum soft computing

A control system and suspension system technology, applied in the field of control systems, can solve problems such as poor control quality and difficulty in generating training signal approximations

Inactive Publication Date: 2005-09-21
YAMAHA MOTOR CO LTD
View PDF1 Cites 32 Cited by
  • Summary
  • Abstract
  • Description
  • Claims
  • Application Information

AI Technical Summary

Problems solved by technology

[0007] When a genetic analyzer is used to generate a training signal in a fuzzy neural network, the training signal typically includes unnecessary random noise, making it difficult to generate an approximation of the training signal later, and when it will be used in an operating condition (such as a different road conditions) training signals may lead to poor control quality when used in different environments (e.g. in different types of road conditions)

Method used

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
View more

Image

Smart Image Click on the blue labels to locate them in the text.
Viewing Examples
Smart Image
  • Intelligent mechatronic control suspension system based on quantum soft computing
  • Intelligent mechatronic control suspension system based on quantum soft computing
  • Intelligent mechatronic control suspension system based on quantum soft computing

Examples

Experimental program
Comparison scheme
Effect test

example 1

[0165] Example 1: Assume that X(t) is uniformly distributed, ie

[0166] p ( x ) = 1 2 Δ , - Δ ≤ x ≤ Δ - - - ( 2.10 )

[0167] Substitute (2.10) into (2.8)

[0168] D. 2 (x)=α(Δ 2 -x 2 ) (2.11)

[0169] In this case, given the desired Ito equation

[0170] dX = αXdt + α ( Δ 2 - X 2 ) dB ( t ) - - - ( 2.12 )

[0171]Interestingly, ...

example 2

[0174] Example 2: Make X(t) Rayleigh distributed

[0175] p(x)=γ 2 xexp(-γx), γ>0, 0≤x<∞ (2.14)

[0176] Its concentrated form Y(t)=X(t)-2 / γ has a probability density:

[0177] p(y)=γ(γy+2)exp(-γy+2), -2 / γ≤y<∞ (2.15)

[0178] From equation (2.8)

[0179] D 2 ( y ) = 2 α γ ( y + 2 γ ) - - - ( 2.16 )

[0180] The lto equation for Y(t) is

[0181] dY = - αYdt + [ 2 α γ ( Y + 2 γ ...

example 3

[0185] Example 3: Consider a family of probability densities that obey the following equation:

[0186] d dx p ( x ) = J ( x ) p ( x ) - - - ( 2.19 )

[0187] After integrating equation (2.19), we get

[0188] p(x)=C 1 exp(∫J(x)dx) (2.20)

[0189] where C 1 is the standardized constant, in this case

[0190] D. 2 (x)=-2αexp[-J(x)]∫xexp[J(x)]dx (2.21)

[0191] A number of special circumstances should be noted, so that

[0192] J(x)=-γx 2 -δx 4 , -∞

[0193] where γ is arbitrary if δ>0, and substituting equation (2.22) into equation (2.8) gives

[0194] D 2 ( x ) = ...

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

PUM

No PUM Login to View More

Abstract

A control system for optimizing a shock absorber having a non-linear kinetic characteristic is described. The control system uses a fitness (performance) function that is based on the physical laws of minimum entropy and biologically inspired constraints relating to mechanical constraints and / or rider comfort, driveability, etc. In one embodiment, a genetic analyzer is used in an off-line mode to develop a teaching signal. The teaching signal can be approximated online by a fuzzy controller that operates using knowledge from a knowledge base. A learning system is used to create the knowledge base for use by the online fuzzy controller. In one embodiment, the learning system uses a quantum search algorithm to search a number of solution spaces to obtain information for the knowledge base. The online fuzzy controller is used to program a linear controller.

Description

technical field [0001] The present invention relates generally to control systems, and more particularly to electronically controlled suspension systems. Background technique [0002] Feedback control systems are widely used to keep the output value of a dynamic system at a desired value despite external disturbances that make it deviate from the desired value. For example, a domestic space heating fireplace controlled by a thermostat is an example of a feedback control system. The thermostat continuously measures the temperature of the air in the room, and when the temperature drops below the expected minimum temperature, the thermostat turns on the fireplace, and when the room temperature reaches the expected minimum temperature, the thermostat turns off the fireplace. While thermostat-fireplace systems maintain the indoor temperature at a relatively constant value despite external disturbances, such as a drop in outdoor temperature, the same type of feedback control is u...

Claims

the structure of the environmentally friendly knitted fabric provided by the present invention; figure 2 Flow chart of the yarn wrapping machine for environmentally friendly knitted fabrics and storage devices; image 3 Is the parameter map of the yarn covering machine
Login to View More

Application Information

Patent Timeline
no application Login to View More
IPC IPC(8): B60G17/015G05B13/02G06N3/00G06N99/00
CPCB82Y10/00G06N99/002G06N10/00
Inventor 谢尔盖·V·乌里扬诺夫谢尔盖·潘菲洛夫萩原孝英高桥一树卢德米拉·利特温谢娃
Owner YAMAHA MOTOR CO LTD
Who we serve
  • R&D Engineer
  • R&D Manager
  • IP Professional
Why Patsnap Eureka
  • Industry Leading Data Capabilities
  • Powerful AI technology
  • Patent DNA Extraction
Social media
Patsnap Eureka Blog
Learn More
PatSnap group products

Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.

© 2024 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com