Method for efficiently solving steady-state microdynamics equation set based on automatic combination of time integration and Newton method
A dynamic equation and time integration technology, applied in complex mathematical operations and other directions, can solve problems such as failure of solution results, long solution time, long solution time, etc., to improve the breadth and diversity, ensure the success rate of solutions, and simplify the algorithm process. Effect
- Summary
- Abstract
- Description
- Claims
- Application Information
AI Technical Summary
Problems solved by technology
Method used
Image
Examples
Embodiment 1
[0064] Pt(111) Surface Catalyzed CO Oxidation Reaction to CO 2 Process microkinetic analysis.
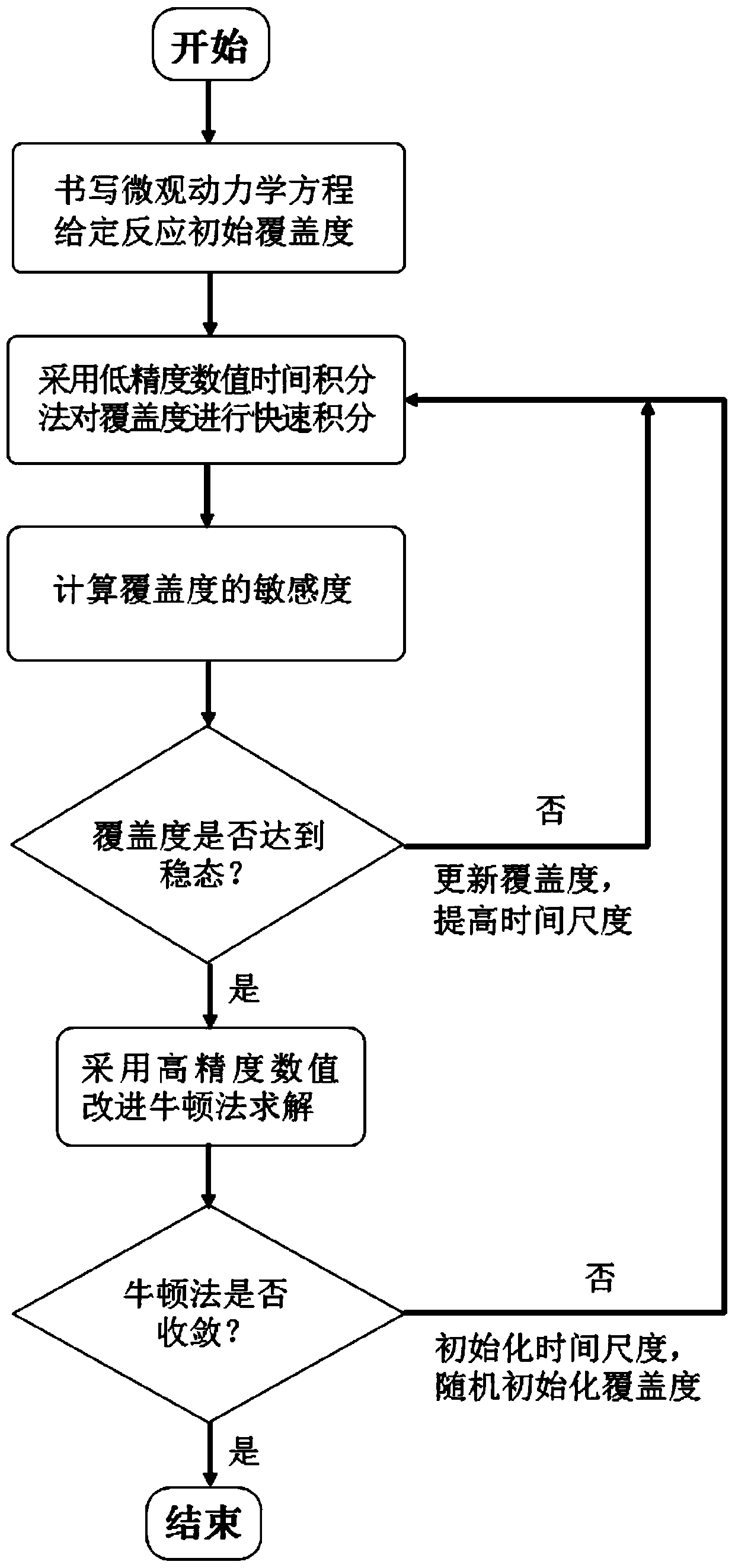
[0065] Such as figure 2 as shown, figure 2 It is a schematic flowchart of the method for automatically and efficiently solving steady-state microscopic dynamics equations based on time integration and Newton's method of the present invention.
[0066] Reaction conditions: CO, O 2 and CO 2 The partial pressures are 0.01, 0.2 and 0.002atm respectively, and the reaction temperature is 300K. The reaction mechanism, reaction free energy barrier and reaction free energy are shown in Table 1:
[0067] Table 1
[0068]
[0069] The energy data were all calculated by the quantum chemical software VASP.
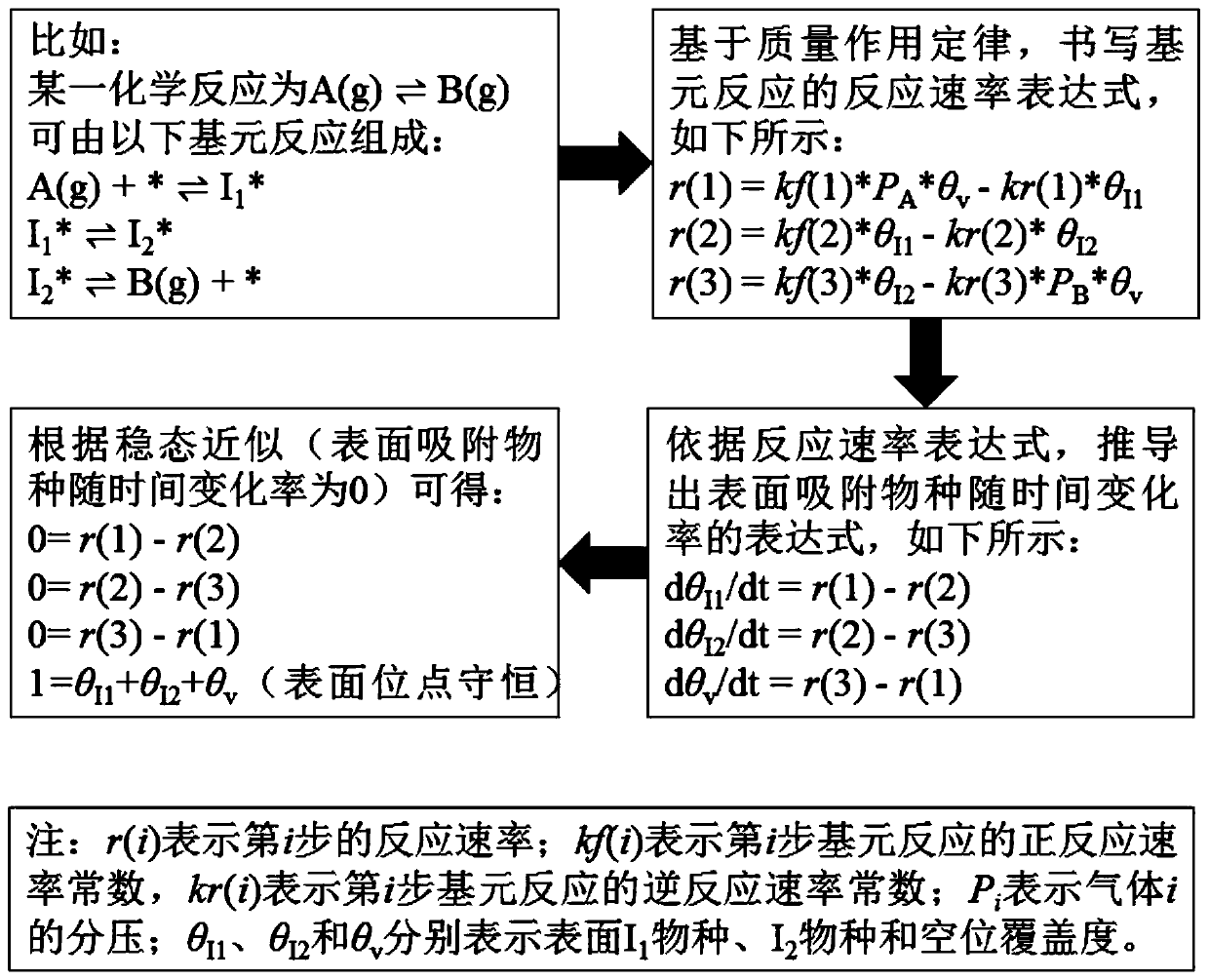
[0070] First, according to the law of mass action, the reaction rate expression for each elementary reaction can be written as:
[0071] r(1)=kf(1)*P CO *θ v -kr(1)*θ CO
[0072] r(2)=kf(2)*P O2 *θ v 2 -kr(2)*θ O 2
[0073] r(3)=kf(3)*θ CO *θ O -kr(3)*P CO 2 *θ v ...
Embodiment 2
[0088] Catalytic N on Fe(211) surface 2 and H 2 The reaction produces NH 3 (Ammonia Synthesis Reaction) Microkinetic Analysis.
[0089] Reaction conditions: reaction temperature is 723K, reaction pressure: H 2 , N 2 , NH 3 75, 25, and 11.6 atm respectively; the reaction mechanism, reaction free energy barrier and reaction free energy are shown in Table 2:
[0090] Table 2
[0091]
[0092] The energy data were all calculated by the quantum chemical software VASP.
[0093] First, according to the law of mass action, the reaction rate expression for each elementary reaction can be written as:
[0094] r(1)=kf(1)*P N2 *θ v 2 -kr(1)*θ N 2
[0095] r(2)=kf(2)*P H2 *θ v 2-kr(2)*θ H 2
[0096] r(3)=kf(3)*θ H *θ N -kr(3)*θ NH *θ v
[0097] r(4)=kf(4)*θ H *θ NH -kr(4)*θ NH2 *θ v
[0098] r(5)=kf(5)*θ H *θ NH2 -kr(5)*θ NH3 *θ v
[0099] r(6)=kf(6)*θ NH3 -kr(6)*P NH3 *θ v
[0100] Among them, r(i) represents the reaction rate of the i-th step; kf(...
Embodiment 3
[0119] Microkinetic analysis of the catalyzed iodine reduction reaction on the rutile 110 surface.
[0120] Reaction condition: reaction temperature is 298.15K, reaction concentration: I 2 , I - and e are 0.03, 0.6 and 1mol / L, respectively; reaction mechanism, reaction free energy barrier and reaction free energy and I adsorption energy E ads I The linear relationship is shown in Table 3:
[0121] table 3
[0122]
[0123] The energy data were all calculated by the quantum chemical software VASP.
[0124] First, according to the law of mass action, the reaction rate expression for each elementary reaction can be written as:
[0125] r(1)=kf(1)*C I2 *θ v 2 -kr(1)*θ I 2
[0126] r(2)=kf(2)*C e *θ I -kr(2)*C I - *θ v
[0127] Among them, r(i) represents the reaction rate of the i-th step; kf(i) represents the forward reaction rate constant of the i-th step elementary reaction, and kr(i) represents the reverse reaction rate constant of the i-th step elementary ...
PUM
 Login to View More
Login to View More Abstract
Description
Claims
Application Information
 Login to View More
Login to View More - R&D
- Intellectual Property
- Life Sciences
- Materials
- Tech Scout
- Unparalleled Data Quality
- Higher Quality Content
- 60% Fewer Hallucinations
Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.
© 2025 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com