Patents
Literature
Hiro is an intelligent assistant for R&D personnel, combined with Patent DNA, to facilitate innovative research.
265results about How to "Reliable engagement" patented technology
Efficacy Topic
Property
Owner
Technical Advancement
Application Domain
Technology Topic
Technology Field Word
Patent Country/Region
Patent Type
Patent Status
Application Year
Inventor
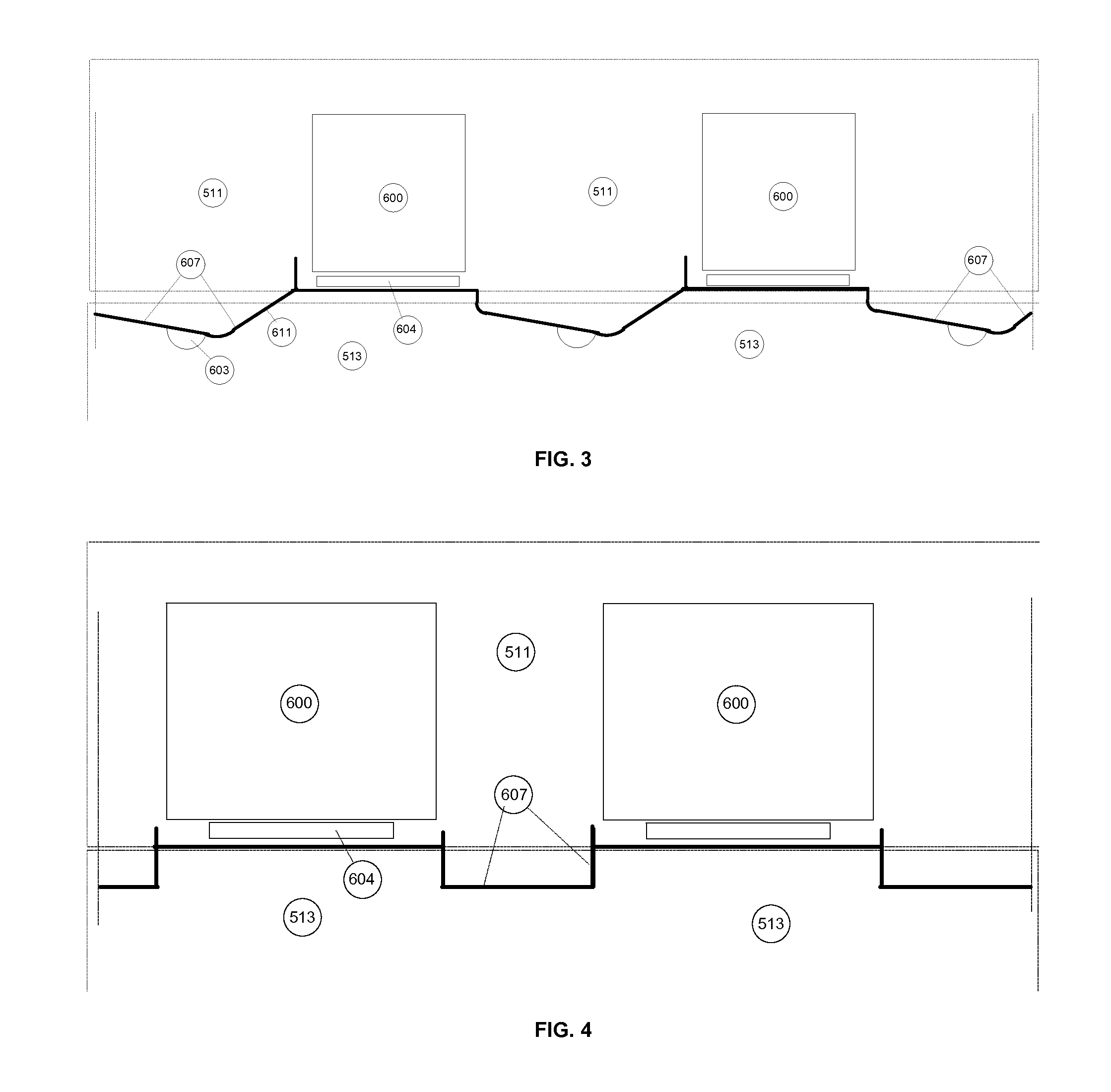
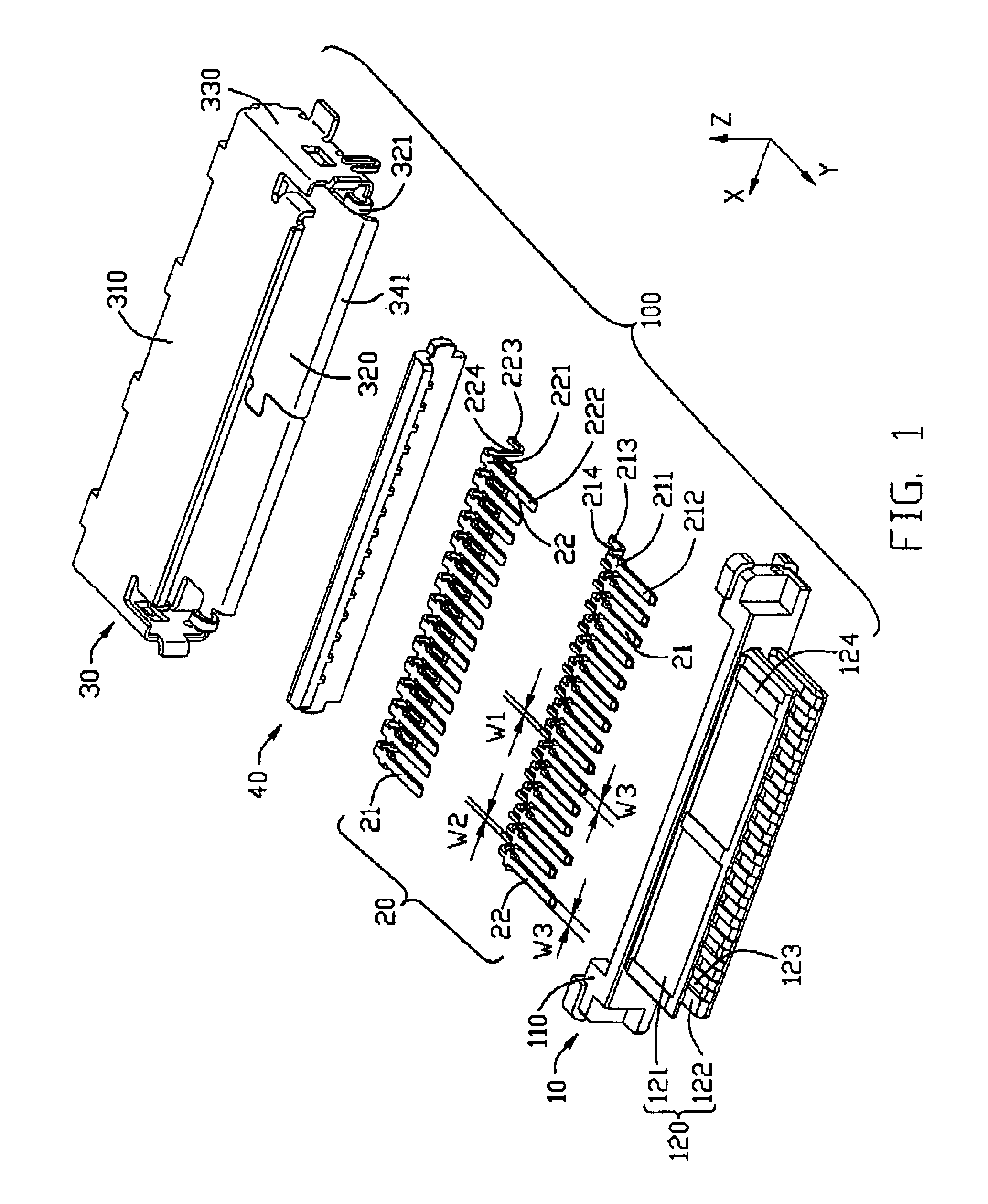
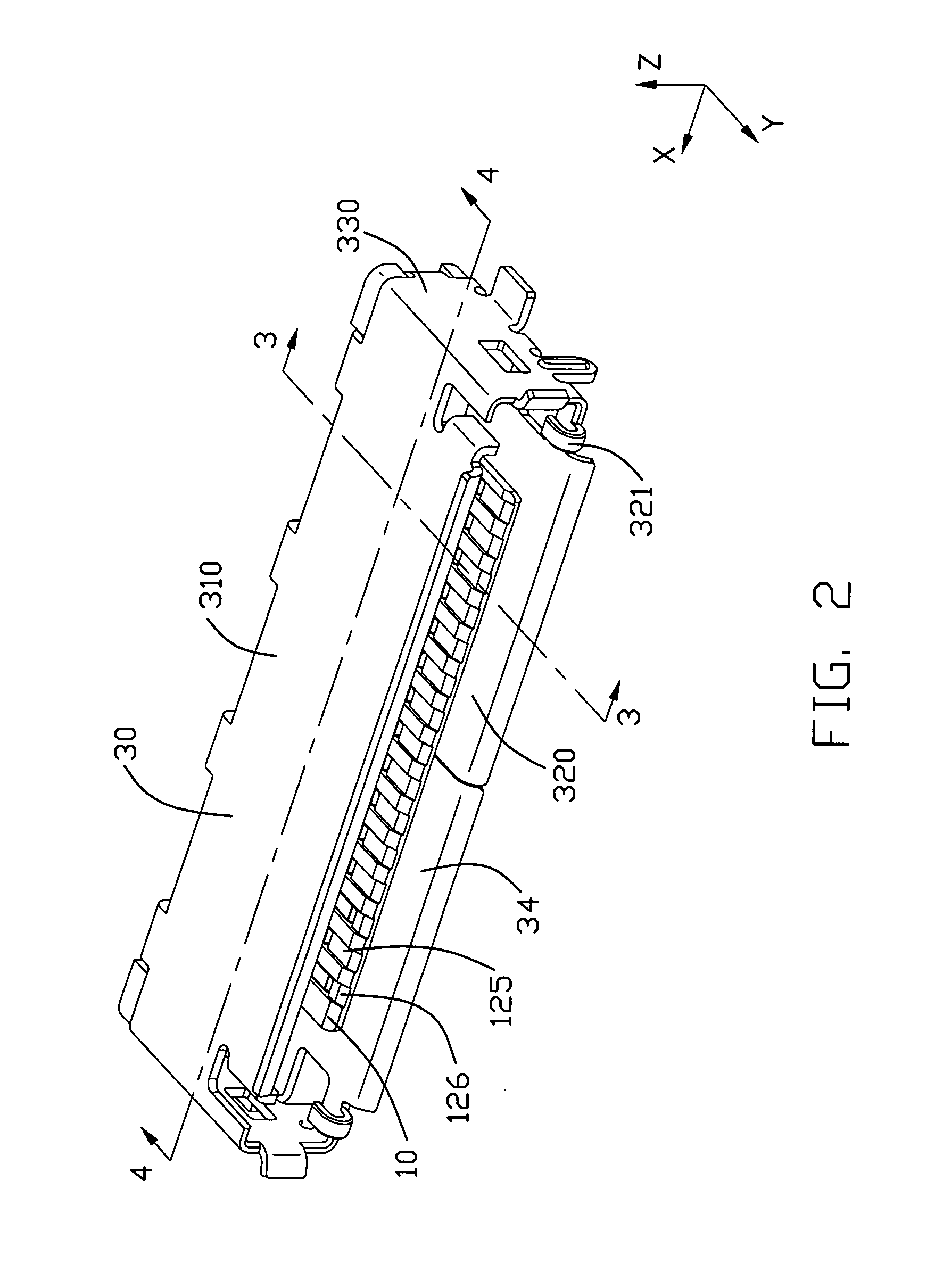
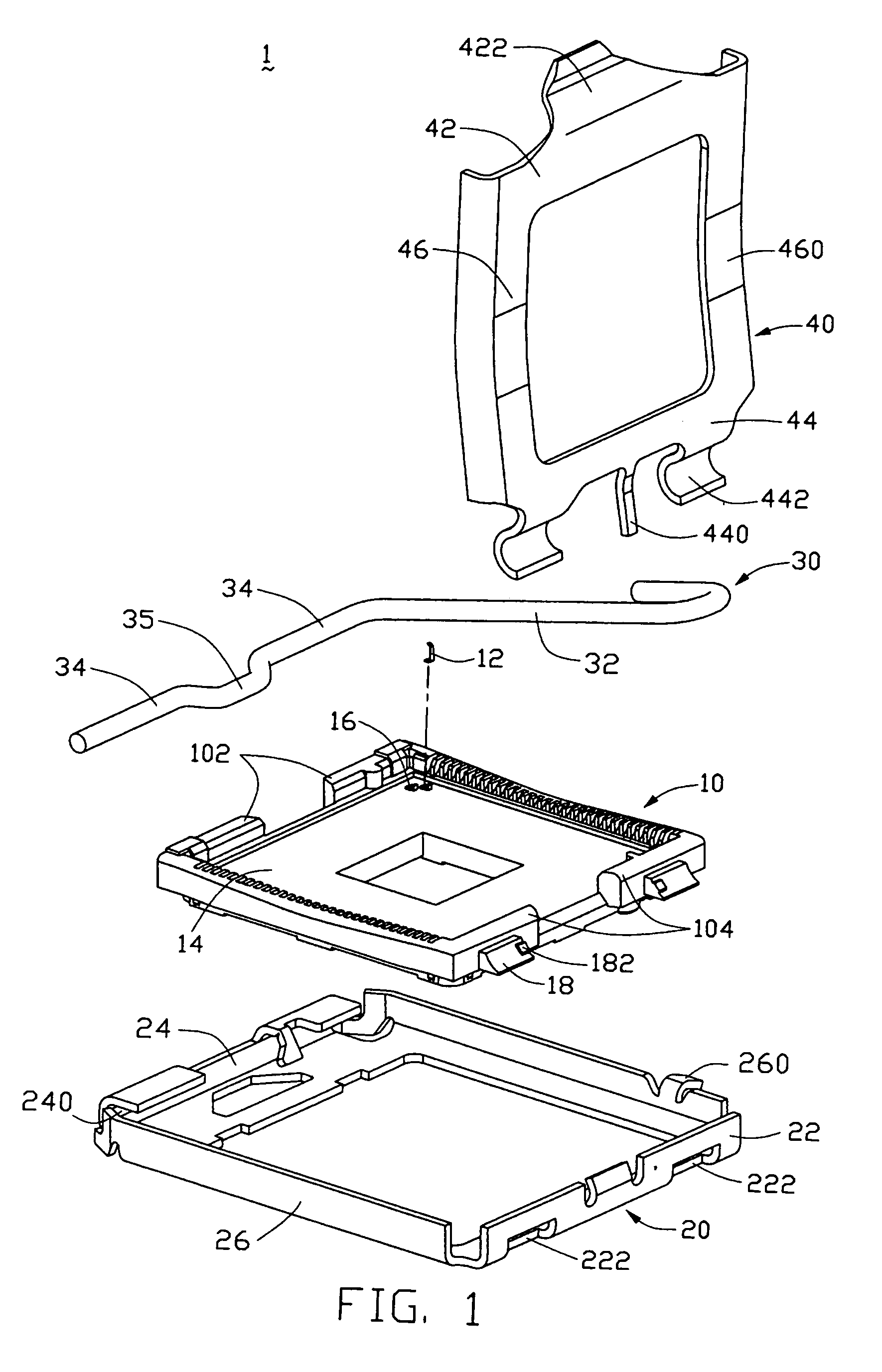
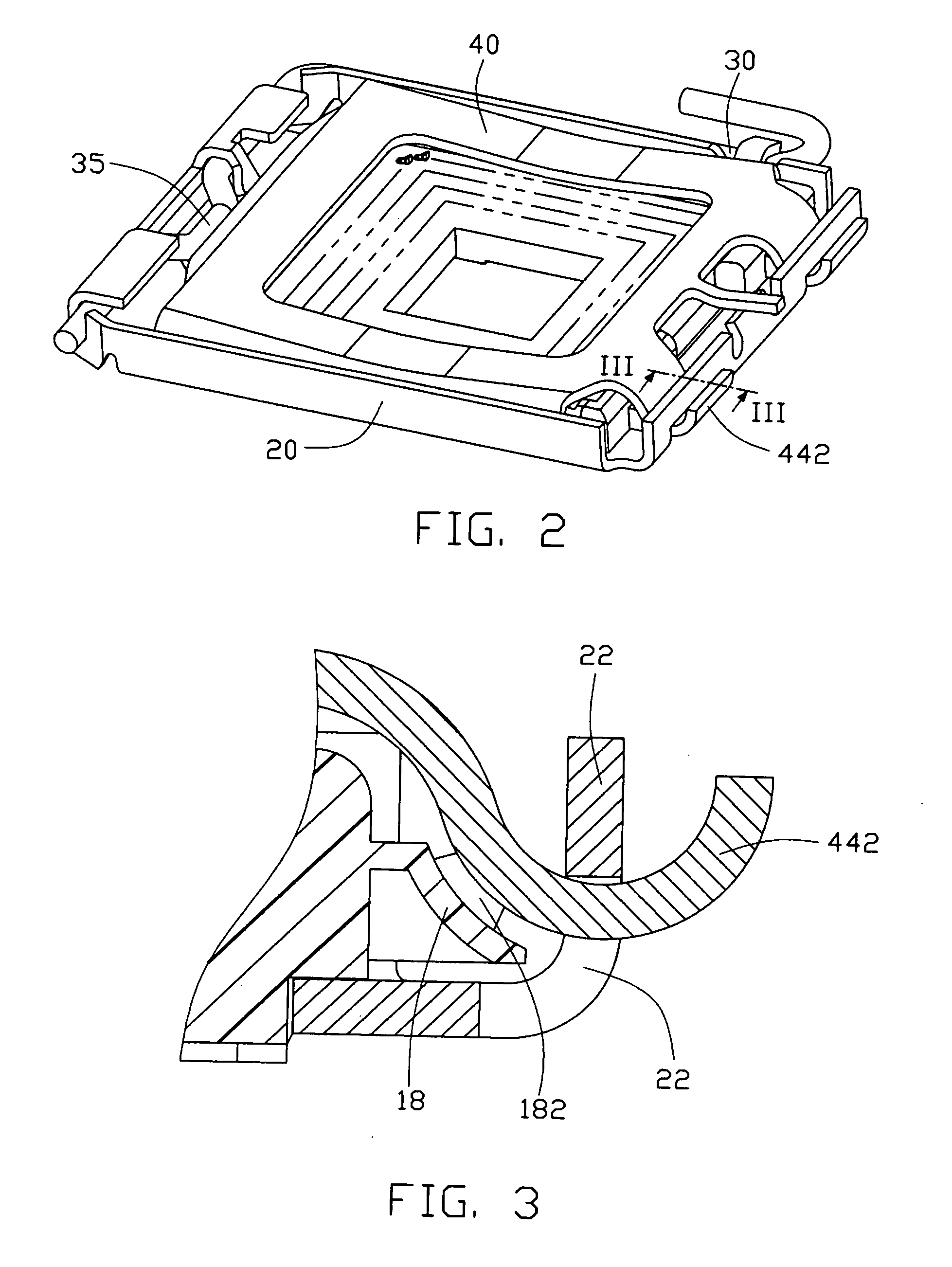
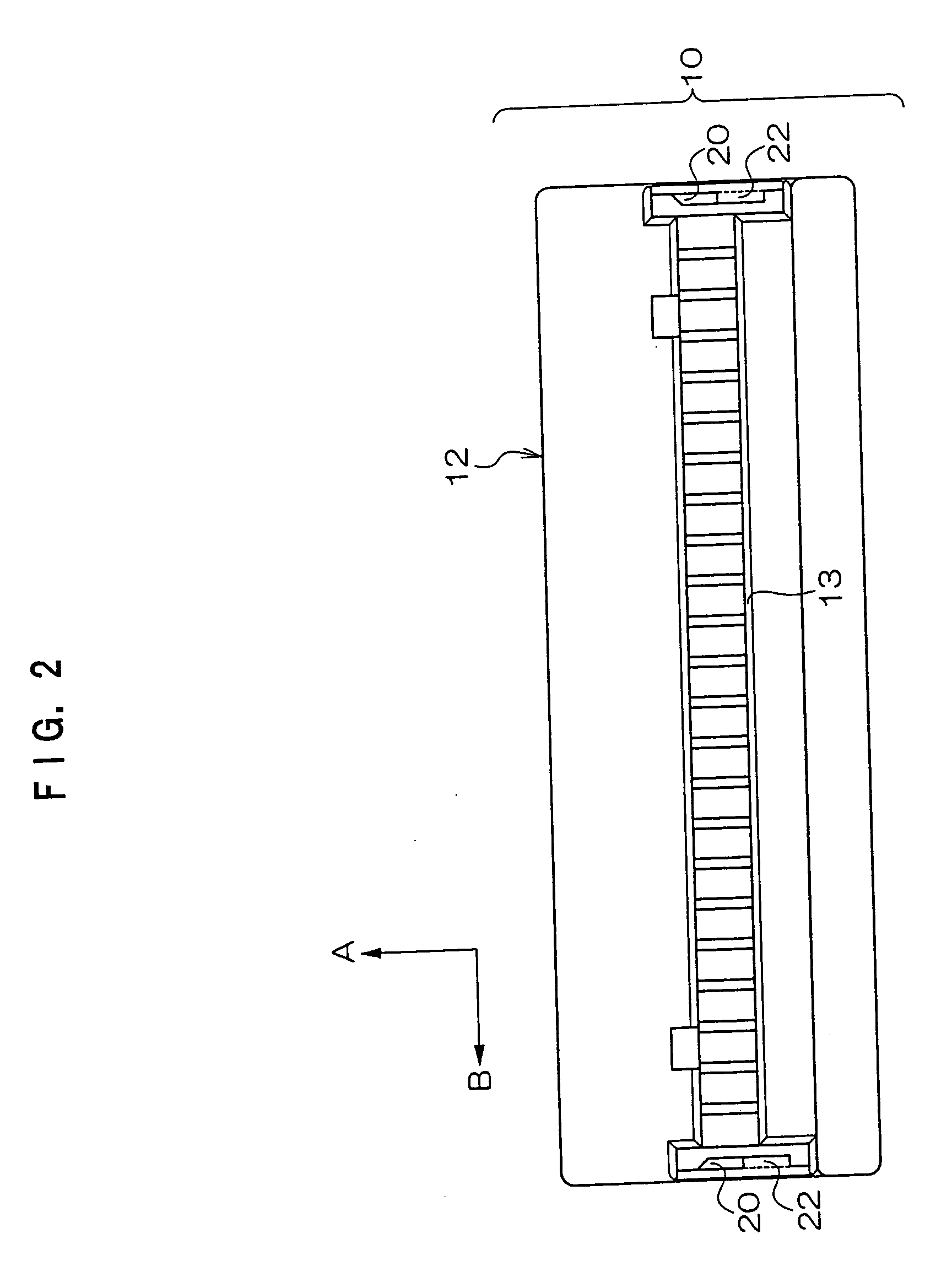
LGA contact with extended arm for IC connector
ActiveUS6955572B1Long elastic armReliable engagementSoldered/welded conductive connectionsCoupling contact membersAcute angleEngineering
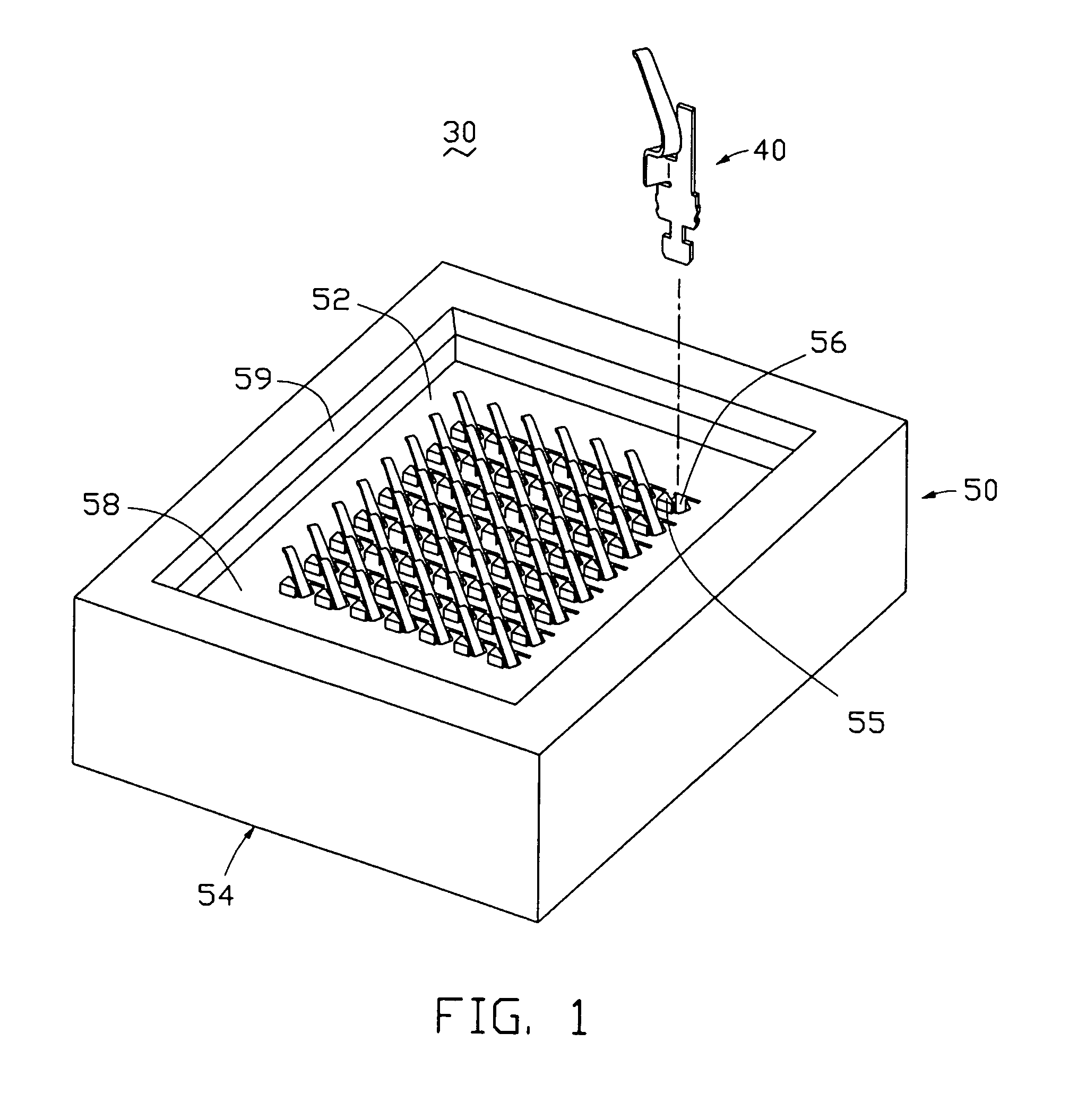
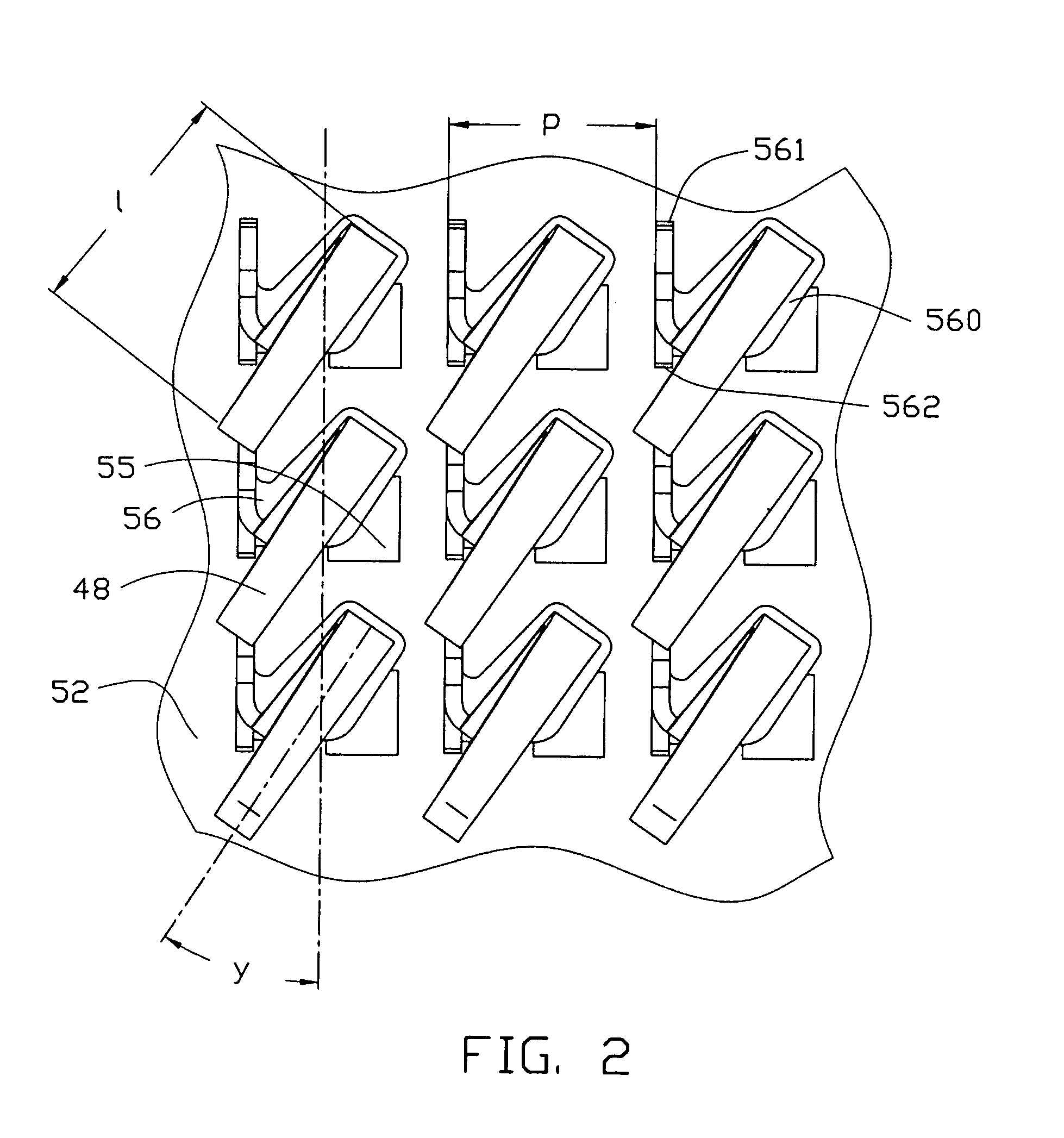
An LGA contact (40, 40′) for electrically connecting an IC package (32, 32′) and a PCB (34, 34′) includes a vertical basic part (42 and 44, 42′ and 44′), a vertical section (43, 43′) bendedly outwardly extending from a lateral side of the vertical basic part, an arm (48, 48′) extending upwardly from a top of said vertical section and a contacting portion (49, 49′) extending from an distal end of the arm. The arm has a rearwardly extending portion (482, 482′) and a forwardly extending portion (480, 480′). Further, two projections of the vertical section and the vertical basic part on a horizontal surface form an acute angle therebetween, thereby the arm can be devised to be relatively long to get good spring characteristics and many of this kind of contacts can be arranged in a relative less pitch as being assembled a housing (50, 50′).
Owner:HON HAI PRECISION IND CO LTD
Apparatus improving item transactions with real-time controls and navigation
InactiveUS20120080517A1Reliable matingReliable engagementComplete banking machinesFinanceReal-time Control SystemPayment
Invention describes real-time control system increasing shopping efficiency, maintaining item quality, inventory, and directing customer transactions, requiring handling and processing of items only once. Invention controls access to items inside modules via card with unique embedded identification features. Invention stores customer transactions and entered shopping lists. Invention per customer selected shopping list and items availability, will in real-time navigate customer to complete required transactions within customer selected criteria. Invention controls card identification features and modules containing items. Invention based on card identification and item identification such as barcode information will store customer executed transactions, and will require customer to pay for completed transactions before leaving the store. Invention in real-time maintains inventory of items, controls environment surrounding items, and reports items exceeding shelf life limit preventing their transaction. Invention analyzes transactions executed by the card, correlates transactions to customer at payment module, stores behavior patterns for future optimizations of transactions.
Owner:BRAUNSTEIN ZACHARY L
Lid opening/closing system for closed container
ActiveUS20100133270A1Avoid deformationAdequate strength in fixationCapsClosure capsEngineeringPosition sensor
The FIMS system is for use with a pod composed of a lid having an engaged portion provided on the outer surface thereof and a pod body having a latch mechanism including an engagement portion that engages the engaged portion as it moves along a predetermined axis to fix the lid to the pod. The FIMS system has a latch mechanism drive means for driving the latch mechanism along a certain axis and an engagement portion position sensor that can generate a signal indicating whether the engagement portion is at an engagement position or non-engagement position when the latch mechanism drive means drives the latch mechanism. The latch mechanism drive means and the engagement portion position sensor are provided in the vicinity of a first opening portion of the FIMS system.
Owner:TDK CORPARATION
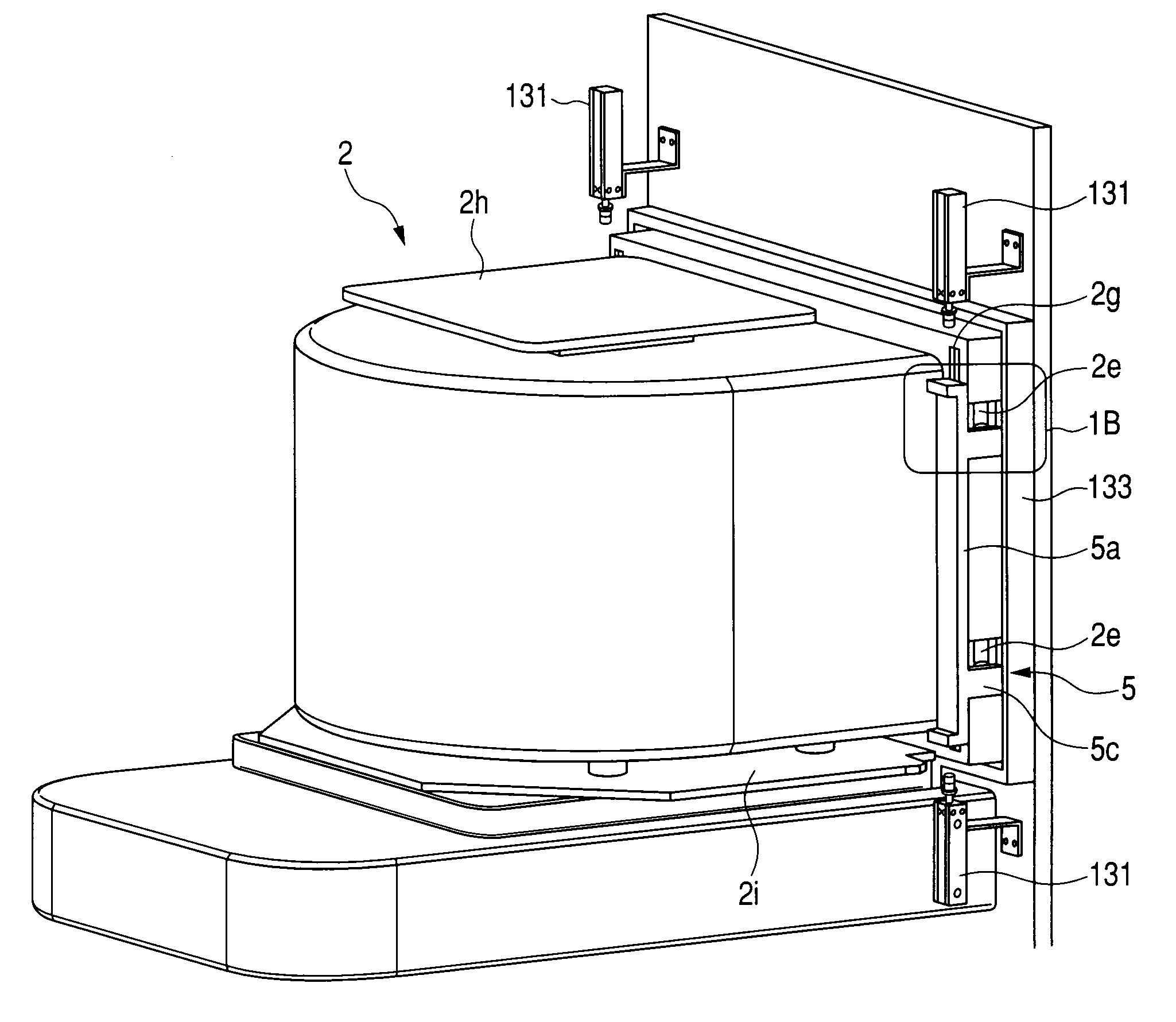
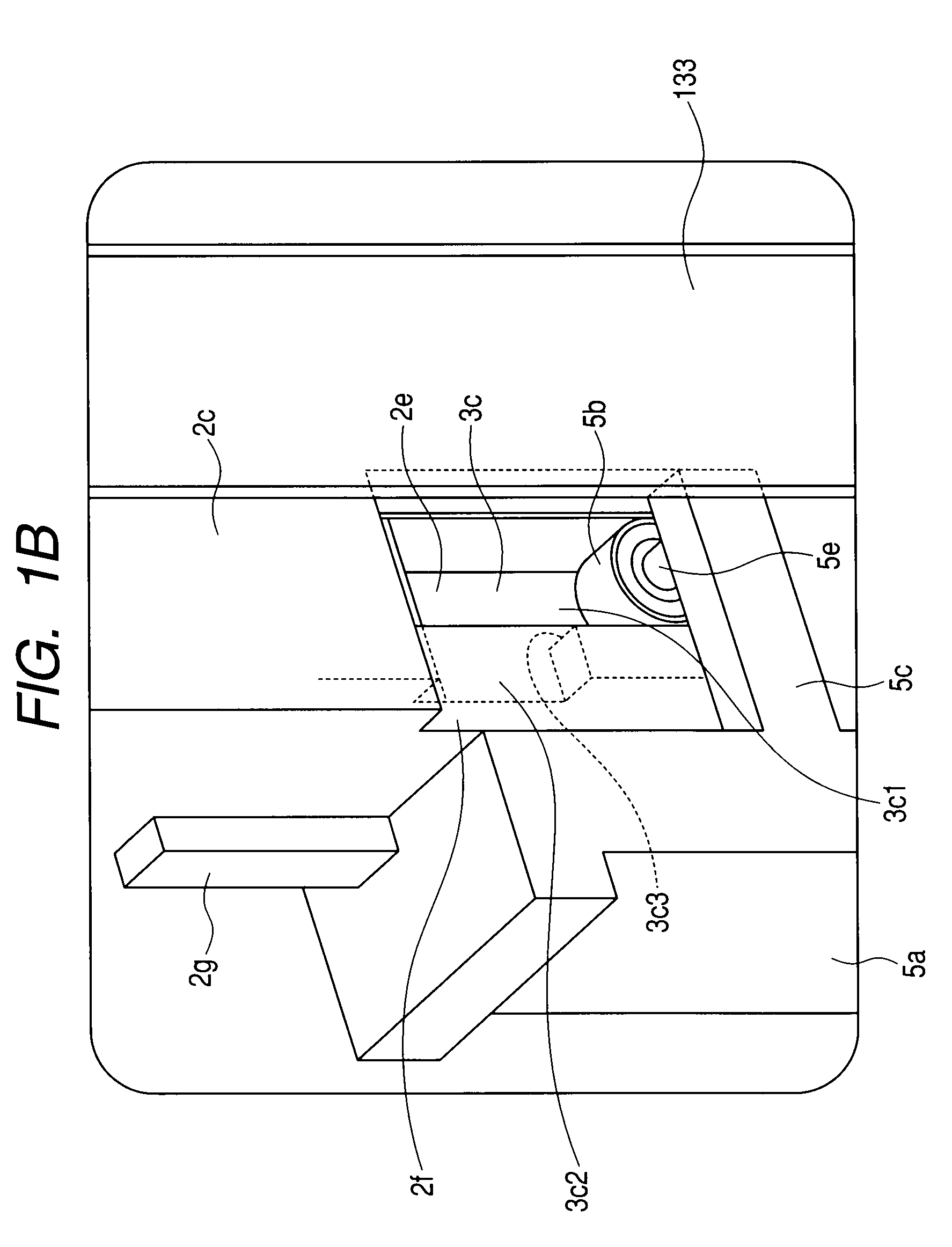
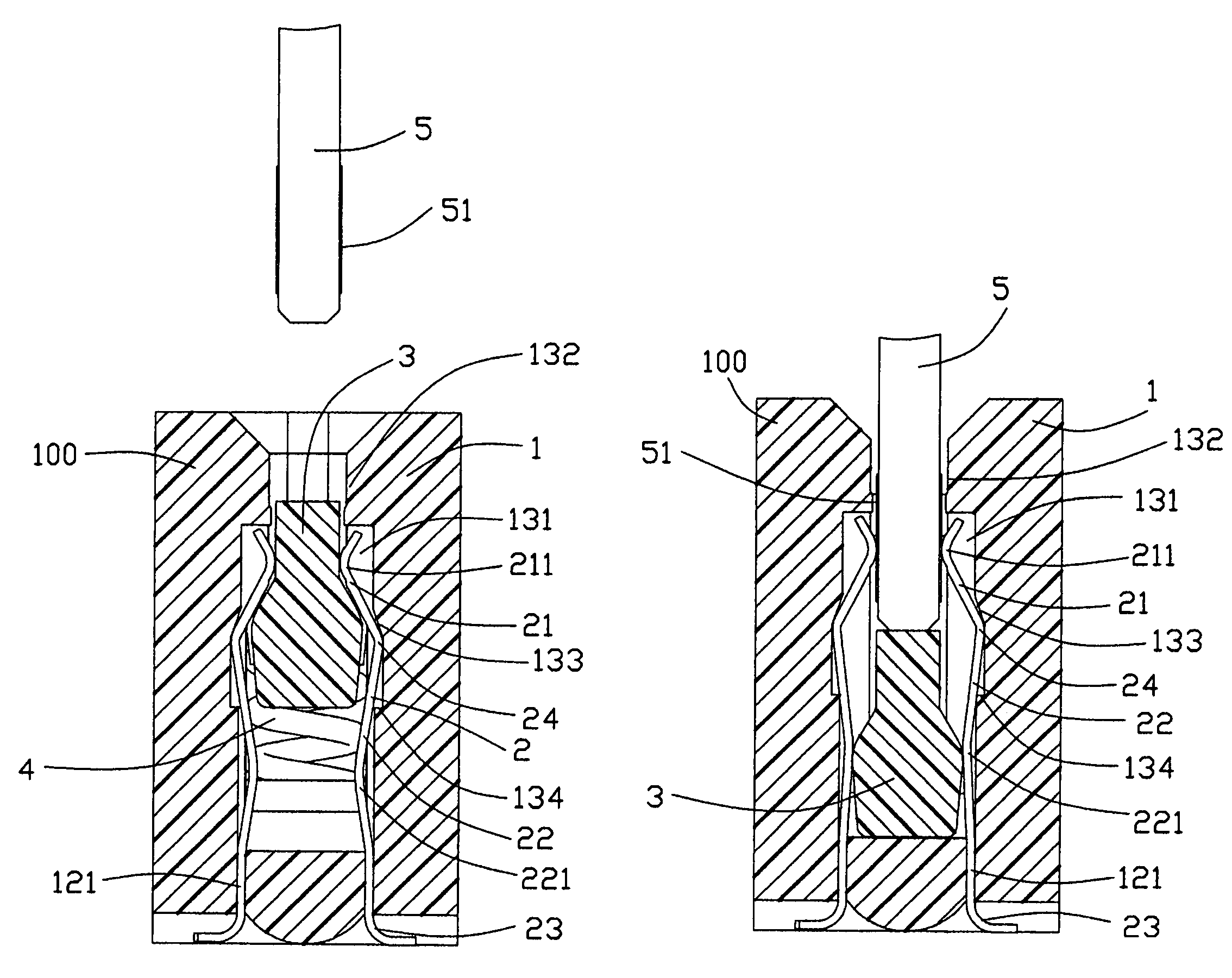
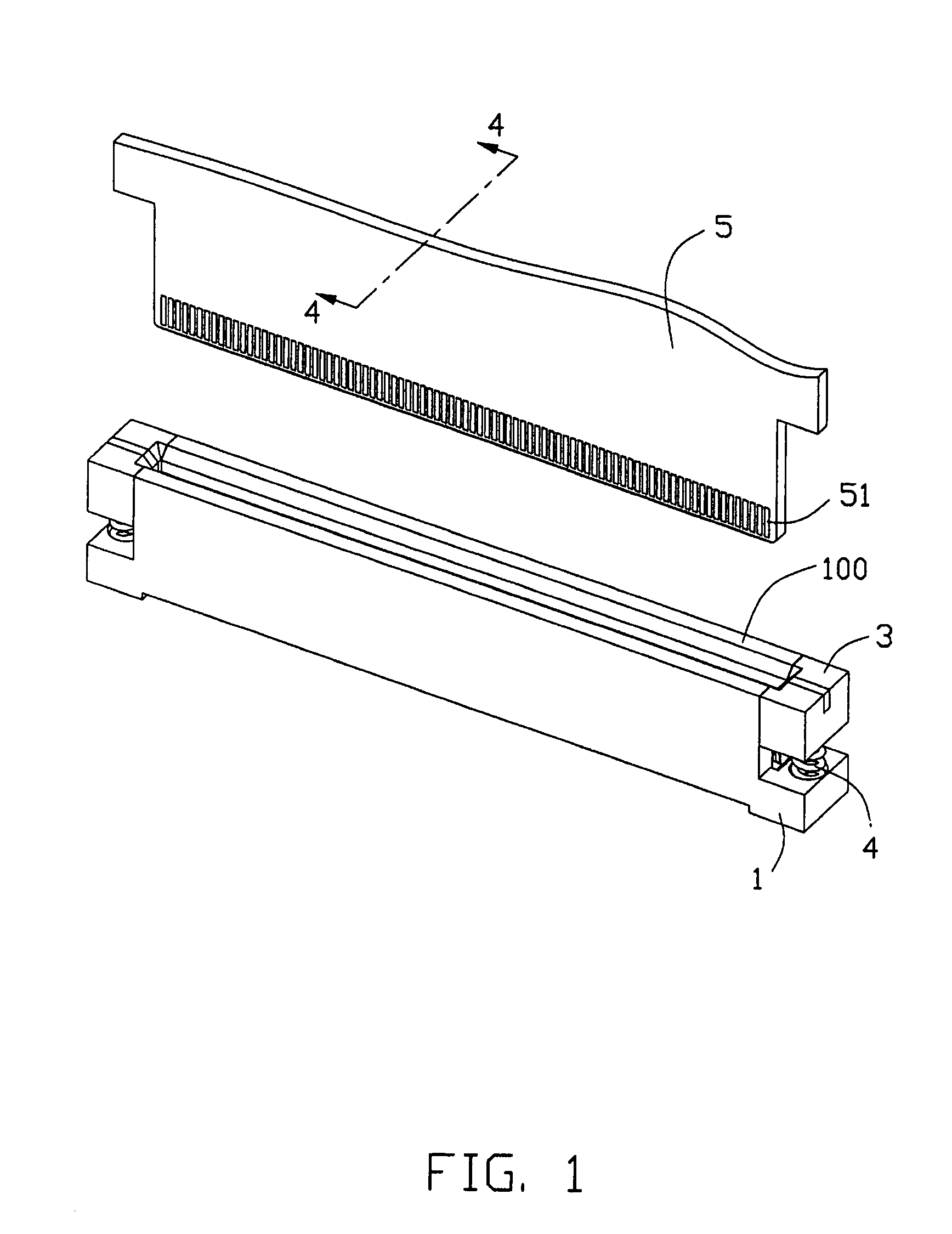
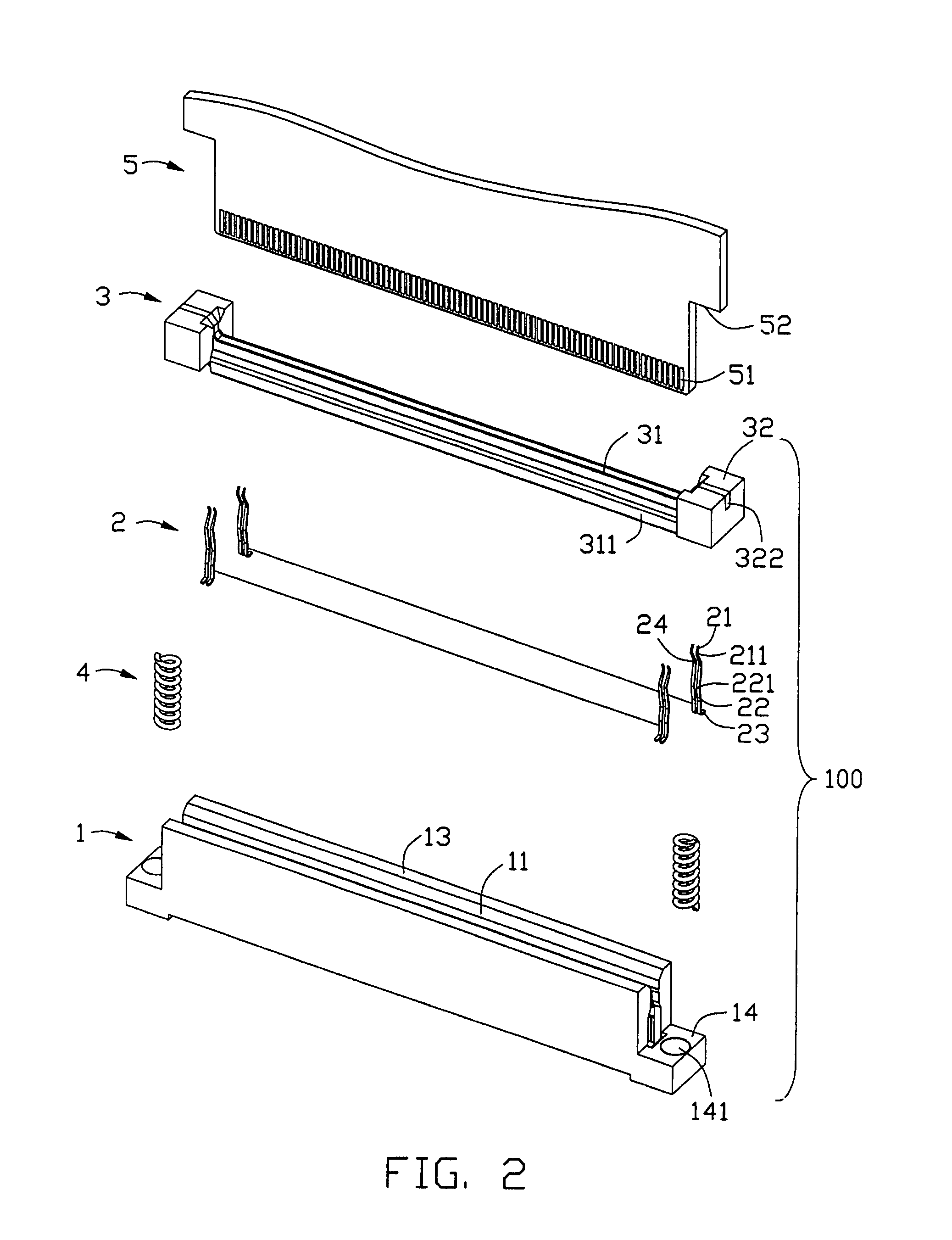
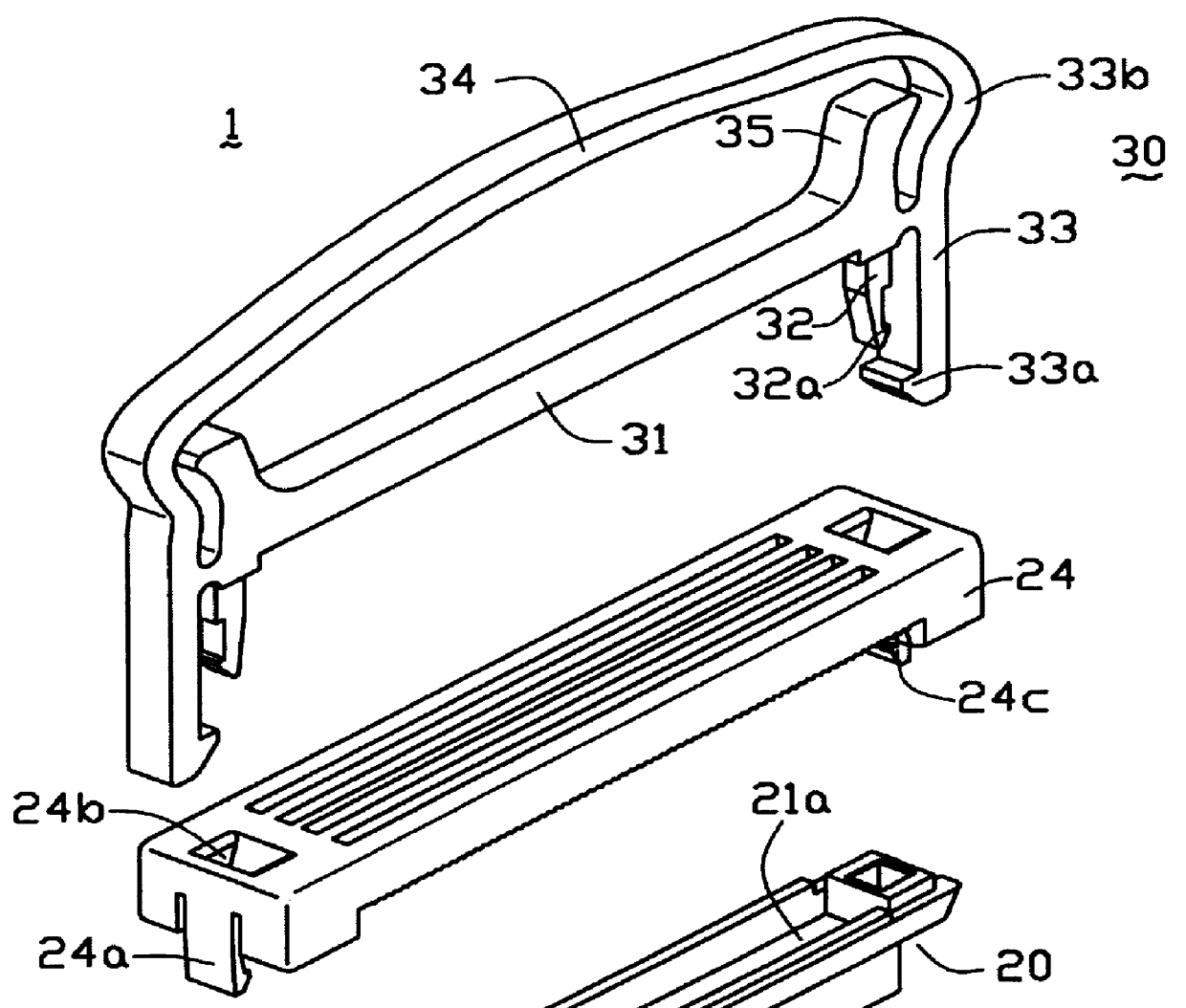
Card connector assembly having improved terminal
InactiveUS7390208B1Reliable engagementEasy extractionEngagement/disengagement of coupling partsCoupling contact membersEngineering
A card connector assembly (100) for insertion of an electronic card (5), includes an insulative housing (1) defining therein a slot (11) and a number of passageways (131) communicating with the slot, and a number of terminals (2) retained in the passageways. Each terminal includes a contact portion (21) exposed in the slot for contacting with the electronic card, and a deformable resilient beam (22) having a depressible portion (221) away from the contact portion. The depressible portions are outwardly depressed due to an insertion of the electronic card, and are inwardly relaxed due to an extraction of the electronic card.
Owner:HON HAI PRECISION IND CO LTD
Electrical connector assembly having protection partition
InactiveUS6132241AIncrease forceReliable engagementRelieving strain on wire connectionContact members penetrating/cutting insulation/cable strandsElectrical connectorPrinted circuit board
An electrical connector assembly, comprises a first connector assembled to a printed circuit board and a second connector releasably mated thereto. The second connector mated to the first connector includes a second housing defining a cavity for receiving an island portion of the first connector. The second housing forms a pair of mounting wedges at ends thereof and retaining recesses adjacent to the mounting wedges. A termination cover assembled to the second connector includes a pair of mounting lugs engaged to the mounting wedges. A pair of passages defined in the cover adjacent to the mounting lugs. A strain relief assembled to the termination cover forms inner latches engaged to the retaining recess and outer latches pivotally hinged to the base bar. The outer latches are releasably received in the retaining recess of the first connector when the second connector is mated to the first connector.
Owner:HON HAI PRECISION IND CO LTD
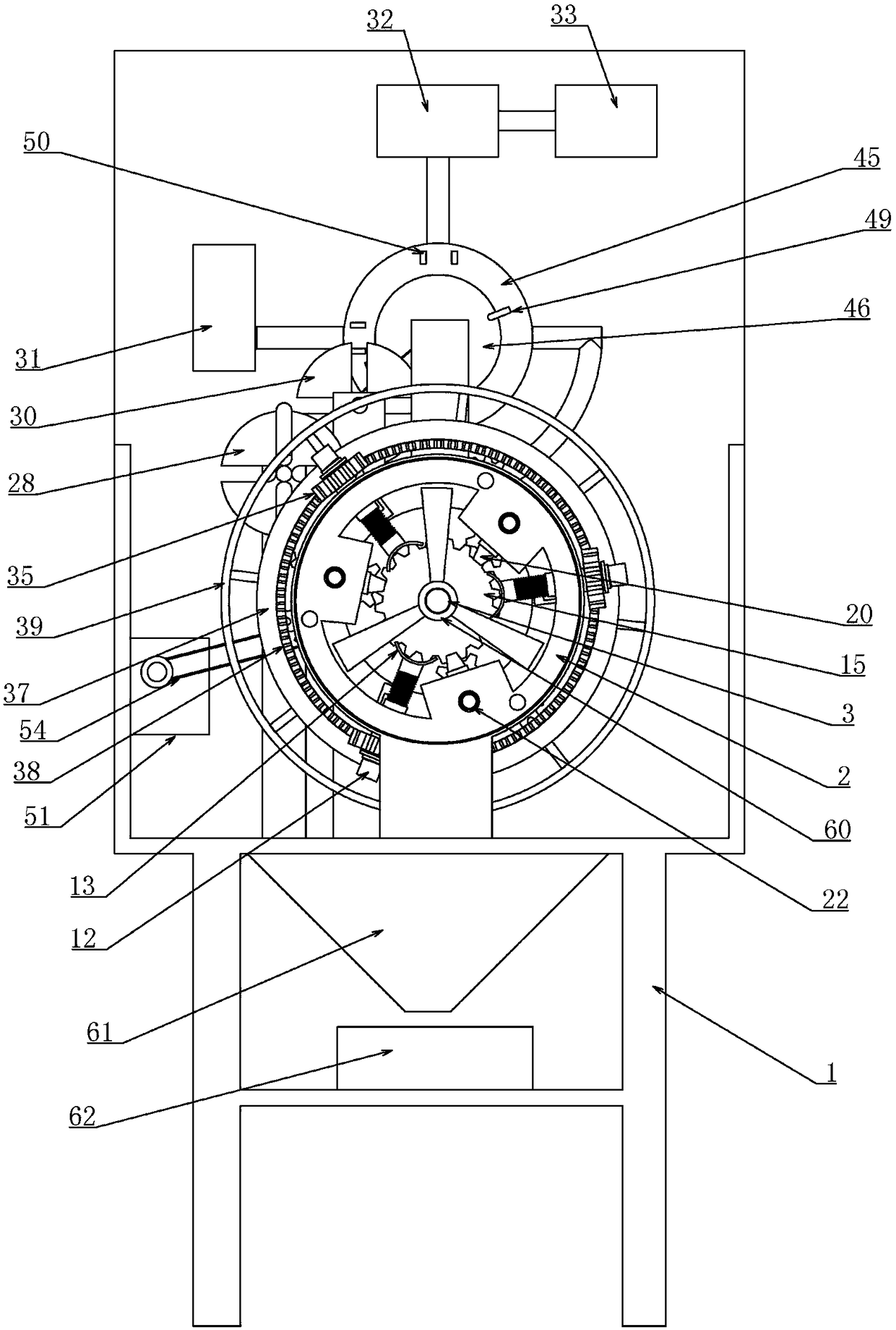
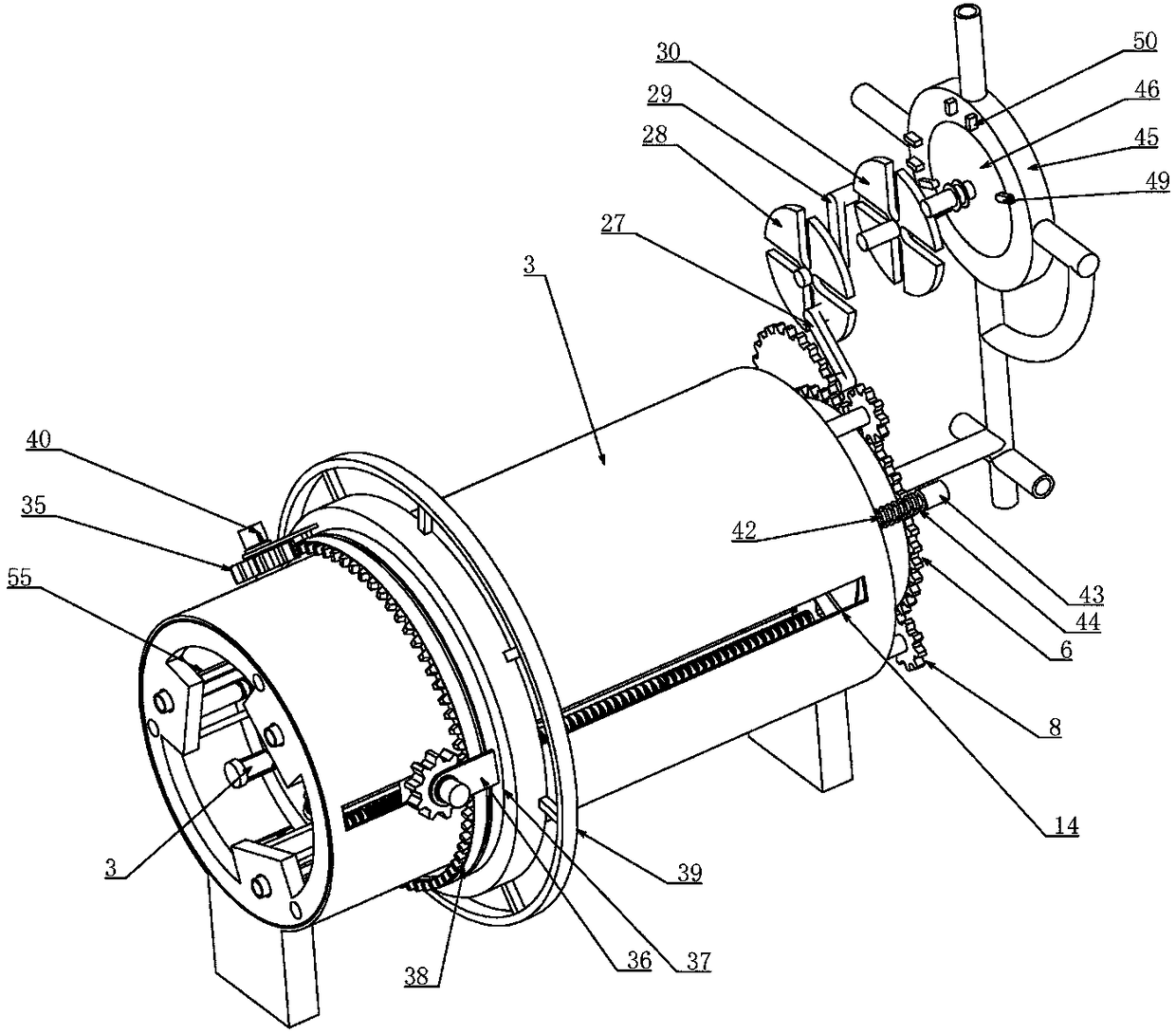
Apparatus cleaning device for pneumology department
ActiveCN109482584AIngenious designEasy to operateDiagnosticsHollow article cleaningEngineeringTracheal intubation
The invention relates to an apparatus cleaning device for the pneumology department. According to the apparatus cleaning device for the pneumology department, a plurality of sets of planetary wheel structures are ingeniously applied, and the structural specificity of an external gear ring is utilized to convert forward and reverse rotating of a motor into different working processes; through the incompleteness of inner teeth of the external gear ring, when the external gear ring is about to be disengaged from a driven gear at a meshing terminal, a set of pre-tensioning device is added, and then the external gear ring is limited at the position of the meshing terminal of the external gear ring and the driven gear wheel, and reliable meshing can be realized when the external gear ring rotatereversely; and meanwhile, transition grooves and rectangular grooves in different positions are made alternately communicate through relative rotation of a circle plate and a fixing plate, and different processes are achieved. According to the apparatus cleaning device for the pneumology department, the transmission characteristics of a screw rod and a reciprocating screw rod are fully utilized to realize reciprocating characteristics of tracheal intubation and cleaning and drying work, the design is ingenious, the operation is simple, cleaning personnel are liberated greatly, working difficulty of the cleaning personnel is lowered, and the cleaning effect is excellent.
Owner:汪铮
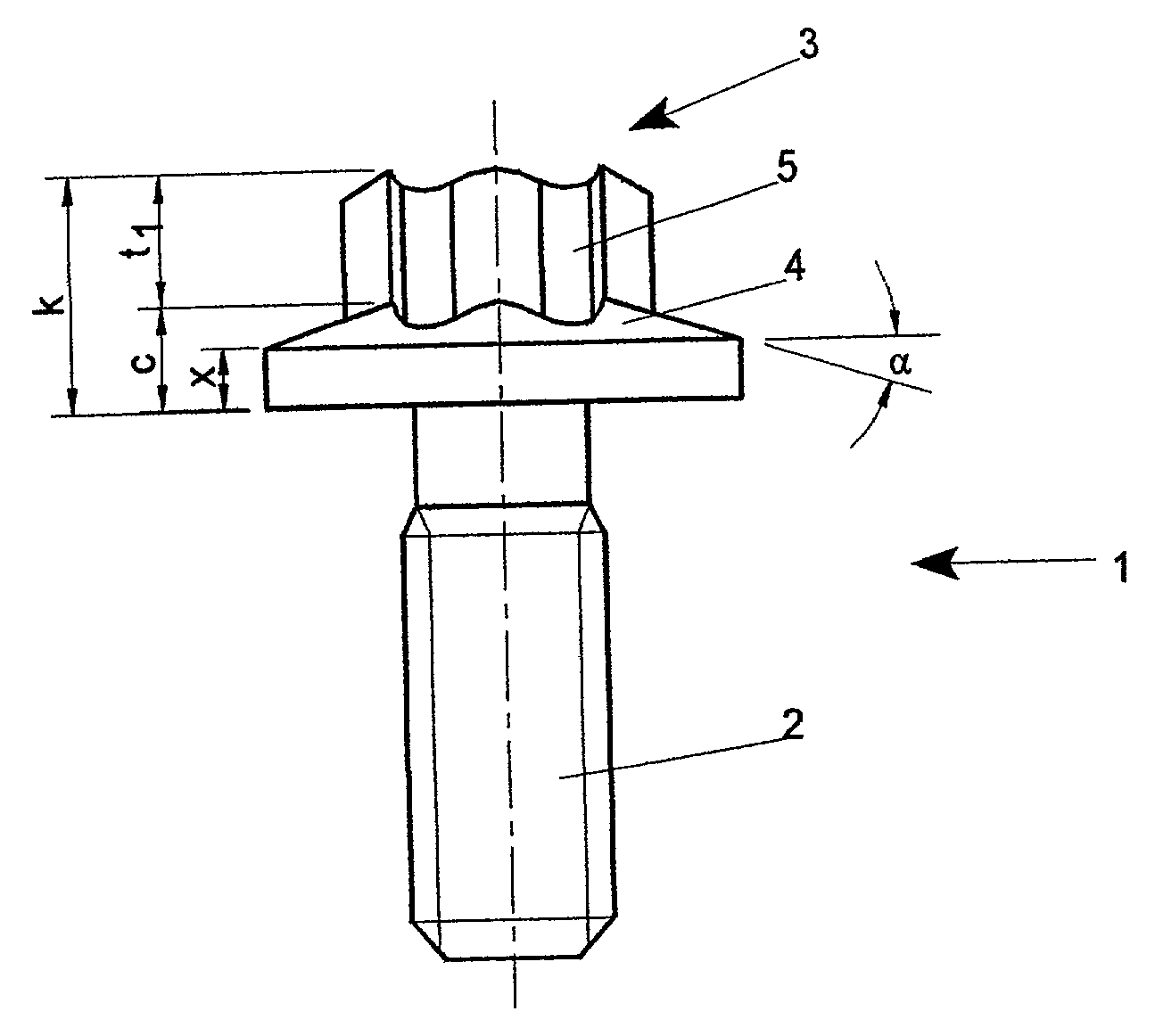
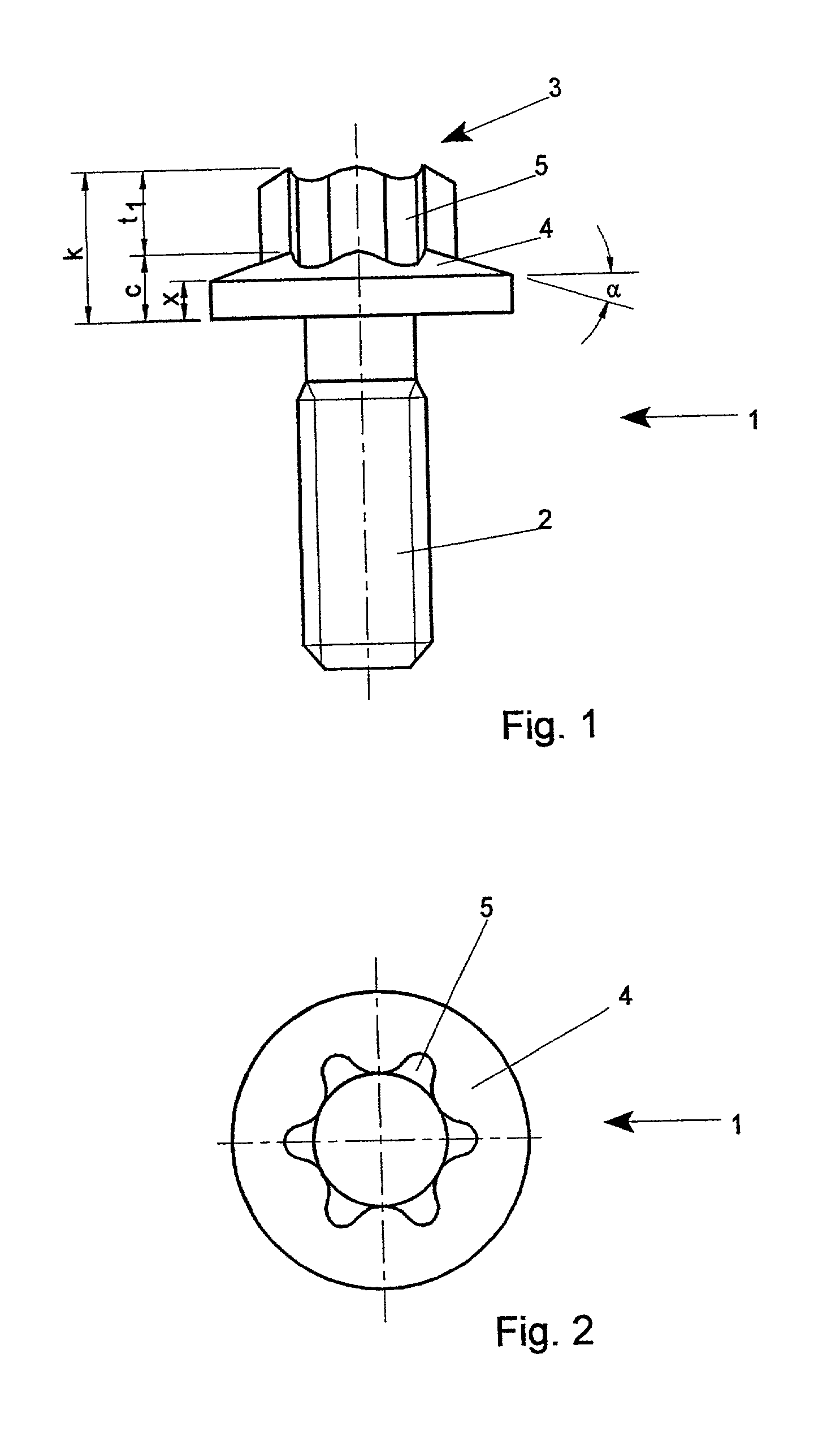
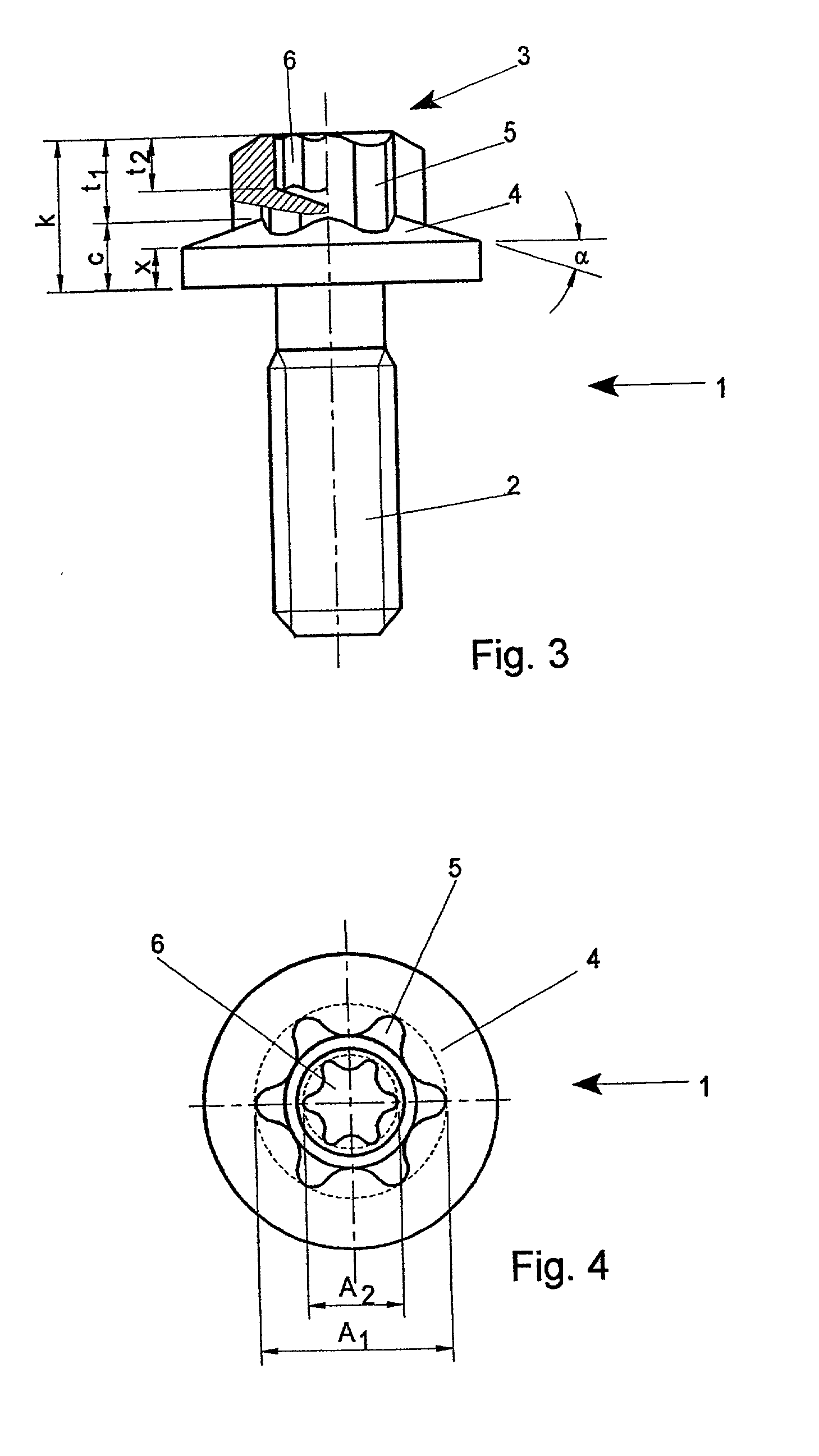
Round hexagon screw and method for its production
The invention concerns an outer hexagon round screw and a method for production of the screw. The screw cap is formed here essentially in the kind of a Torx head, with an outer hexagon round contour (5) and a flange (4) having a slope (4.1) reaching in the direction of the outer hexagon round contour (5) under an angle (alpha) thereby assuring a force engagement with a tool. The slope (4.1) of the flange (4) follows immediately to the outer hexagon round contour (5) such that the overall height (k) of the head (3) is substantially decreased, wherein at the same time the height (t1) of the outer hexagon round contour (5) assures a reliable force engagement. The production of the flange (4) and of the outer hexagon round contour (5) is performed either by scraping with a scraping tool or by head flattening or upset compressing or compression molding, wherein additionally a socket is formed in the head region. (FIG. 1).
Owner:ESKA SACHSISCHE SCHRAUBENWERKE
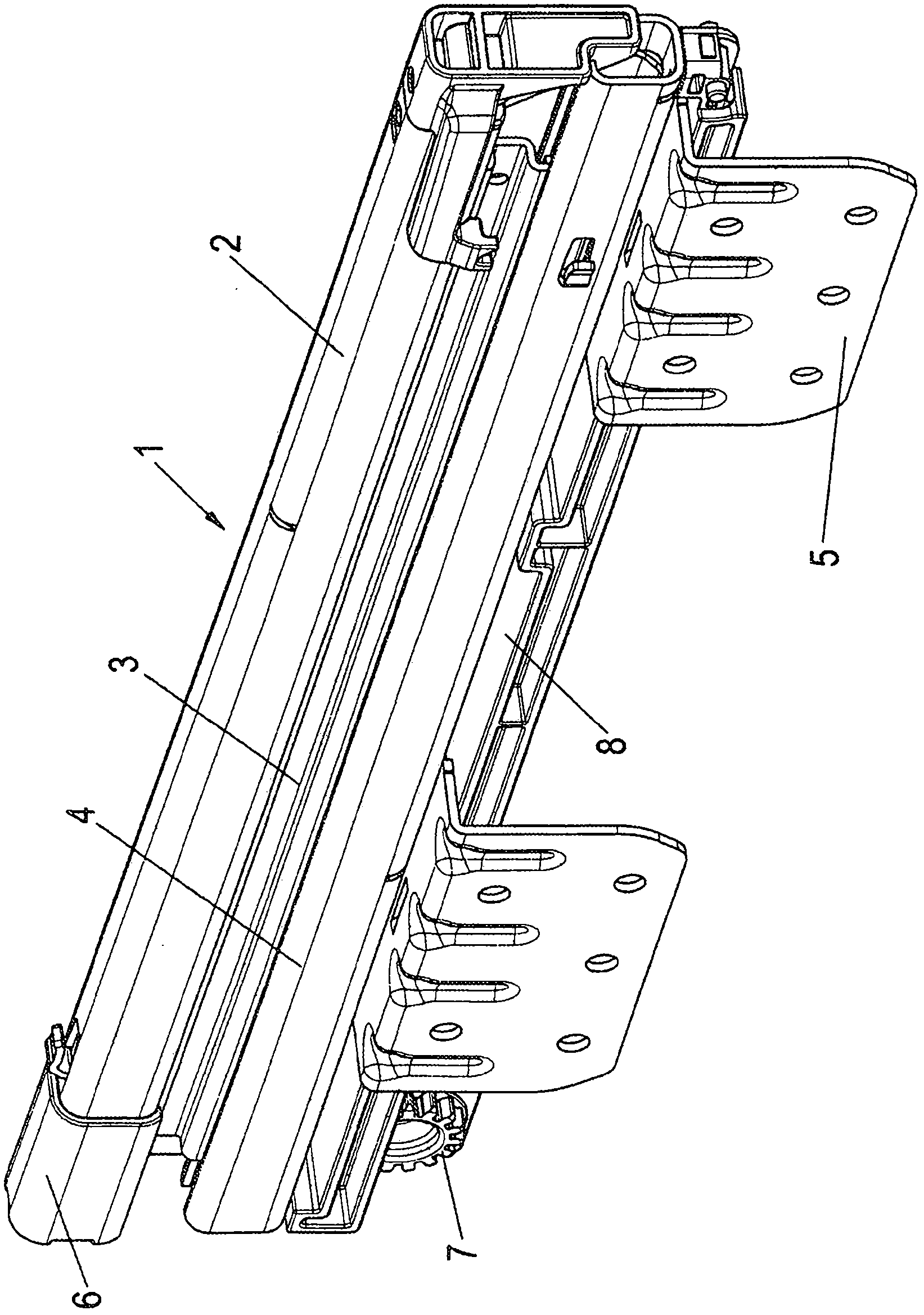
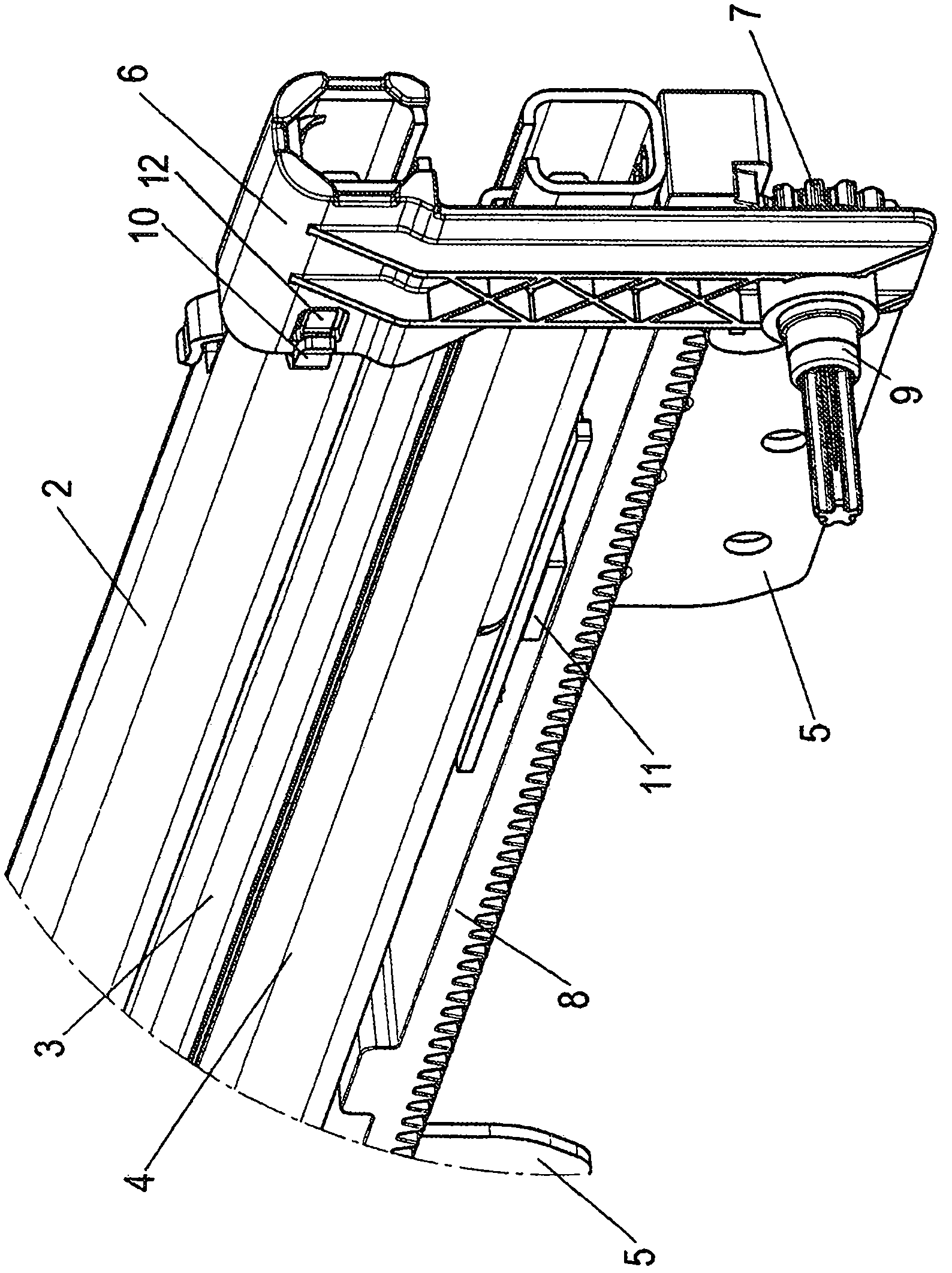
Synchronous guide of a push member and piece of furniture
ActiveCN102469879AEasy to installReliable engagementMechanical apparatusLighting and heating apparatusGear wheelEngineering
Synchronous guide of a push member (25) mounted in a body (24) of a piece of furniture or household appliance by way of pullout guides (1), comprising two stationary toothed racks (8), which are located opposite to each other and associated with a side member of the body, and into which gear wheels (9) engage that are interconnected via coupling rods (26), wherein the gear wheels (9) are mounted in a stationary manner on the guide rails (2) of the pullout guides (1).
Owner:PAUL HETTICH
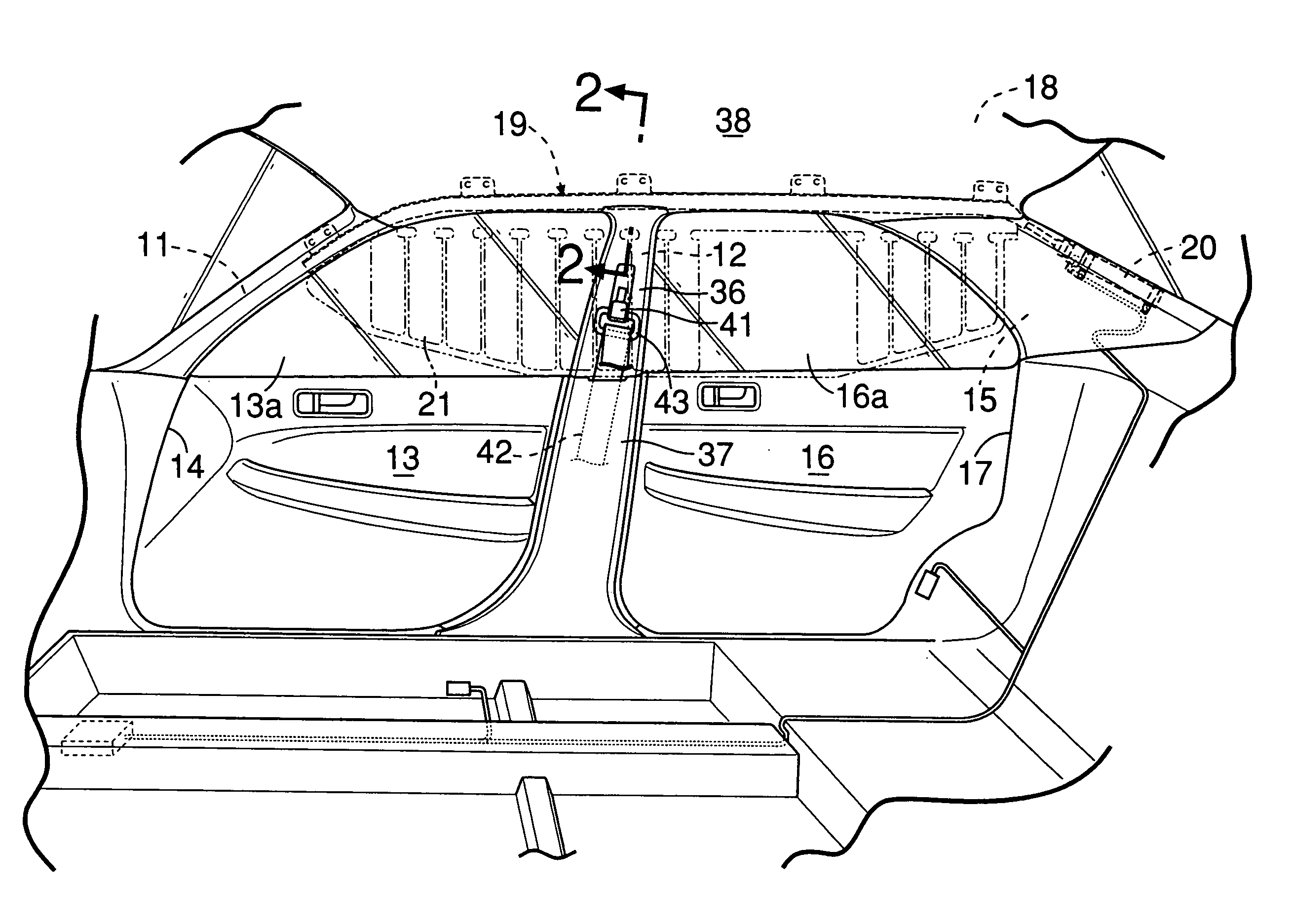
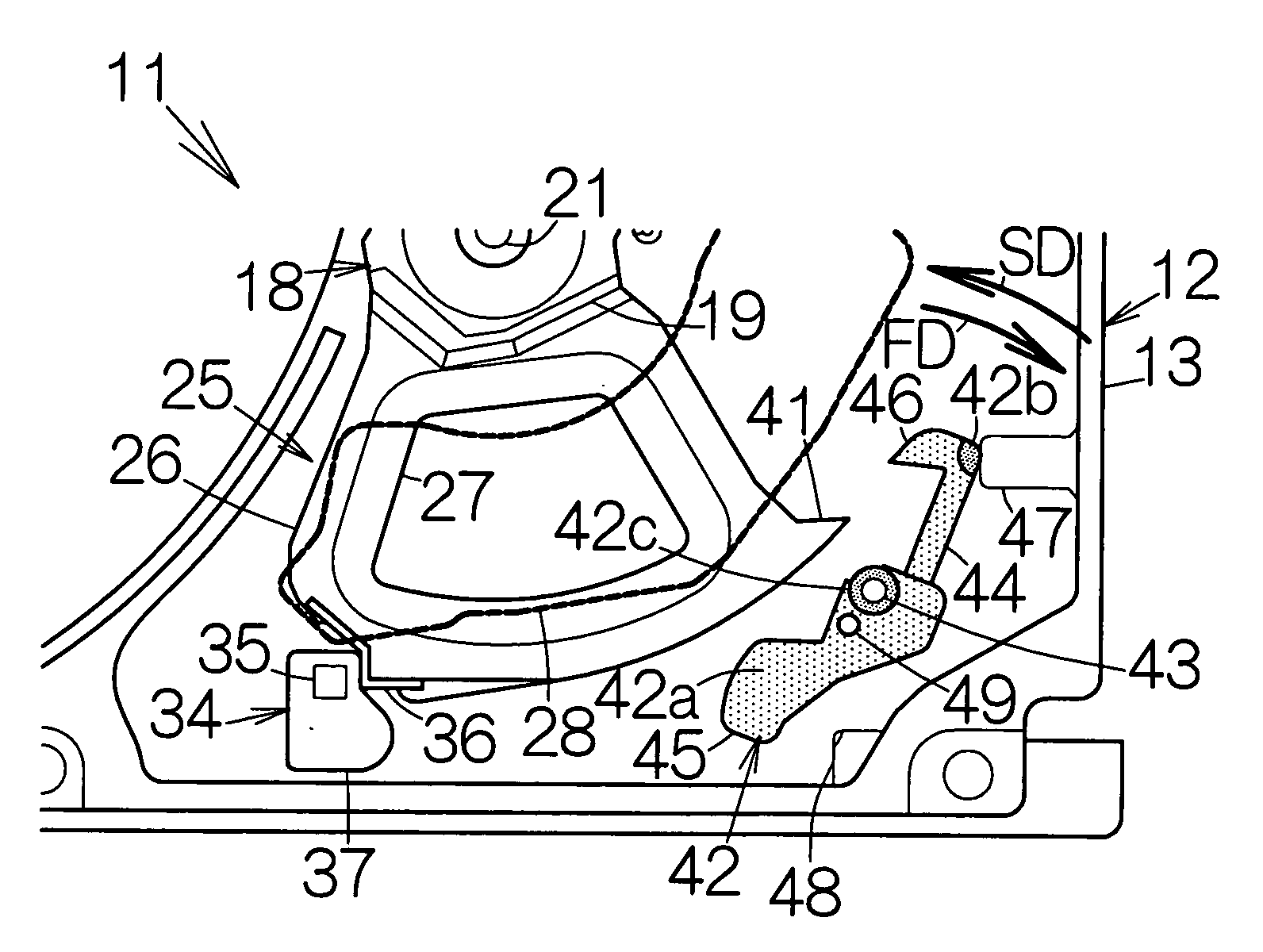
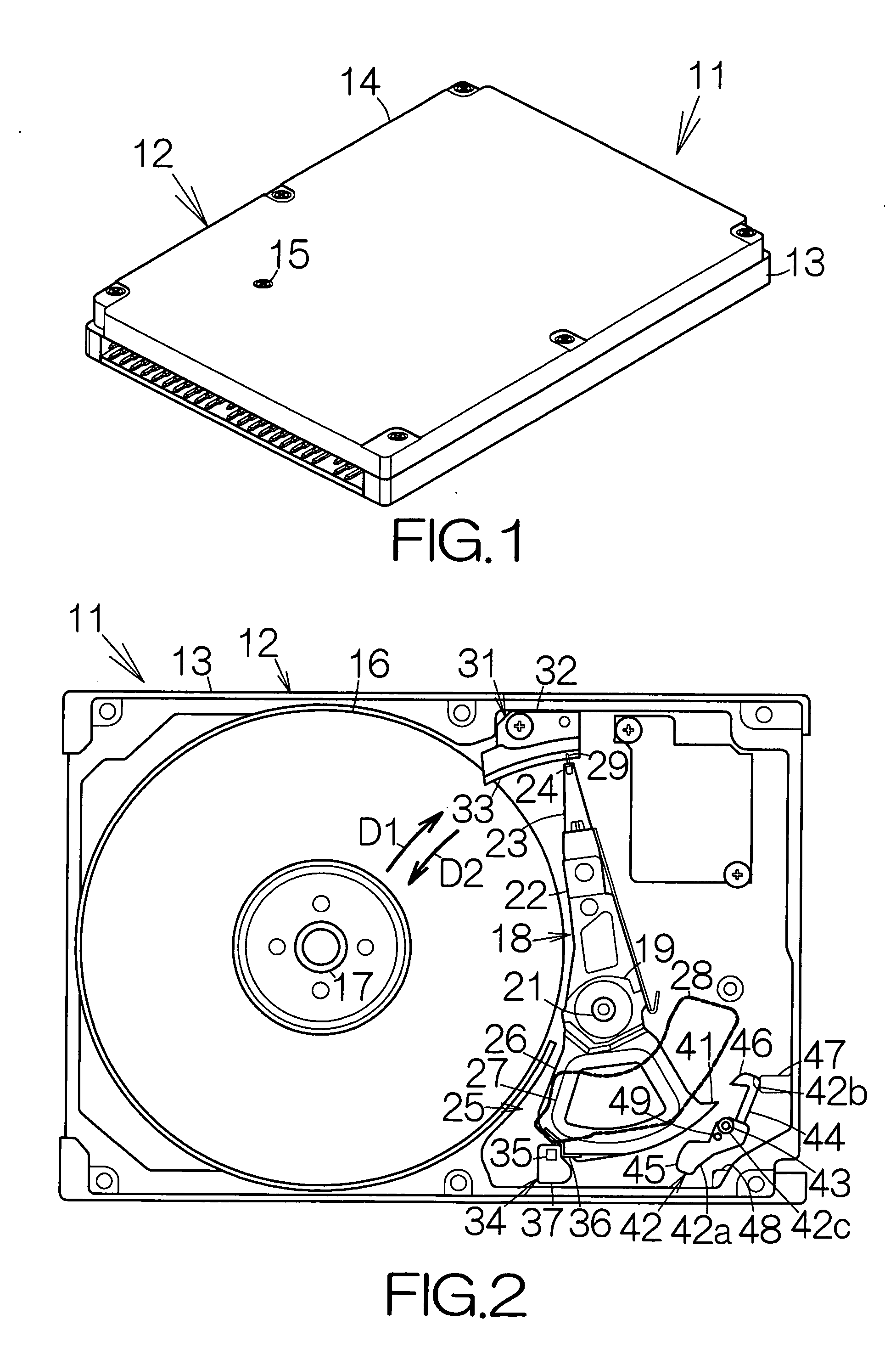
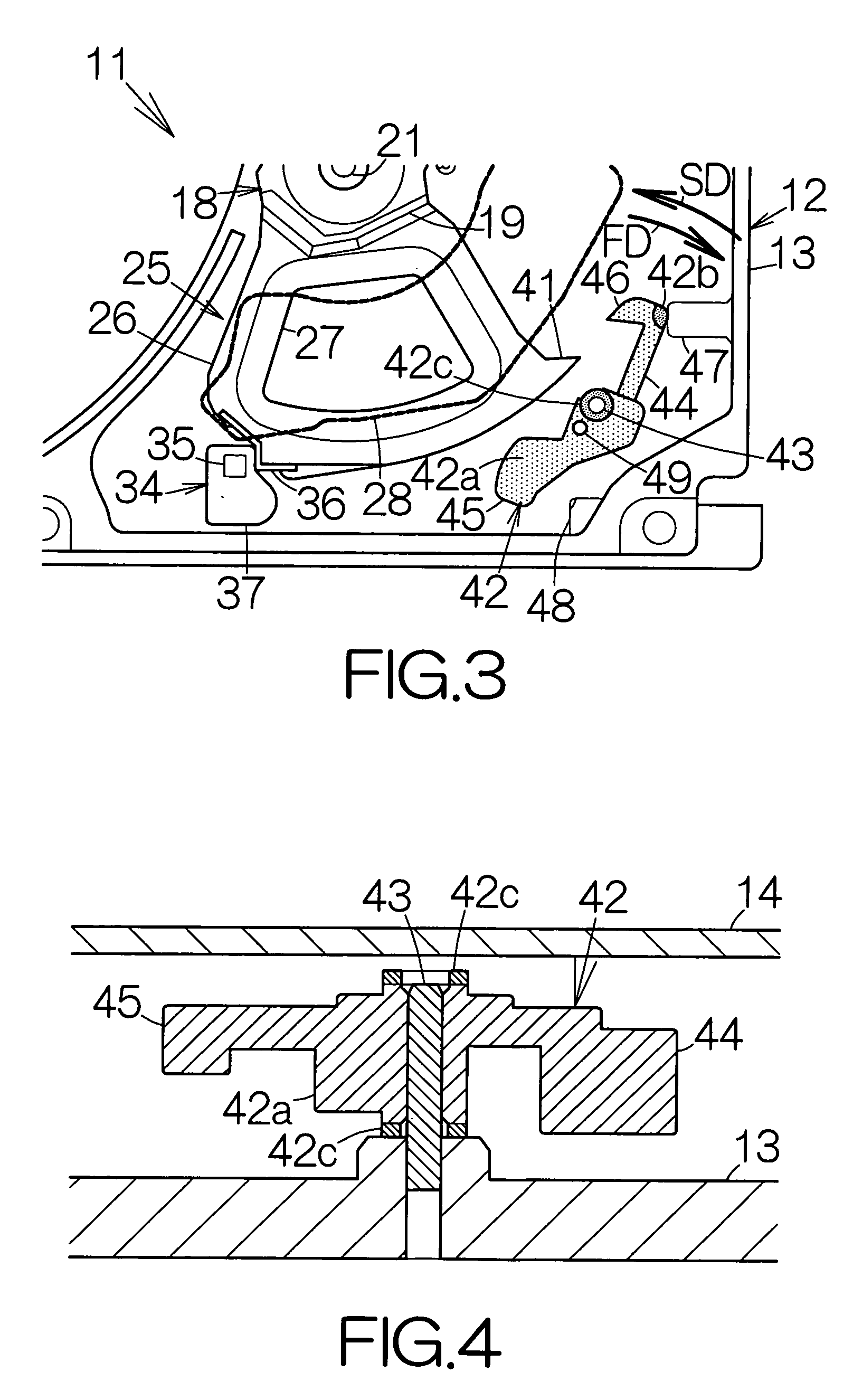
Occupant restraint system
ActiveUS7172211B2Good lookingEasy to assemblePedestrian/occupant safety arrangementSuperstructure subunitsEngineeringAirbag
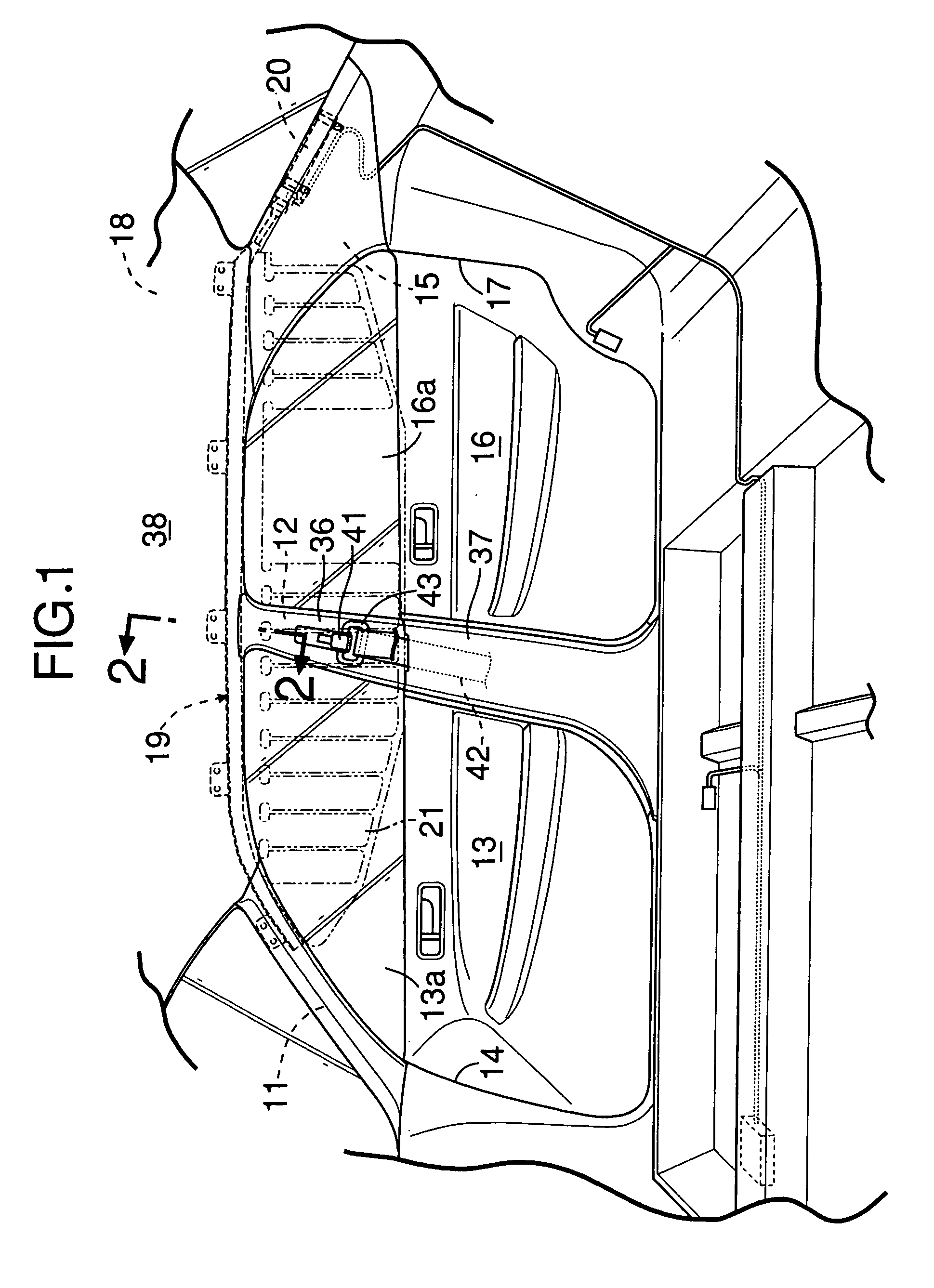
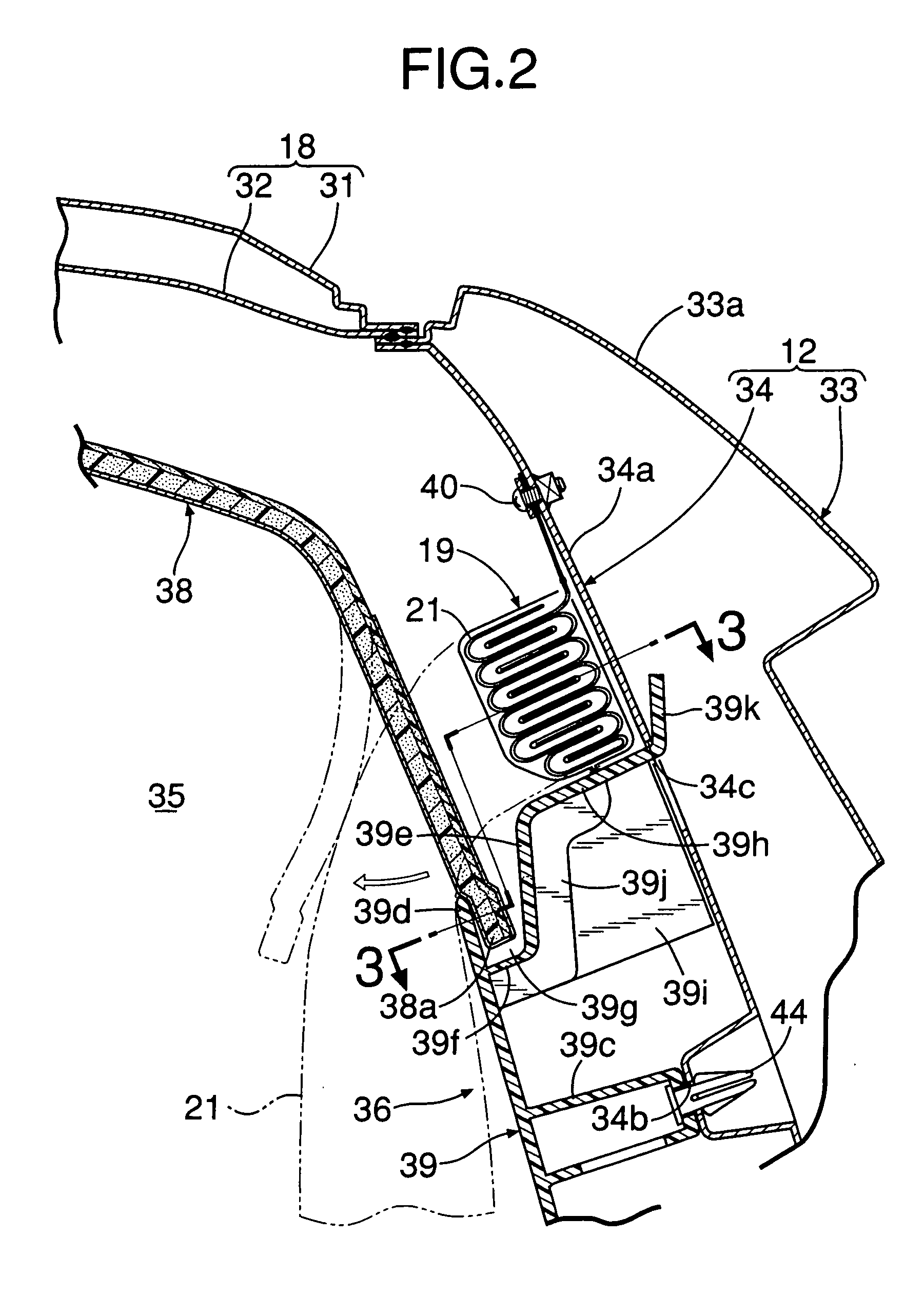
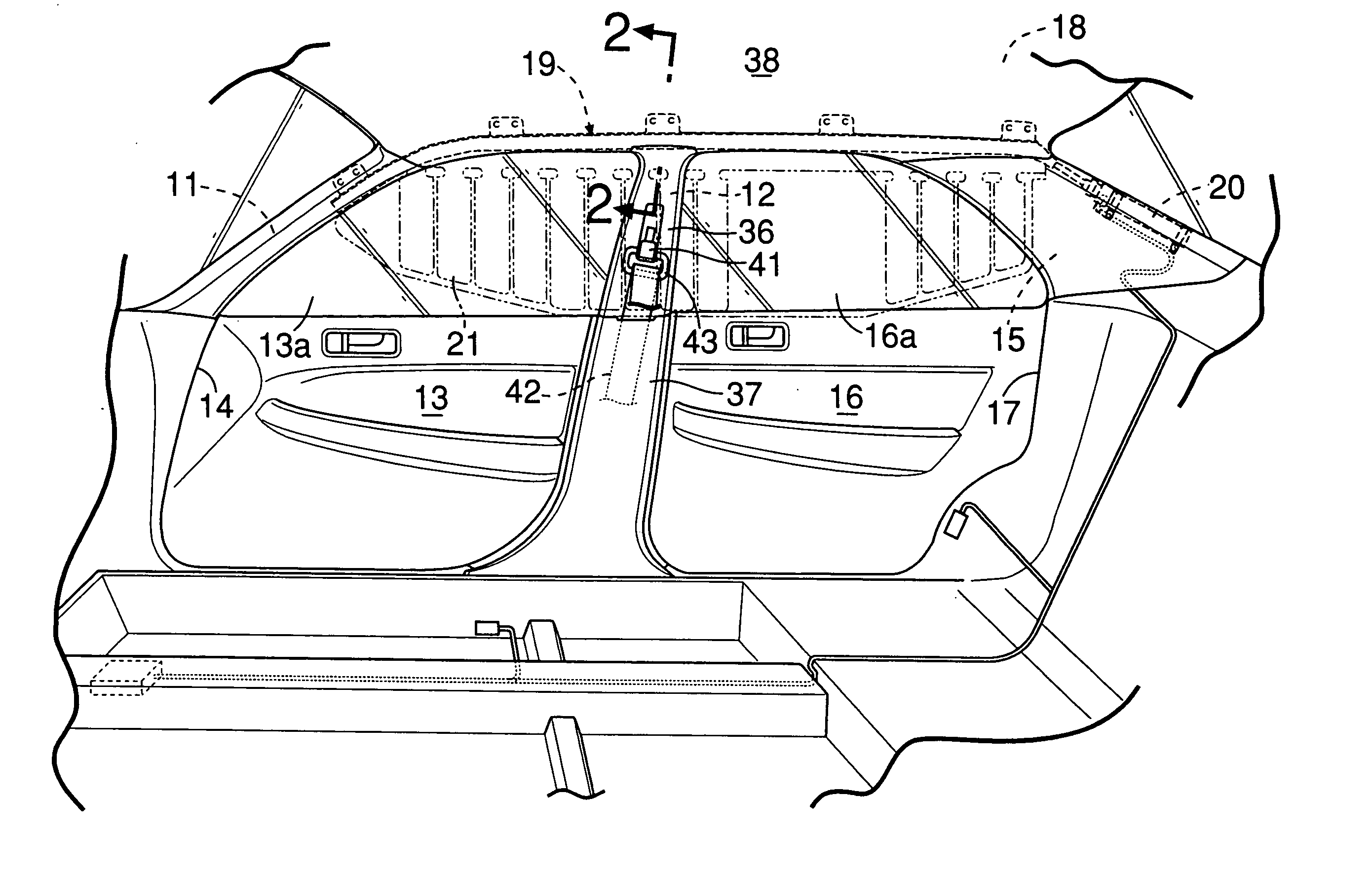
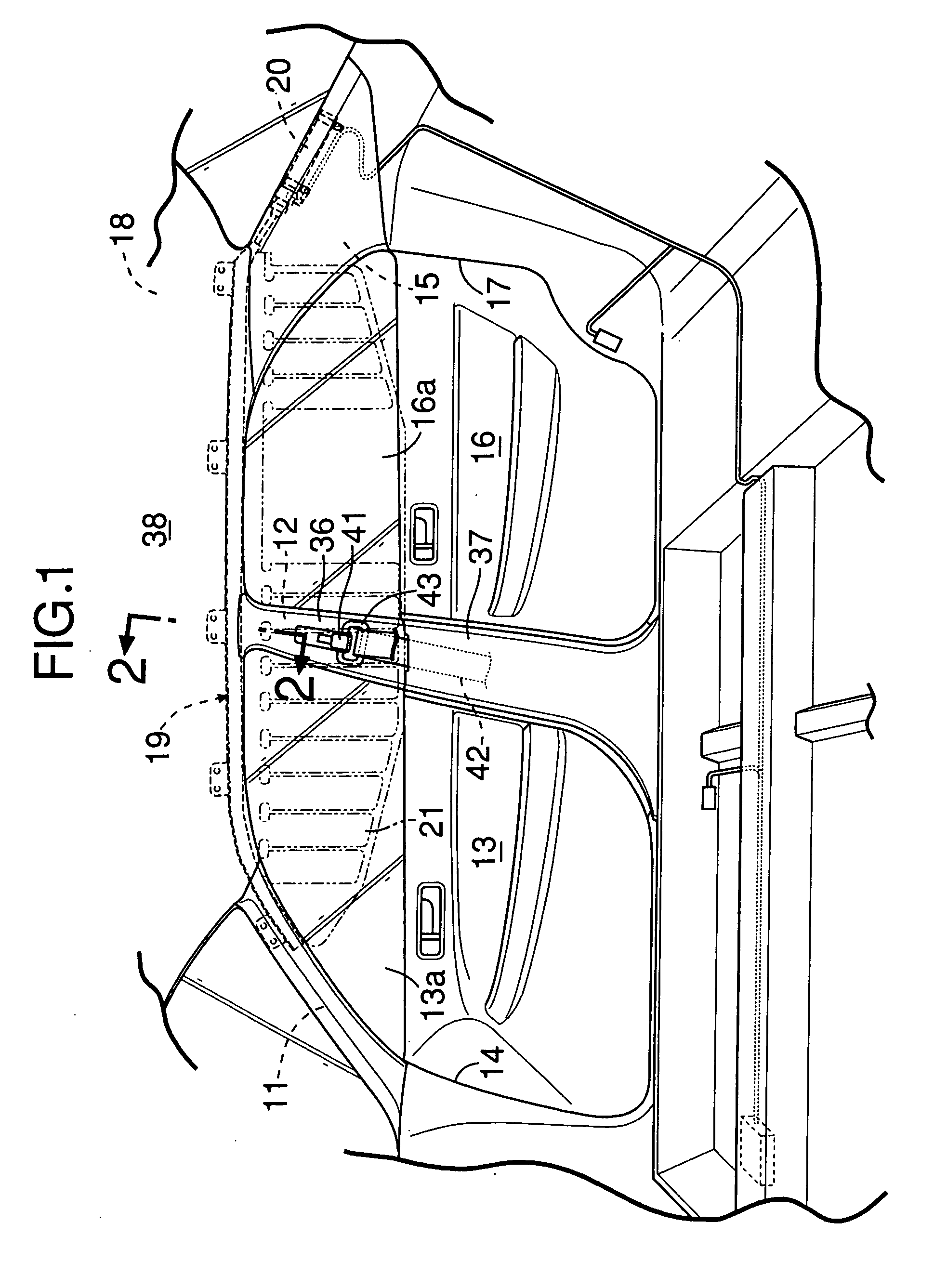
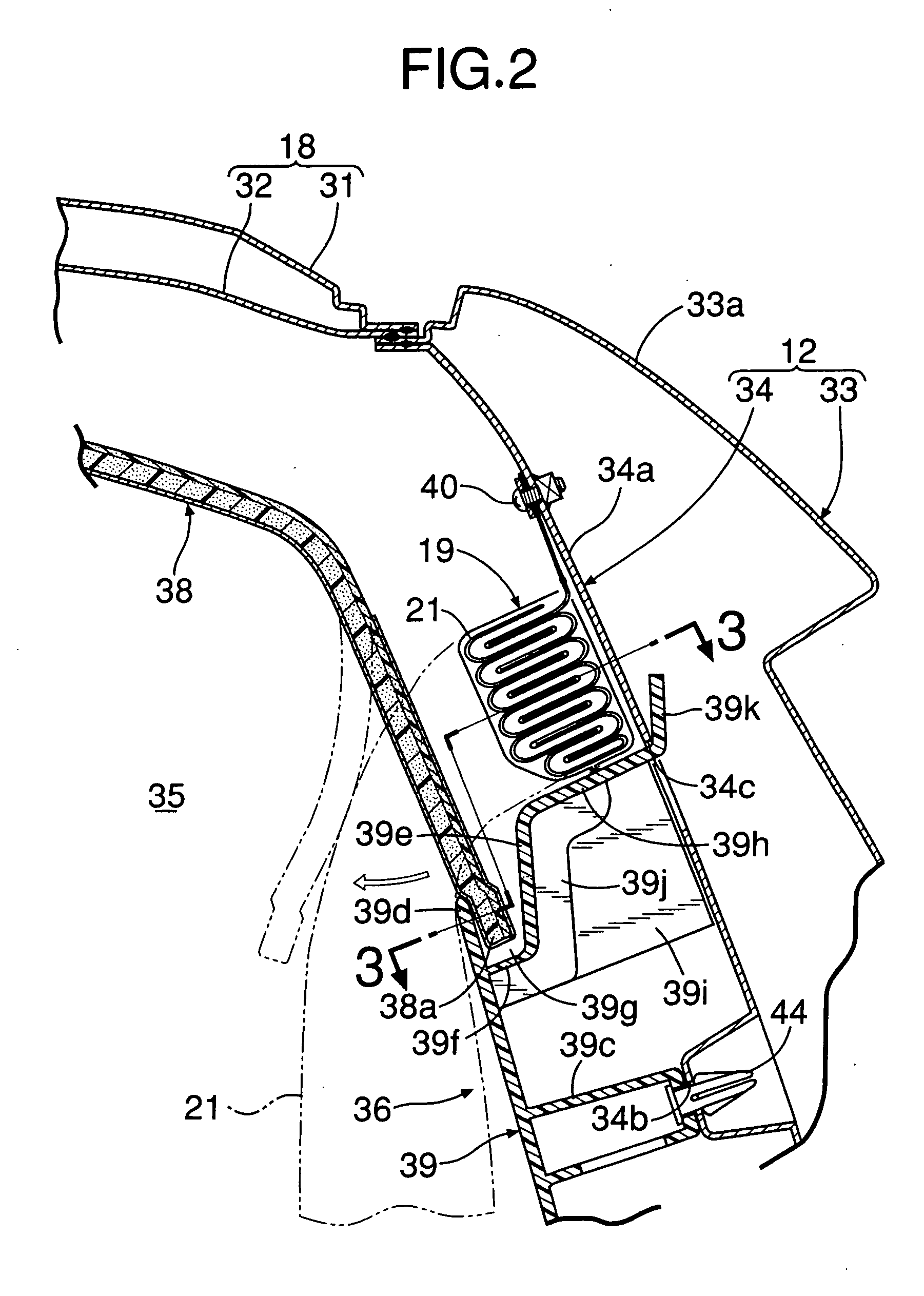
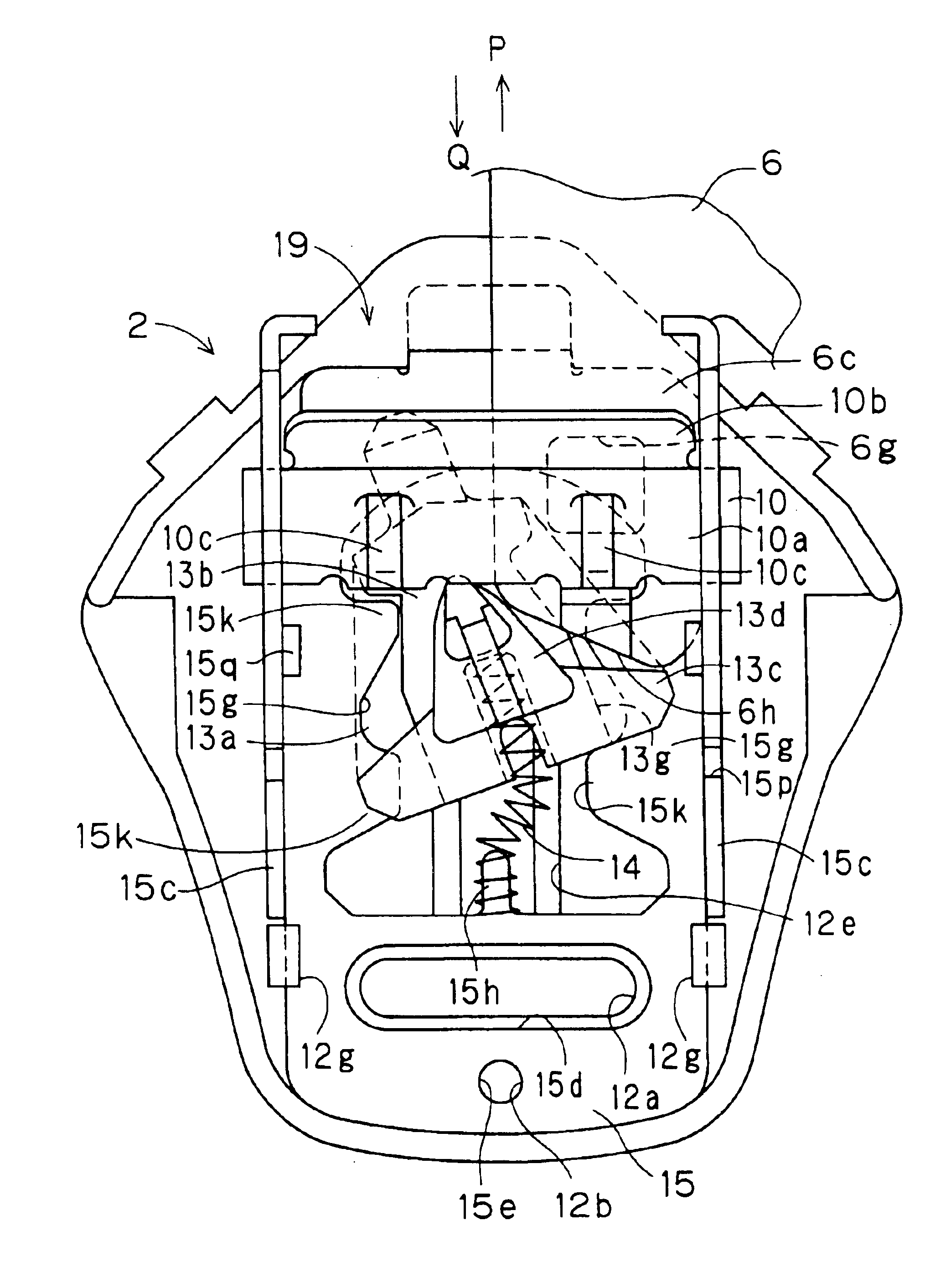
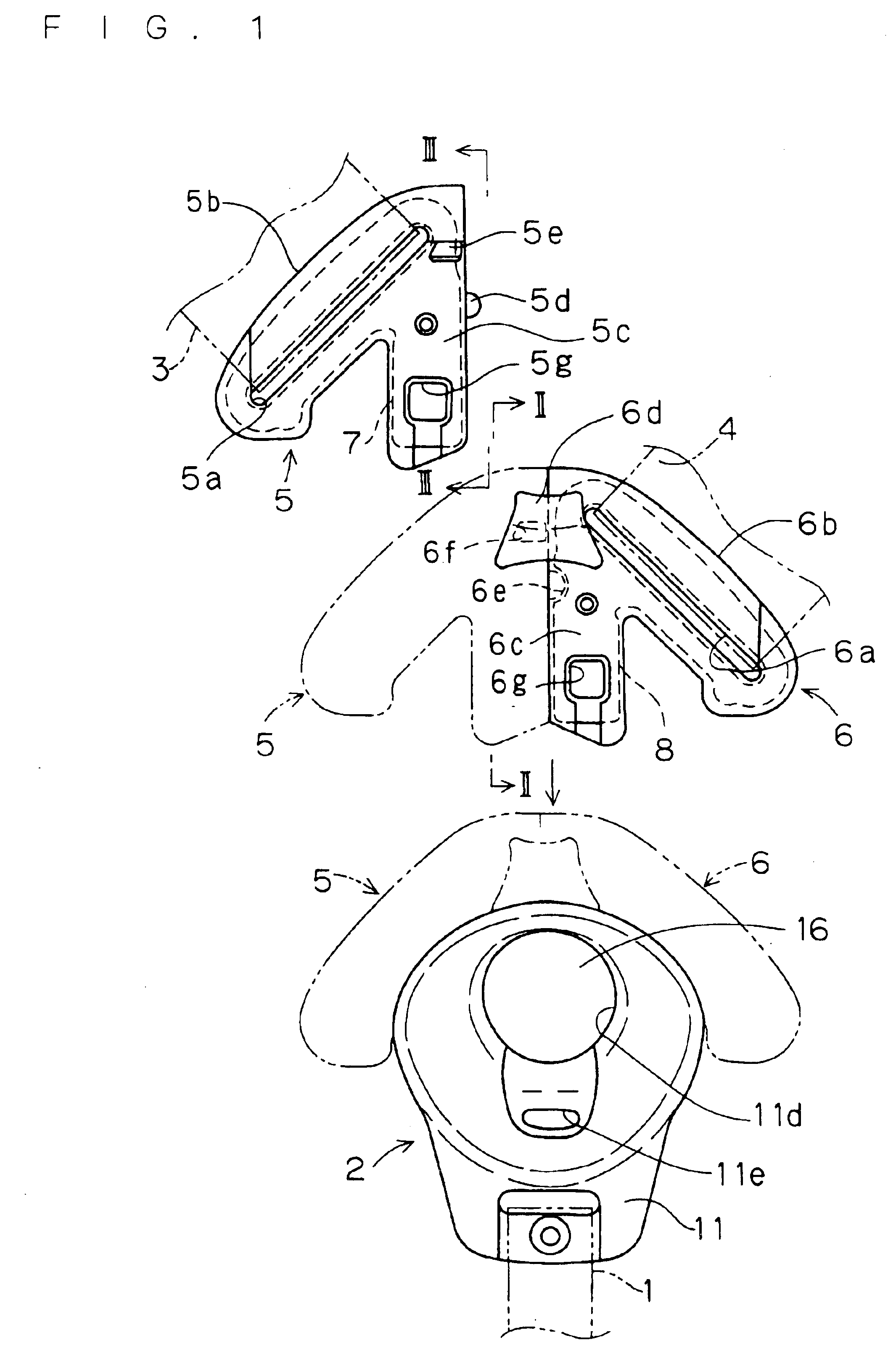
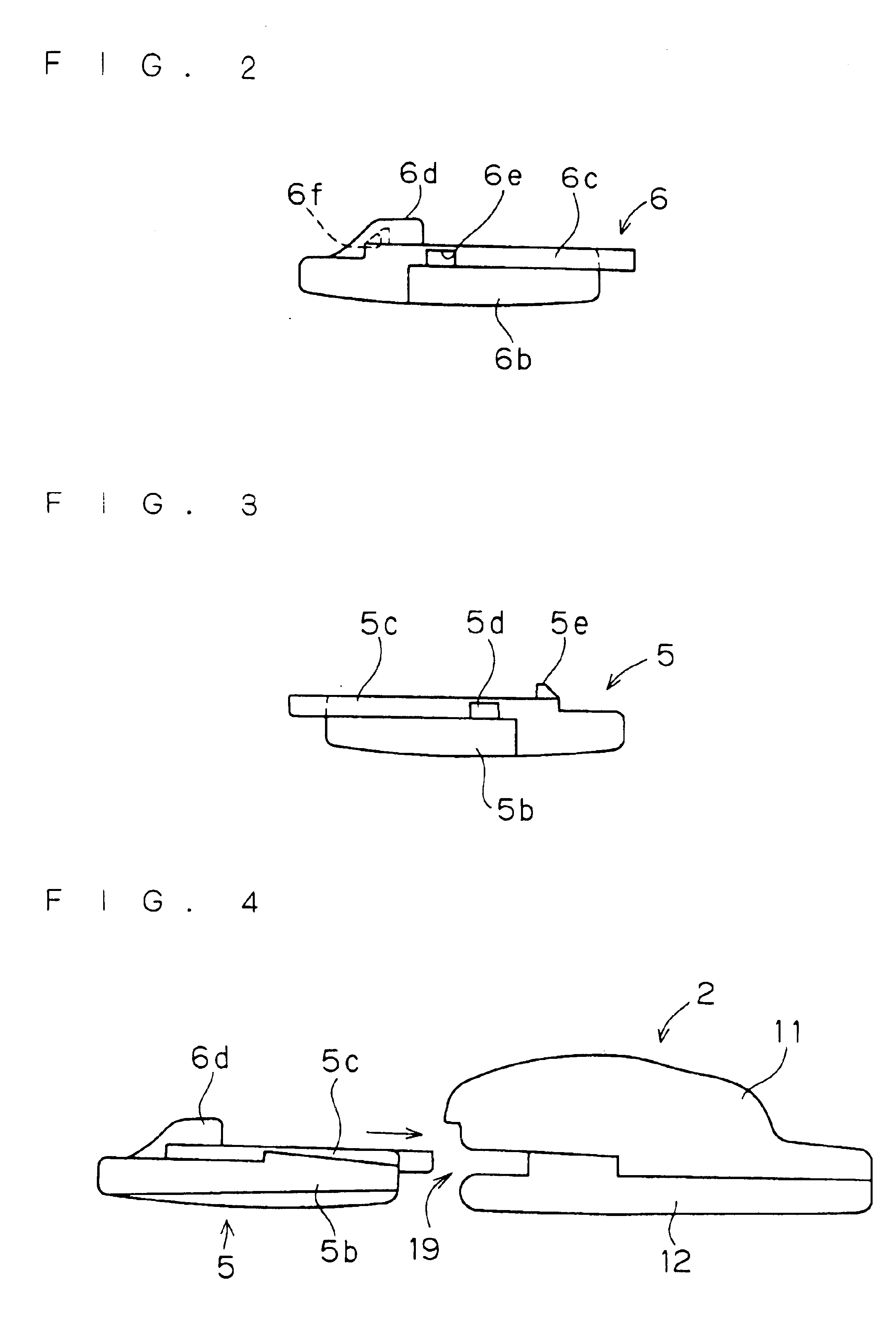
An end edge of a roof lining is engaged in a roof lining engaging groove extending along a side surface of an upper end portion of a pillar garnish at a side of a pillar, thereby eliminating a gap in a connecting portion between the pillar garnish and the roof lining to improve the appearance. Airbag deployment guide walls extend to an outside of a vehicle compartment from an upper end of a roof lining engaging groove at the side of the pillar, and an airbag module is disposed at a position sandwiched by the pillar and the roof lining, above the airbag deployment guide walls. Therefore, an inflating airbag is guided to the side of the vehicle compartment so as not to be caught by the upper end of the pillar garnish and can be deployed into the vehicle compartment by reliably pressing to open the roof lining, without providing a special guide member.
Owner:HONDA MOTOR CO LTD
Occupant restraint system
ActiveUS20050236818A1Good lookingEasy to assemblePedestrian/occupant safety arrangementSuperstructure subunitsEngineeringAirbag
An end edge of a roof lining is engaged in a roof lining engaging groove extending along a side surface of an upper end portion of a pillar garnish at a side of a pillar, thereby eliminating a gap in a connecting portion between the pillar garnish and the roof lining to improve the appearance. Airbag deployment guide walls extend to an outside of a vehicle compartment from an upper end of a roof lining engaging groove at the side of the pillar, and an airbag module is disposed at a position sandwiched by the pillar and the roof lining, above the airbag deployment guide walls. Therefore, an inflating airbag is guided to the side of the vehicle compartment so as not to be caught by the upper end of the pillar garnish and can be deployed into the vehicle compartment by reliably pressing to open the roof lining, without providing a special guide member.
Owner:HONDA MOTOR CO LTD
Overload protection arrangement for a rotatable power tool
InactiveUS6863165B2Reliable engagementMechanical actuated clutchesSlip couplingControl electronicsPortable power
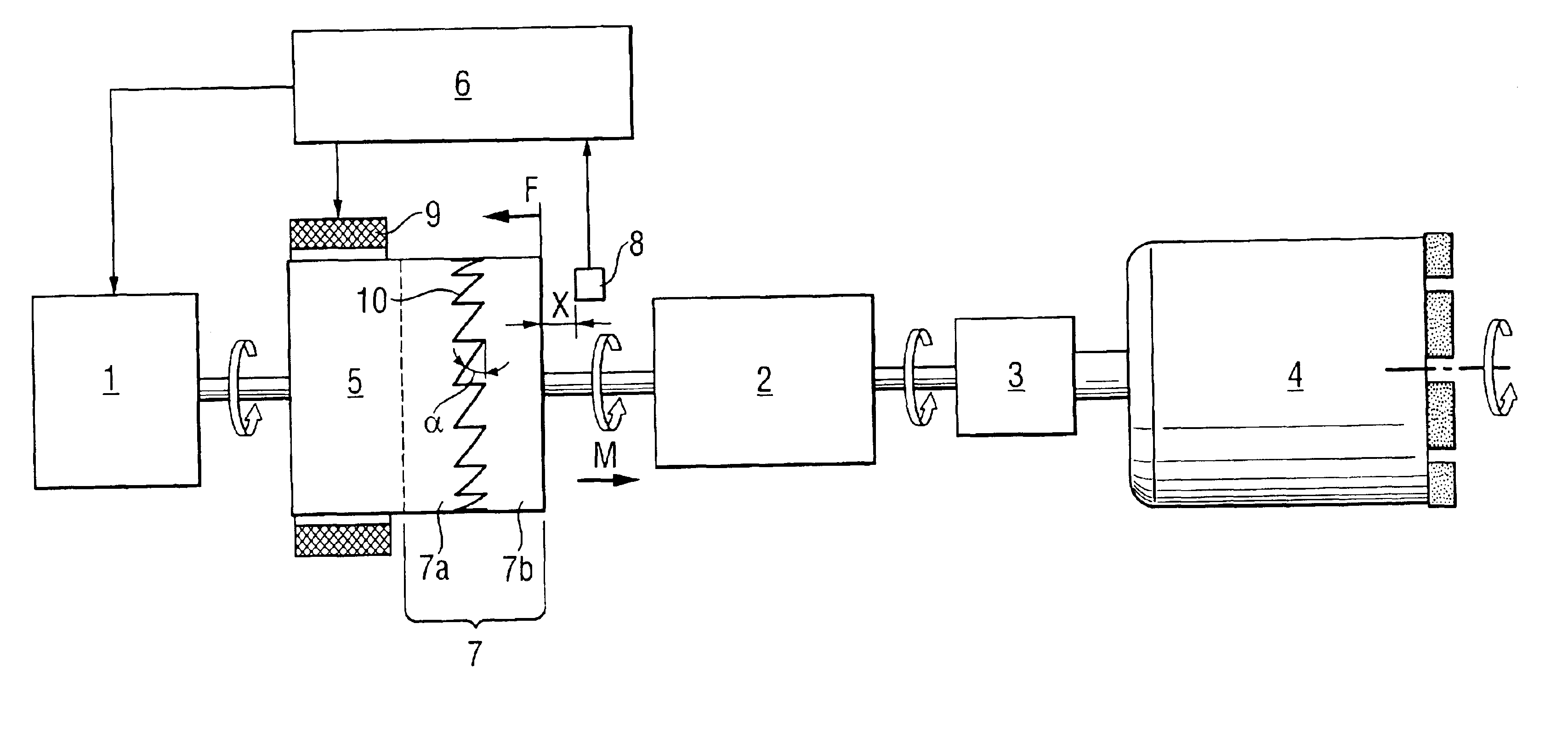
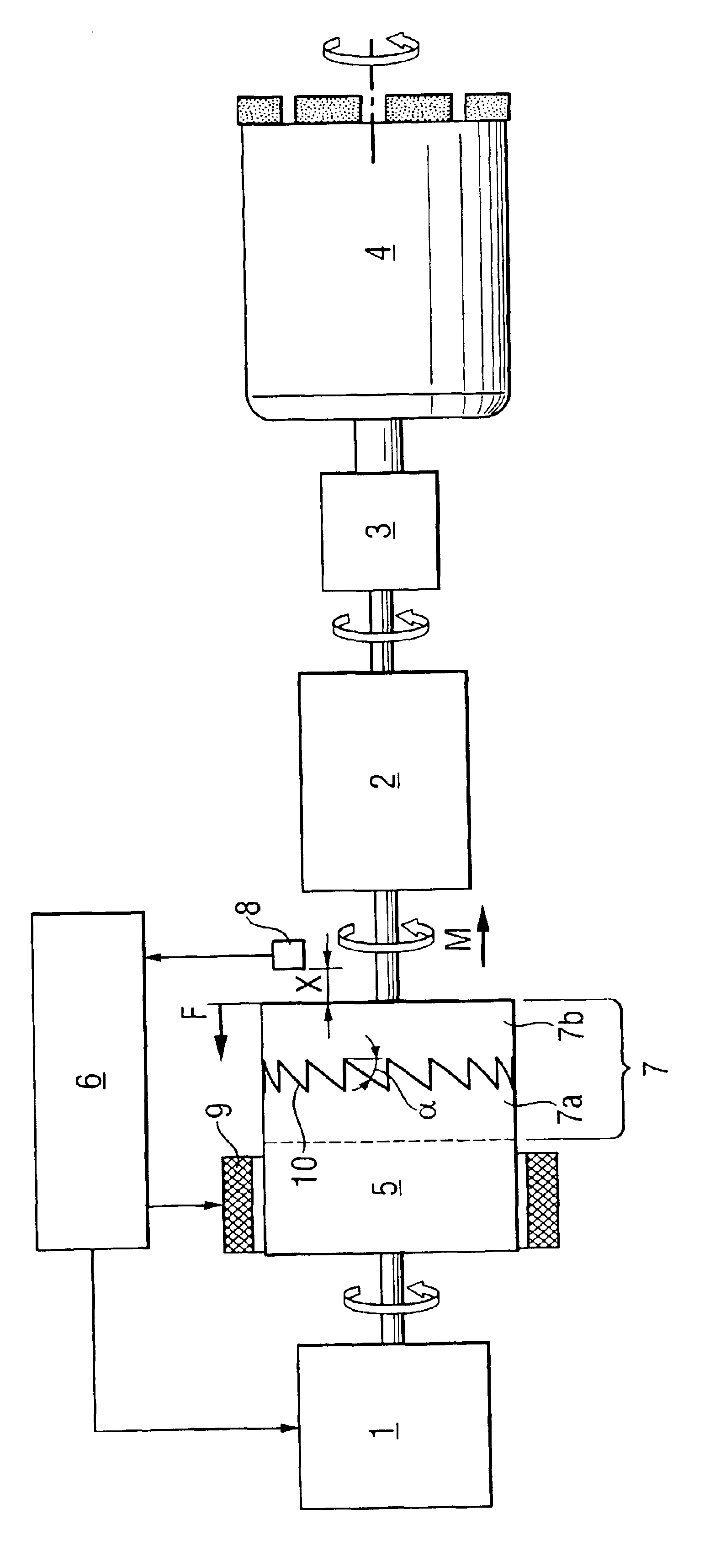
An overload protection arrangement for a portable power tool includes a magnetic clutch (5) arranged in a drive train of the power tool between an electromotor (1) and a tool chuck (3), control electronics (6) for controlling the magnetic clutch (5), a self-actuated torque-dependent overload clutch (7) likewise arranged in the drive train, and a sensor (8) for detecting an open condition of the overload clutch (7) upon the overload of the drive train and connected with the control electronics (6).
Owner:HILTI AG
Method for replacing insulator chains in 220 kV long-span high tower in live working
ActiveCN103227431AReliable engagementGuaranteed reliabilityApparatus for overhead lines/cablesArcing hornsEngineering
The invention relates to an operating tool for replacing insulator chains in live working, particularly to a method for replacing insulator chains in a 220 kV long-span high tower in live working. The key point is that the technology bias is overcome in a way that arcing horns and grading rings on a yoke plate for connecting the insulator chains are temporarily dismantled on a cross arm of the 220 kV long-span high tower to reserve screw holes for connecting fixtures on the yoke plate; the upper fixture and the lower fixture are connected with the yoke plate to lift the insulator chains, and the stress borne by the entire tool is half of that in the prior art, so that bearing and operation on the big-load insulator chains can be realized; and an equipotential electrician is borne by a chairlift to enter and go out of the electric field at the cross arm, so that the safety and controllability are stronger, the operating method is simple, the operating tool can be directly obtained from the existing tools, time and labor saving as well as economy are realized, and the replacement of the insulator chains on a steel pipe pole high tower comes true.
Owner:STATE GRID CORP OF CHINA +2
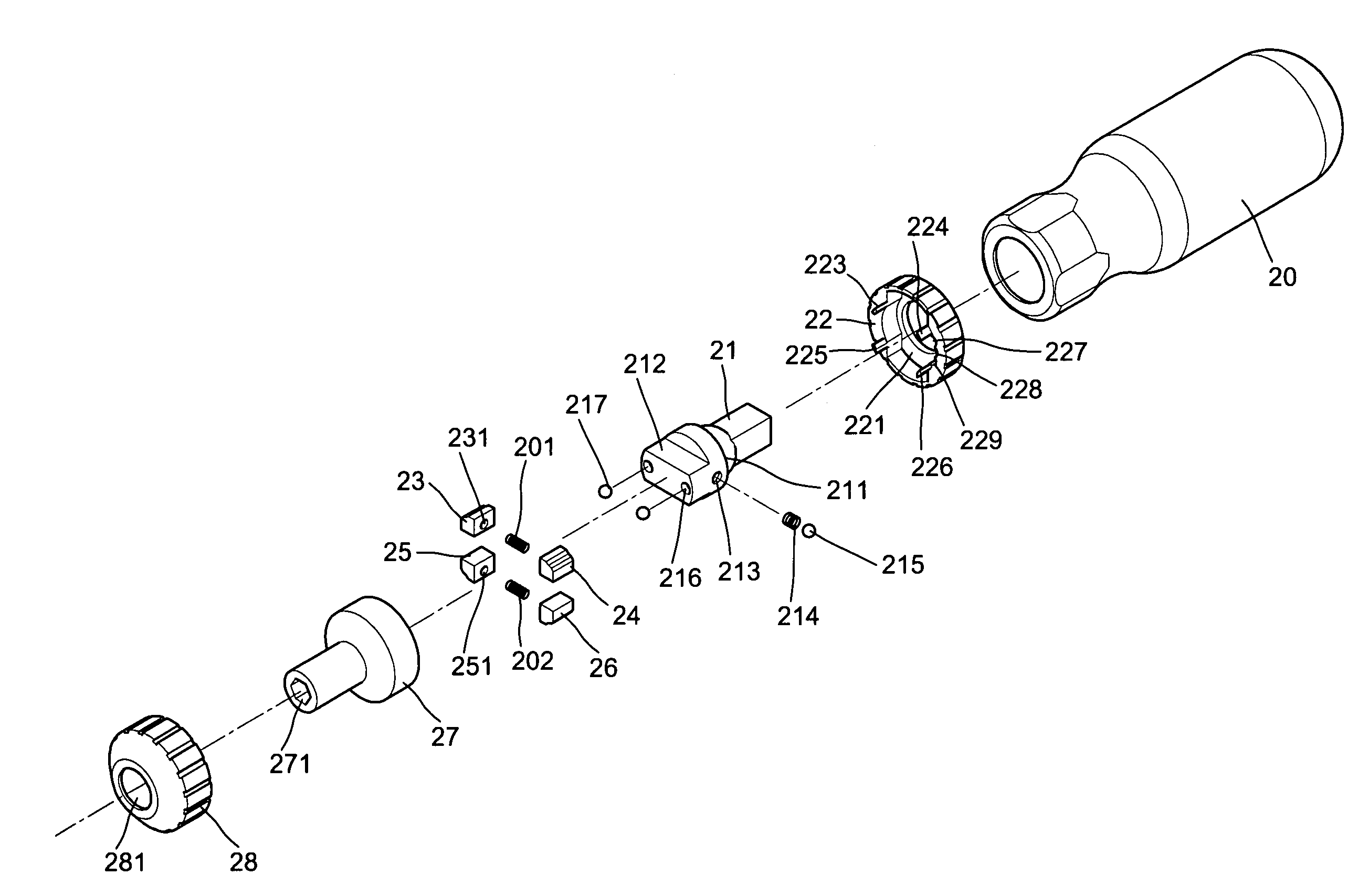
Flat belt made of elastomeric material
Owner:CONTITECH ANTRIEBSSYST
Recording medium drive including latch member for head actuator member
InactiveUS20070146937A1Reliable engagementReduce impactDisposition/mounting of recording headsRecord information storageEngineeringActuator
A recording medium drive allows a latch stop member to receive a latch member. The swinging movement around a rotation shaft causes the latch member to enter the movement path of a head actuator member. The latch member thus engages with the head actuator member. The latch member has the portion in contact with the latch stop member. The portion is made of a material different from the main portion of the latch member. The repulsive coefficient can be adjusted between the portion and the latch stop member. An identical condition can be established for transmission of an impact between the head actuator member and the actuator stop member as well as between the latch member and the latch stop member. Even if impacts of different duration act on the recording medium drive, the latch member is allowed to reliably engage with the head actuator member.
Owner:FUJITSU LTD
Electrical connector with improved contact
ActiveUS7686628B2Increase production costImproved connectorLine/current collector detailsTwo-part coupling devicesSolderingElectrical connector
Owner:HON HAI PRECISION IND CO LTD
Connector capable of preventing incomplete fitting
ActiveUS6918783B2Reliable engagementReliable conductionElectrically conductive connectionsIncorrect coupling preventionEngineeringMechanical engineering
A first connector housing is provided with a flexible lock arm. A tubular fitting detector is fitted to an outer periphery of the first connector housing while being slidable thereon between a first position and a second position. A retainer is provided on the fitting detector, and adapted to be engaged with a first portion of the lock arm in a case where the fitting detector is placed at the first position, and engaged with a second portion of the lock arm in a case where the fitting detector is placed at the second position. A second connector housing is provided with a projection adapted to release the engagement between the retainer and the first portion of the lock arm in a case where the second connector housing is completely fitted with the first connector housing, so that the fitting detector is allowed to slide from the first position to the second position. A finger pad is provided on the fitting detector, and arranged such that an operator's finger is placeable thereon while being separated from at least the lock arm, during a fitting operation between the first connector housing and the second connector housing.
Owner:YAZAKI CORP
Pre-fastened absorbent article having simplified fastening features
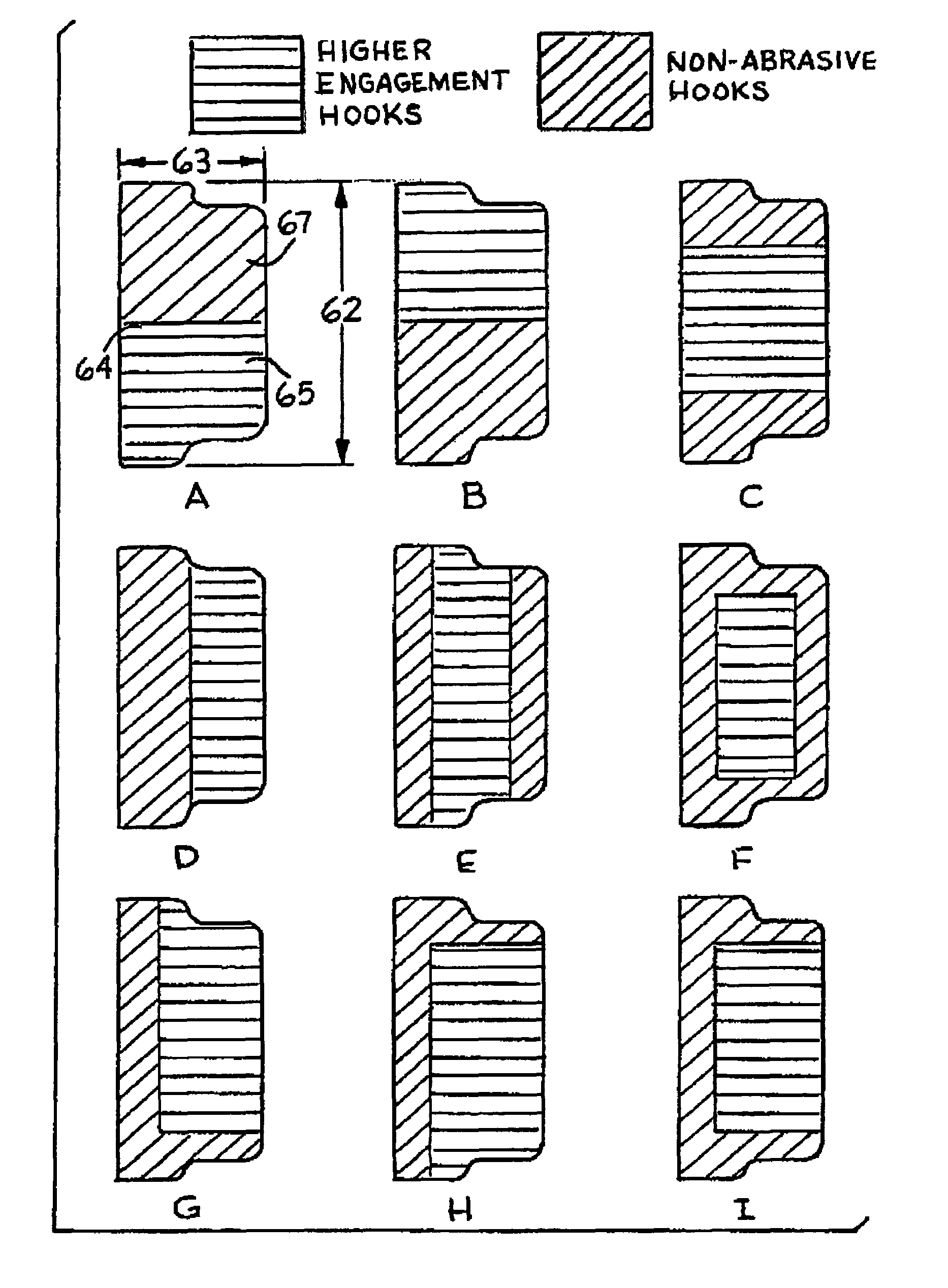
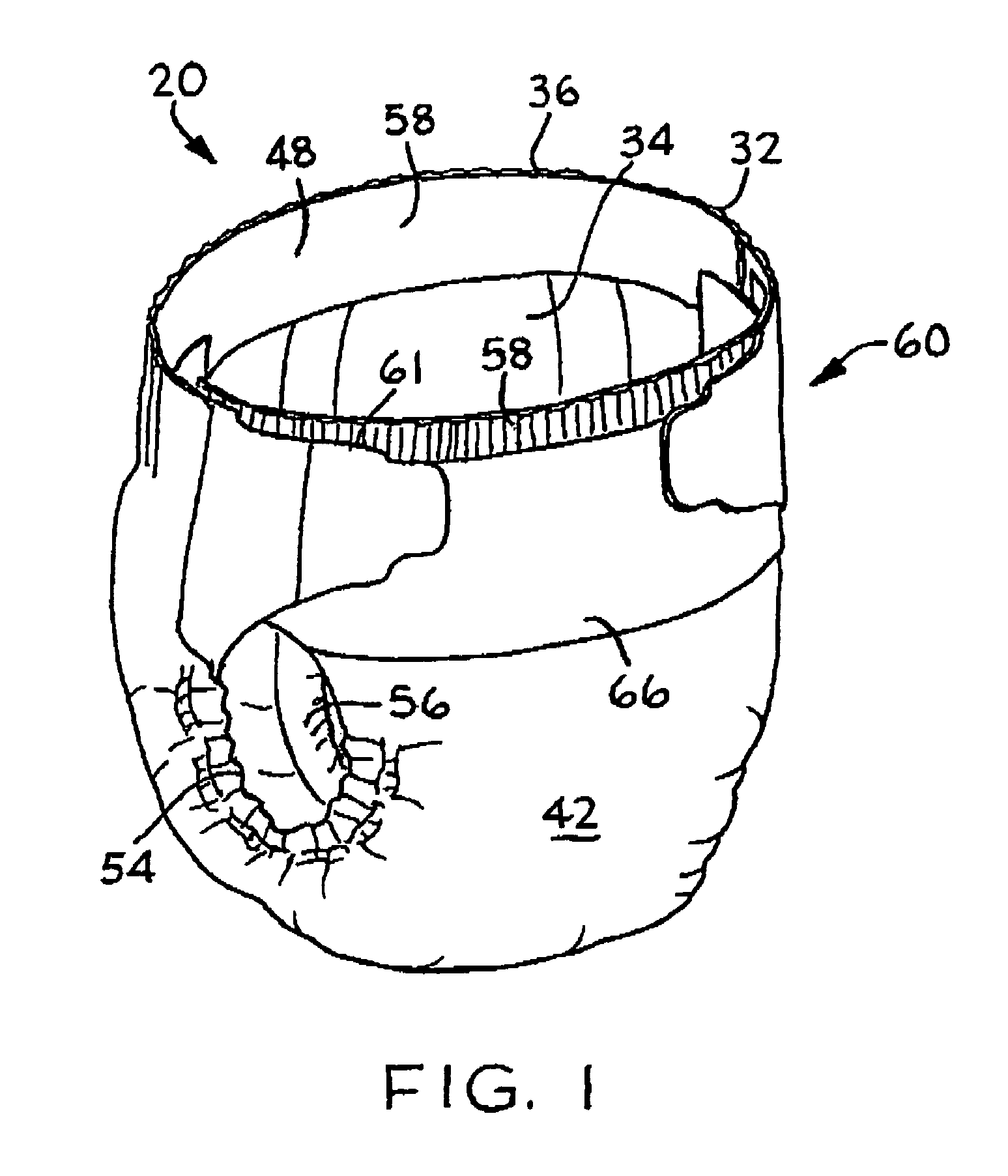
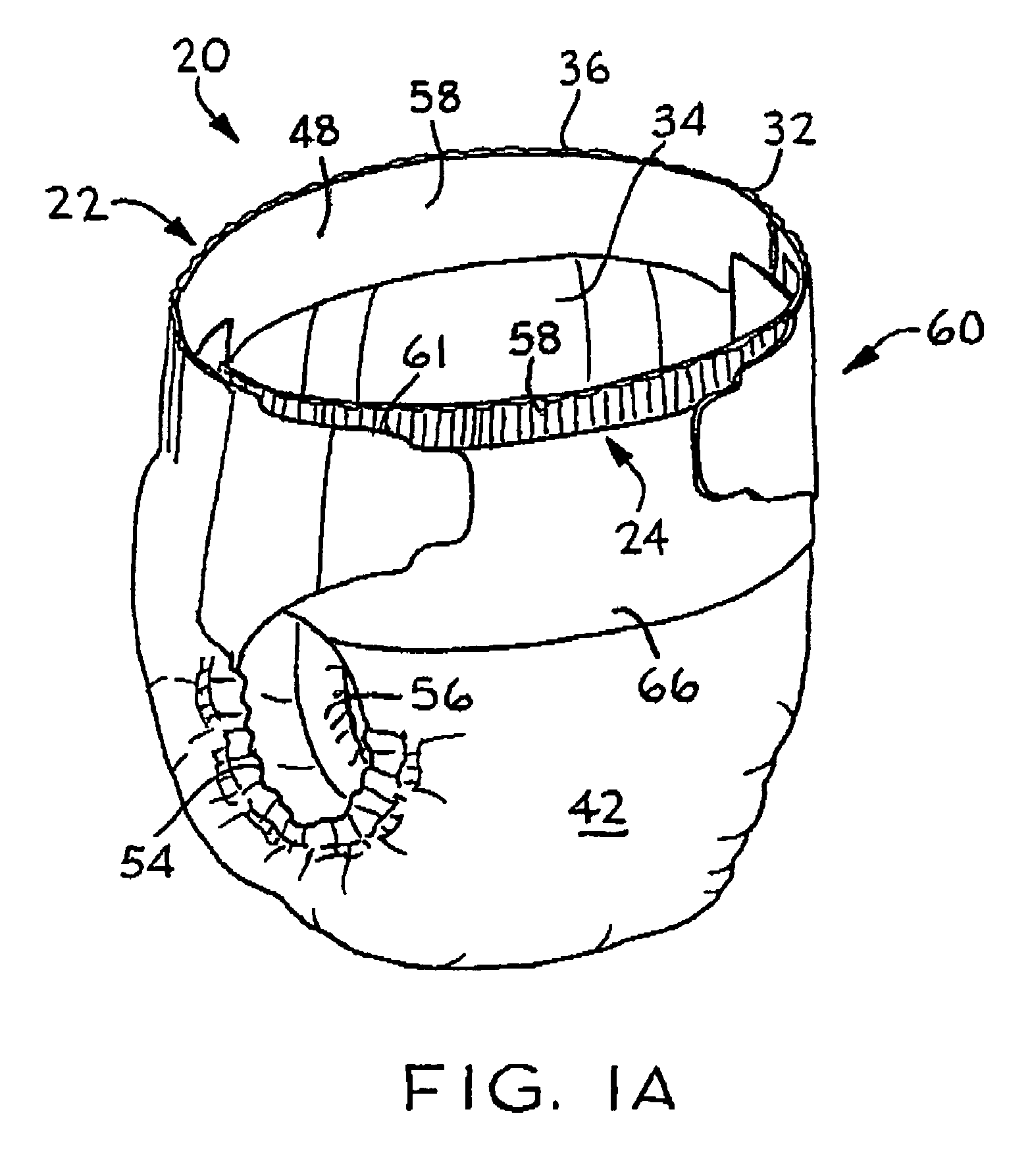
Prefastened absorbent articles (20) may include a singular fastening mechanism that includes an oversized fastener (60), to provide stability between the front waist region and the back waist region and to maintain the prefastened configuration. The prefastened absorbent articles can include a multiple property fastener (60) that has at least one engagement zone (65) and at least one non-abrasive zone (67).
Owner:KIMBERLY-CLARK WORLDWIDE INC
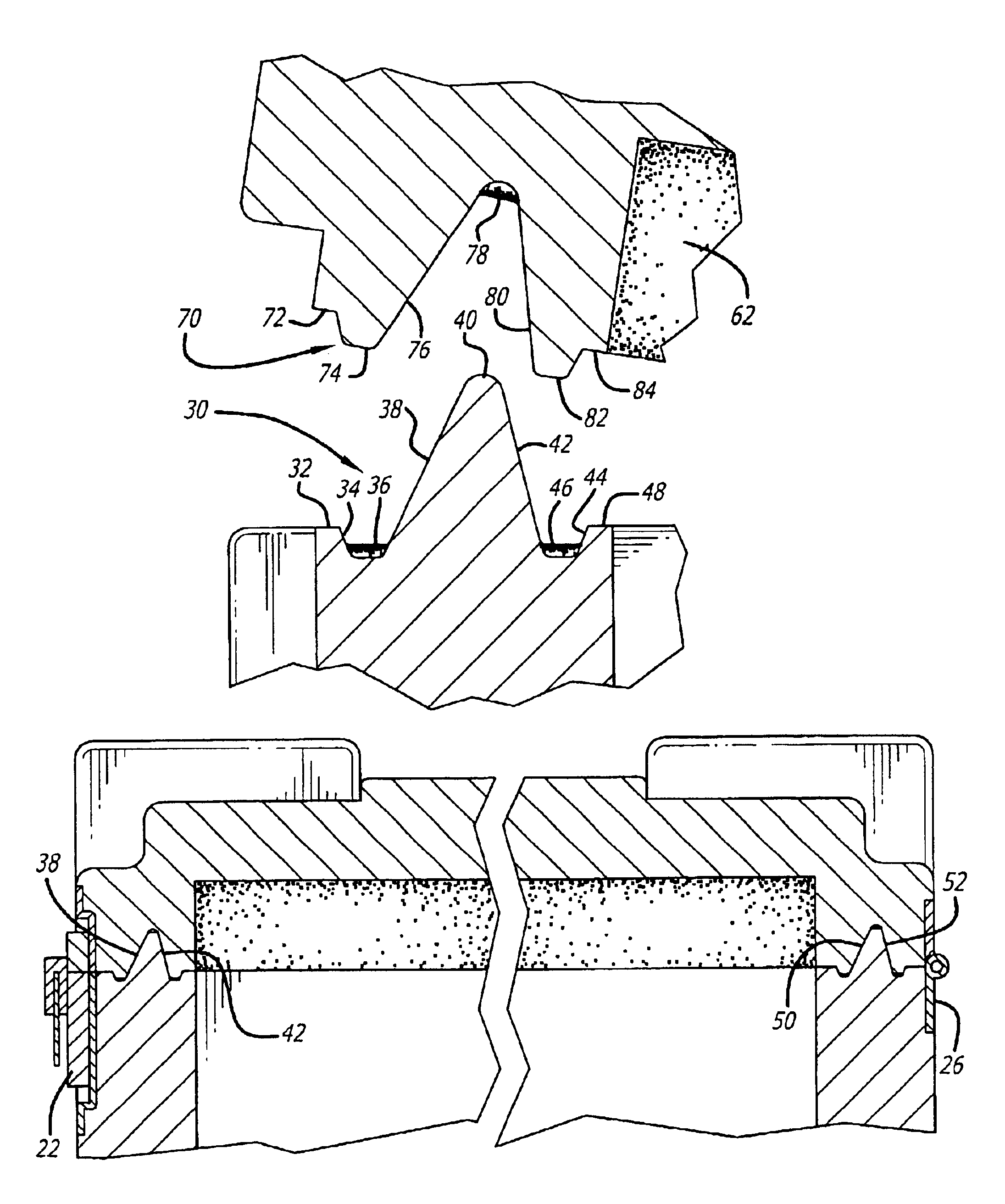
RF connector assembly
InactiveUS7789667B2Reliable engagementEffectively provides a controllable twisting forceTwo pole connectionsCouplings bases/casesInterior spaceElectrical conductor
An RF connector assembly (100) assembly has a first connector and a second connector (3) electrically connecting with the first connector. The first connector includes a first main body (11) defining an interior space (110), an insulative member (12) received in the interior space, a center conductor (13) retained within the insulative member, a flexible bushing (14) and a magnetic member (15) retained in the interior space. The second connector includes a mating member (35) firmly resisting against the flexible bushing under an attractive force exerted by the magnetic member.
Owner:HON HAI PRECISION IND CO LTD
System for restarting internal combustion engine when engine restart condition is met
ActiveUS8671903B2Smoothly engagedReduce noiseAnalogue computers for vehiclesDigital data processing detailsStart timeGear wheel
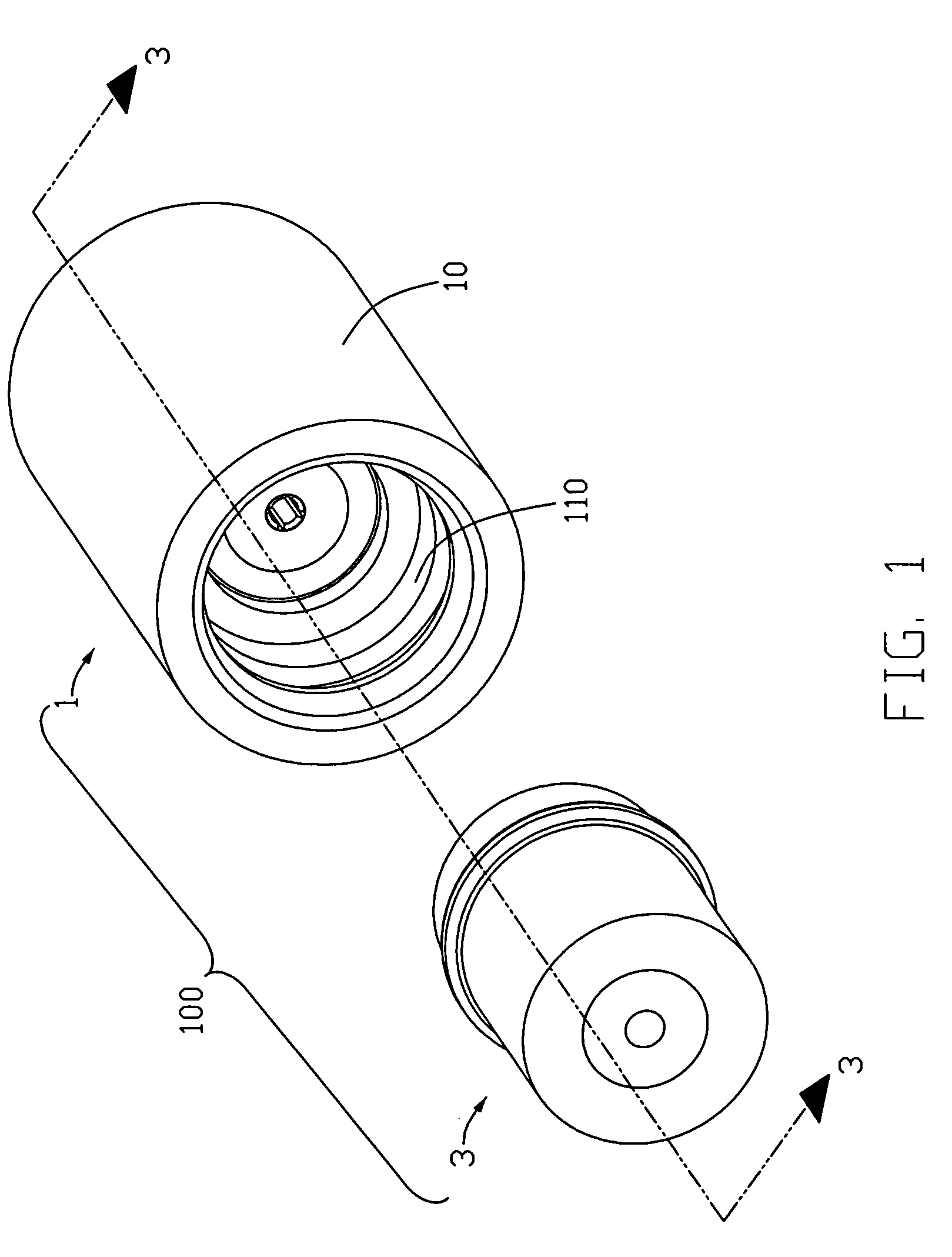
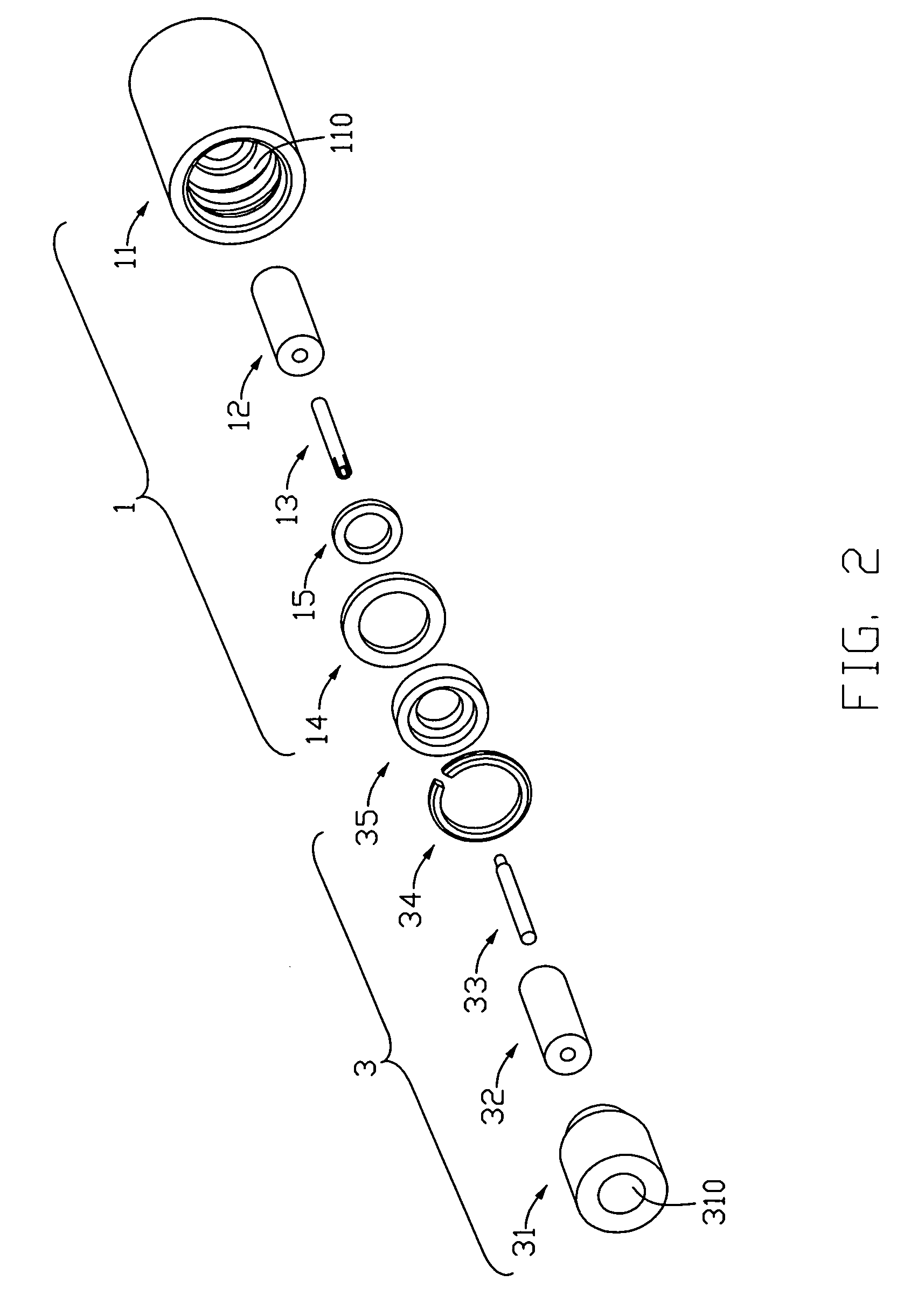
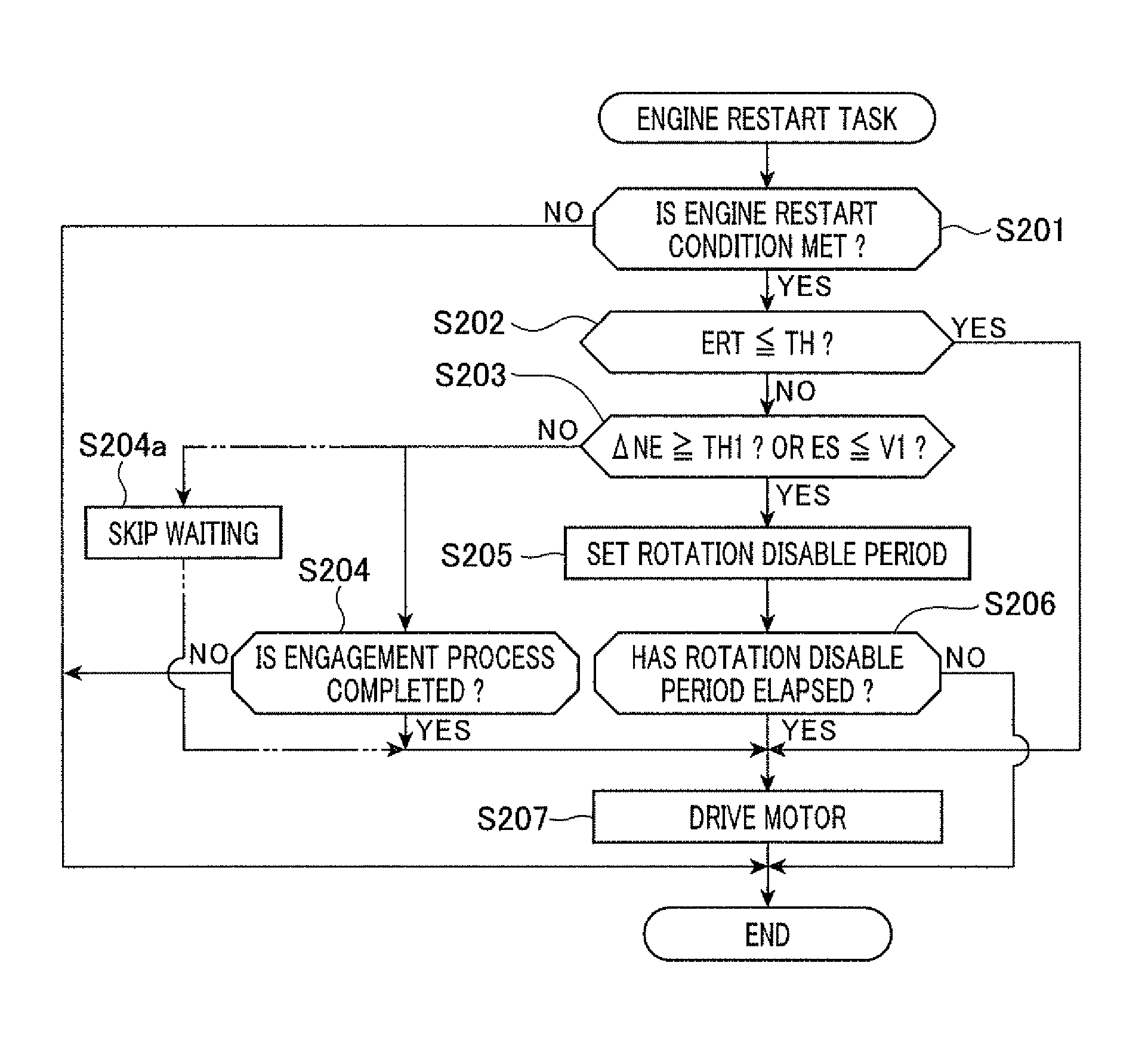
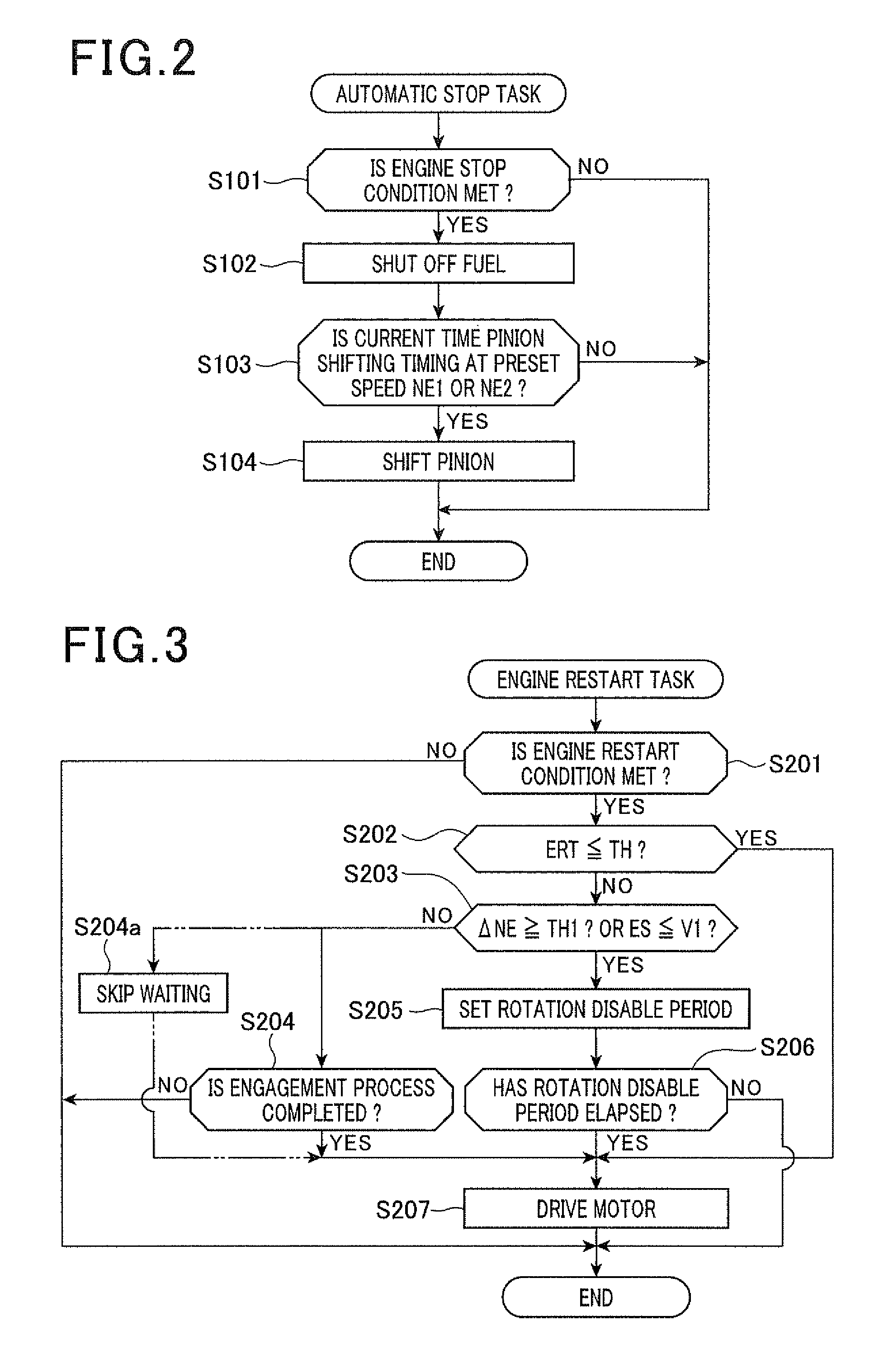
In a system, a pinion shift unit starts shift of a pinion to a ring gear for engagement therebetween during an internal combustion engine coasting in a forward direction after an automatic stop of the internal combustion engine. An engagement determining unit determines whether the pinion and the ring gear have any one of first and second positional relationships therebetween. The first positional relationship represents that the pinion is at least partly engaged with the ring gear. The second positional relationship represents that the pinion is in abutment with the ring gear. When an engine restart condition is met before it is determined that the pinion and the ring gear have any one of first and second positional relationships therebetween after the start of the shift of the pinion to the ring gear, a rotation adjusting unit adjusts a start timing of rotation of the pinion.
Owner:DENSO CORP
Buckle device
InactiveUS6922875B2Stand steadyLimit its operationSnap fastenersClothes buttonsEngineeringTongue part
A buckle apparatus includes an insertion control subassembly press-operating an ejector when a pair of tongue parts are simultaneously inserted into a buckle body for allowing insertion up to an engaging position between the respective tongue parts and an engaging member while regulating the press operation by posture tilting of the ejector when only one tongue part is inserted. The insertion control subassembly includes a frame member having a guide rail part reciprocatively slidingly guiding the ejector along the direction of the press operation while including a stopper part separably coming into contact with the ejector in posture tilting from an insertion allowing posture for regulating movement in the direction of the press operation on the guide rail part.
Owner:ASHIMORI INDS
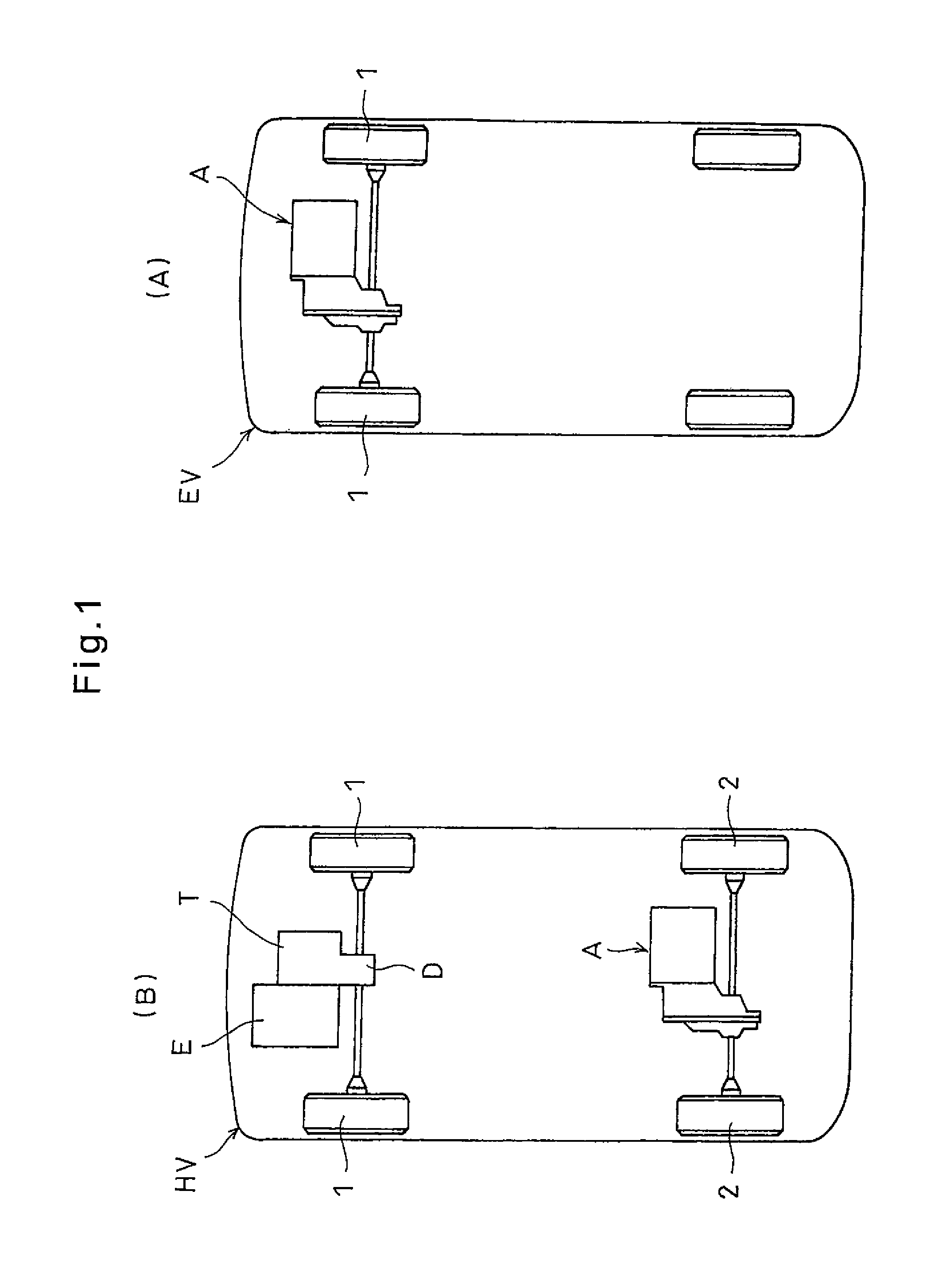
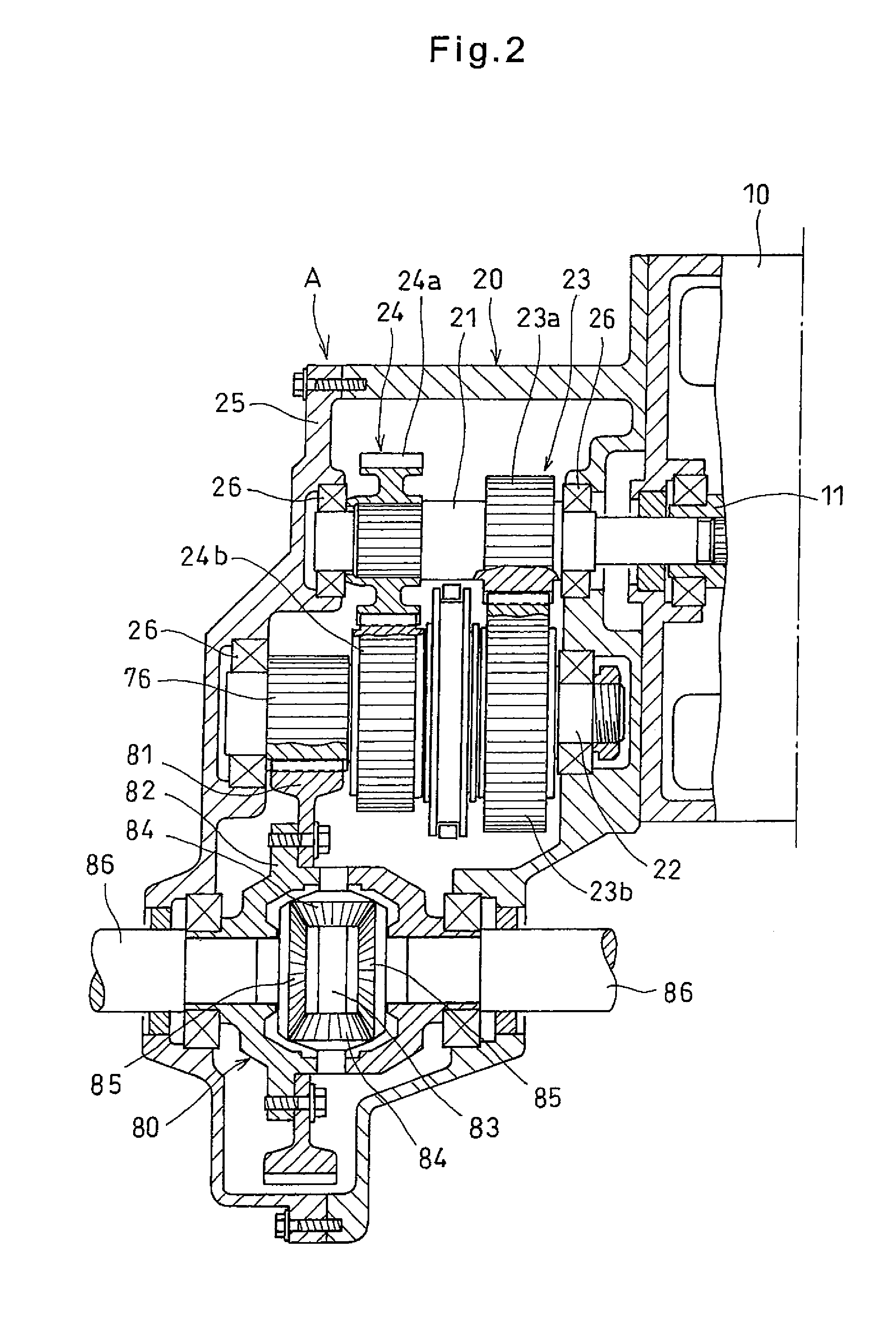
Motor drive apparatus for vehicle and motor vehicle
ActiveUS20120158233A1Reduce energy lossPrevent rotationDigital data processing detailsGearing controlMotor driveEngineering
A motor drive apparatus for a vehicle is provided which can prevent its transmission and motor from being rotated by vehicle wheels, and which allows quick gear change. This apparatus includes an electric motor (10), a first shaft (21) driven by the electric motor (10), a second shaft (22), first and second reduction gear trains (23 and 24) disposed between the first and second shafts (21 and 22). Two-way roller clutches (30A and 30B) are mounted between a first output gear (23b) of the first reduction gear train (23) and the second shaft (22) and between a second output gear (24b) of the second reduction gear train (24) and the second shaft (22), respectively. Gear changes are made by selectively engaging and disengaging the two-way roller clutches (30A and 30B) with a speed changing mechanism (50).
Owner:NTN CORP
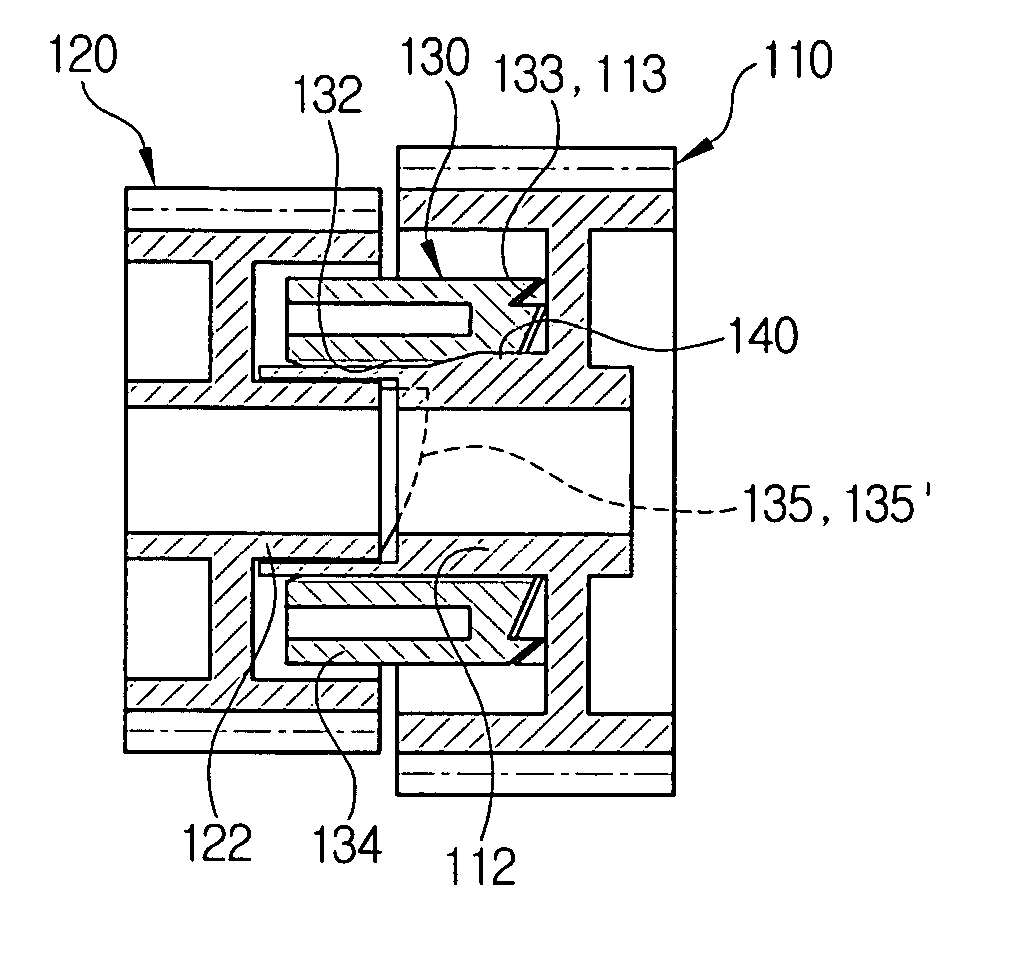
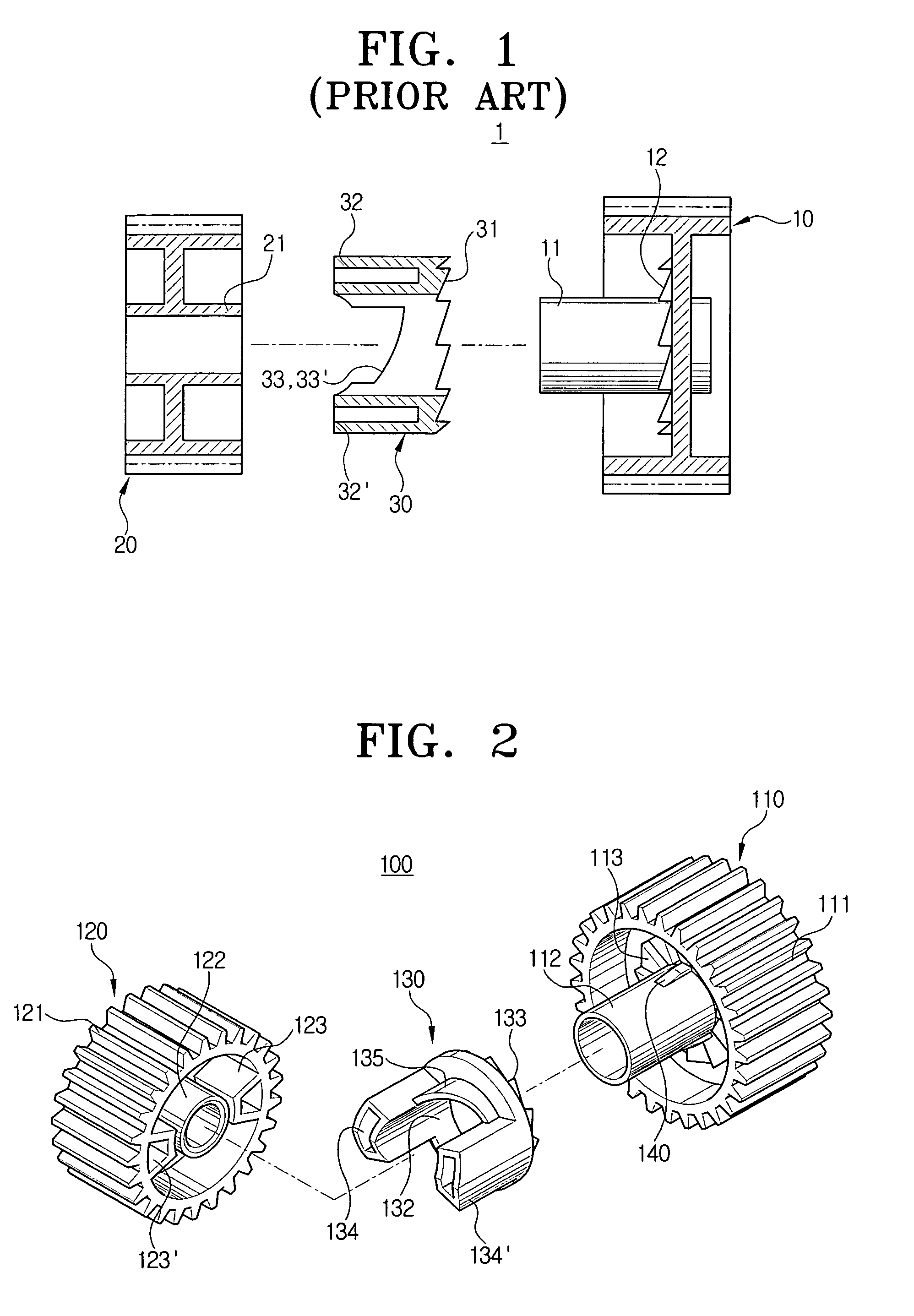
One-way power transmission unit, a fusing unit driving apparatus for duplex printer using the same, a method for one way power transmission, and a method for driving a fusing unit
ActiveUS20050241902A1Reliable performanceImprove performanceMultiplex system selection arrangementsElectrically conductive connectionsElectric power transmissionEngineering
A one-way power transmission unit has force-fitting means formed on at least one of an outer circumference of a boss of a driving member and an inner circumference of a boss hole of a latch member, for force-fitting the driving member into the latch member with a friction which is less than a load exerted to a driven member. A method of one-way power transmission including, inter alia, force-fitting the driving member into the latch member with a friction which is less than a load exerted to a driven member. Since the latch member force-fits over the boss of the driving member, the driving member and the latch member rotate together with each other when the driving member switches from a driving force disconnection state to a driving force connection state, and thus, engagement of latch gears of the driving member and the latch member is reliably achieved. Accordingly, the time interval between switching from the driving force disconnection state to the connection state can be maintained to be essentially constant.
Owner:HEWLETT PACKARD DEV CO LP
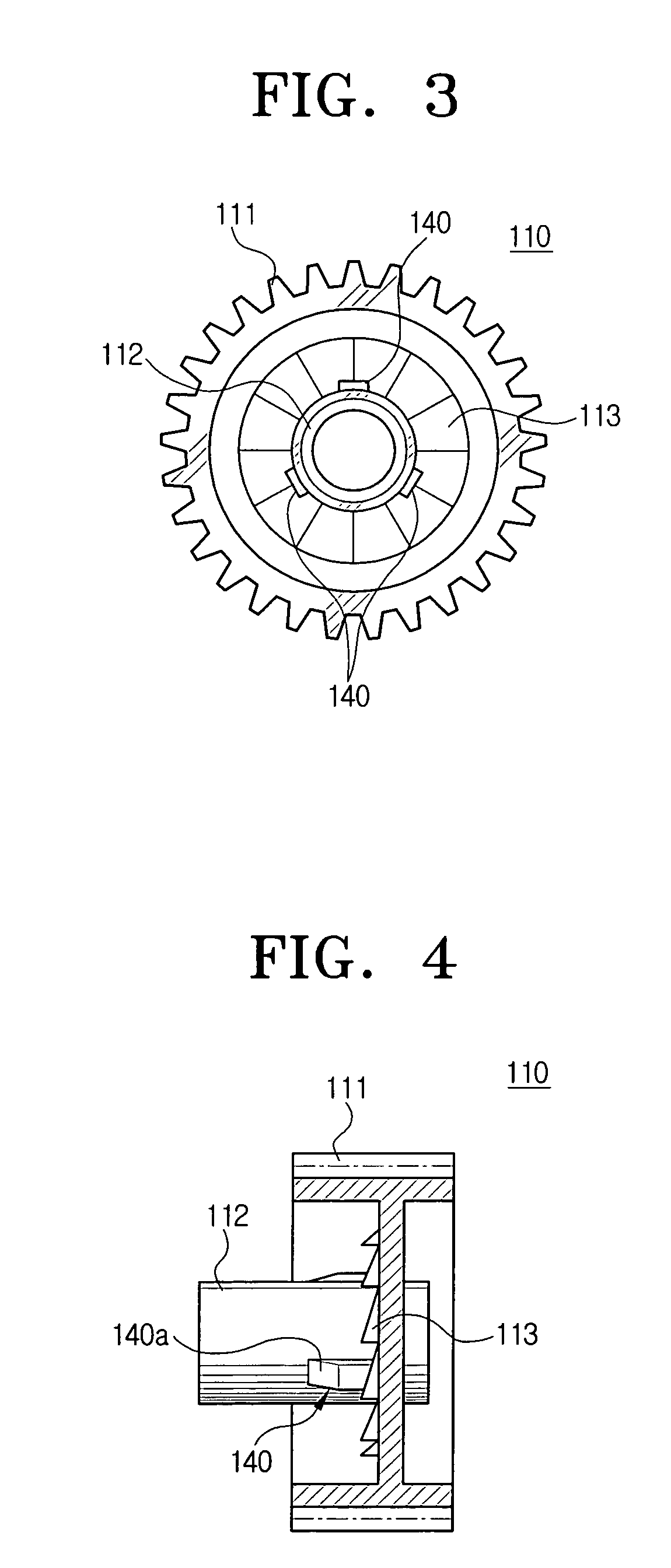
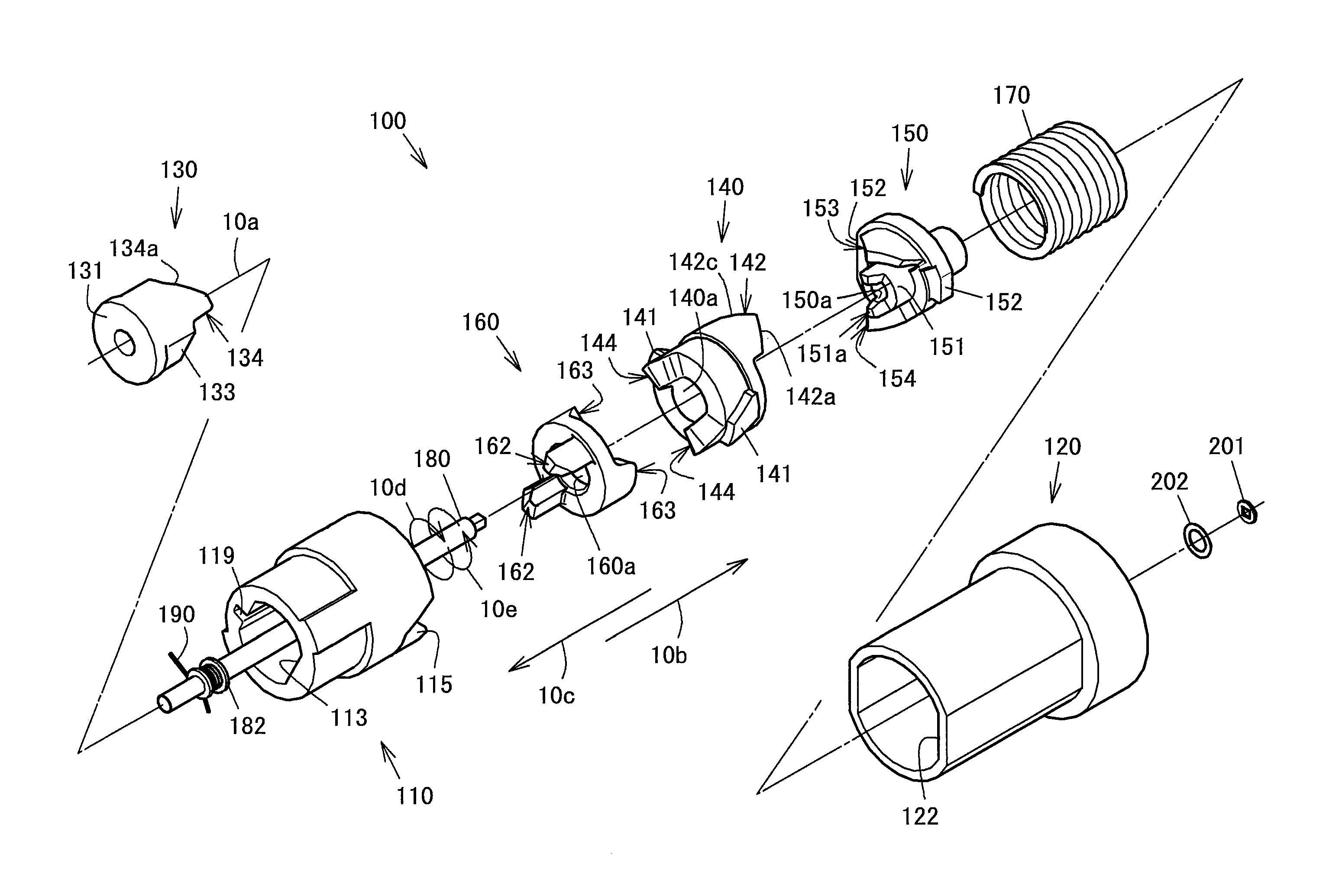
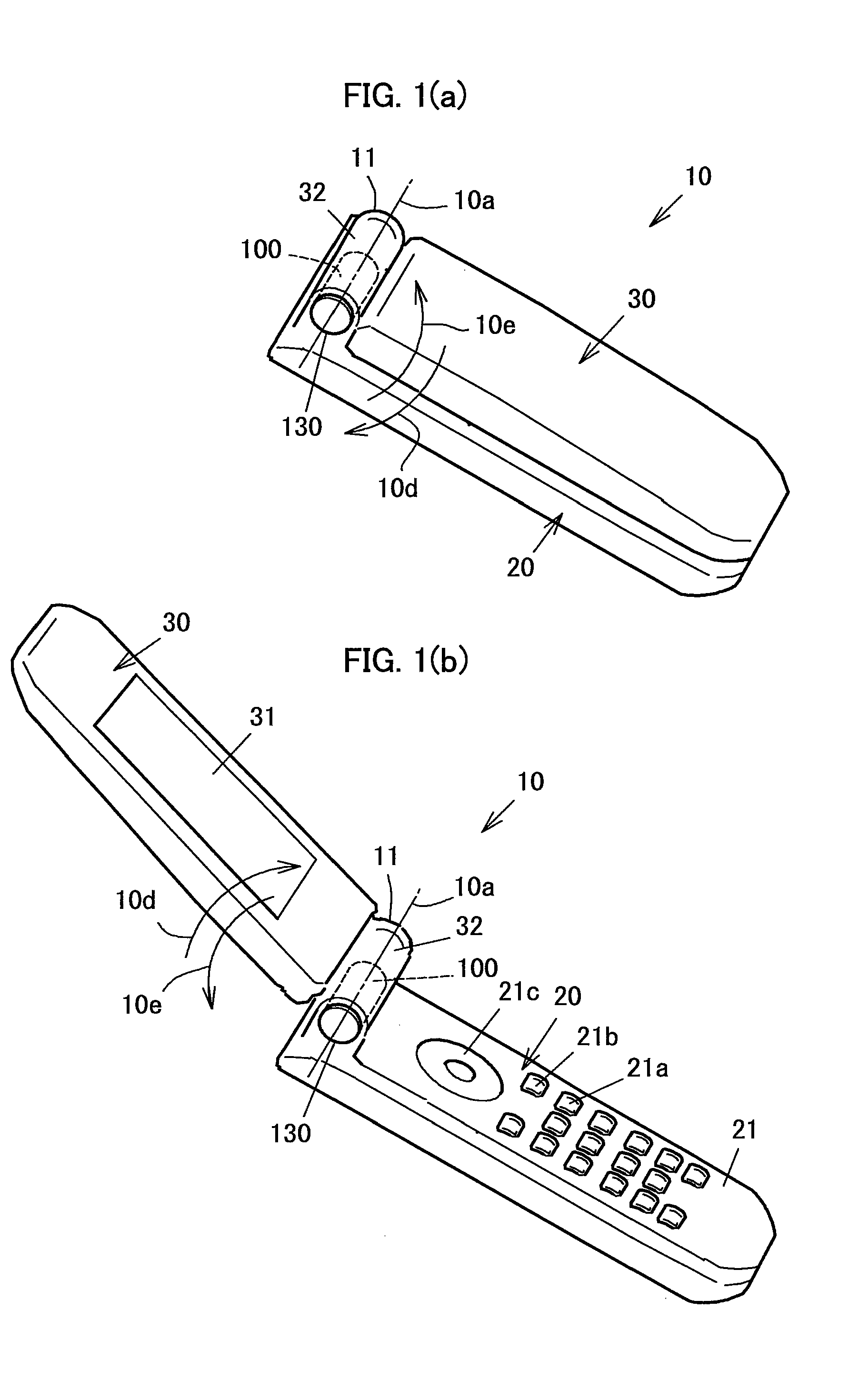
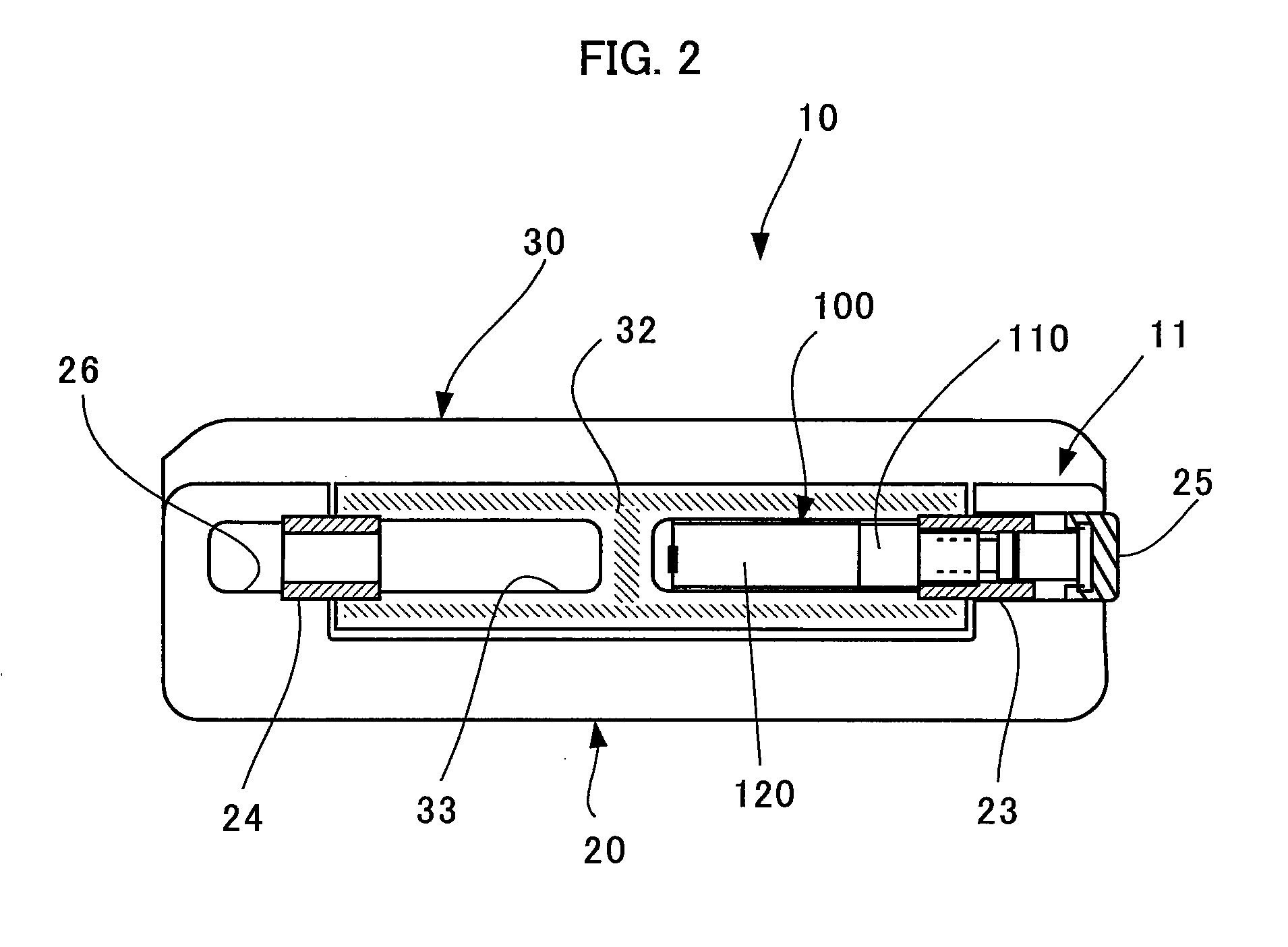
Opening/Closing Device and Mobile Device Using the Same
InactiveUS20100024165A1Smooth transferring operationReliable engagementSubstation equipmentHingesSurface pressureEngineering
It is an object of the present invention to provide an opening / closing device which can be produced simple in construction and reduce the surface pressure of its cam portions, and a mobile phone which can be reduced in cost and sufficiently reliable in the hinged members by using the opening / closing device. In the opening / closing device operable to rotate the second member (30) with respect to the first member (20) hingedly connected the second member (30) in an opening operation direction by operating first and second rotation cams (160, 140), and a movable cam (150) held in engagement with the second rotation cam (140) in response to the displacement of the operation cam (130), the second rotation cam (140) and the movable cam (150) have a plurality of kinds of end cam portions ((142, 153), (143, 154)) formed thereon at different radiuses (r1, r2) from the guide center axis (110c) in opposing relationship with each other, the urging direction of the urging means (170) to the second member (30) is changed by the end cam portions ((142, 153), (143, 154)) when the operation cam (130) is operated to cause the first rotation cam (160) to be rotated from the predetermined operation starting position.
Owner:PANASONIC CORP
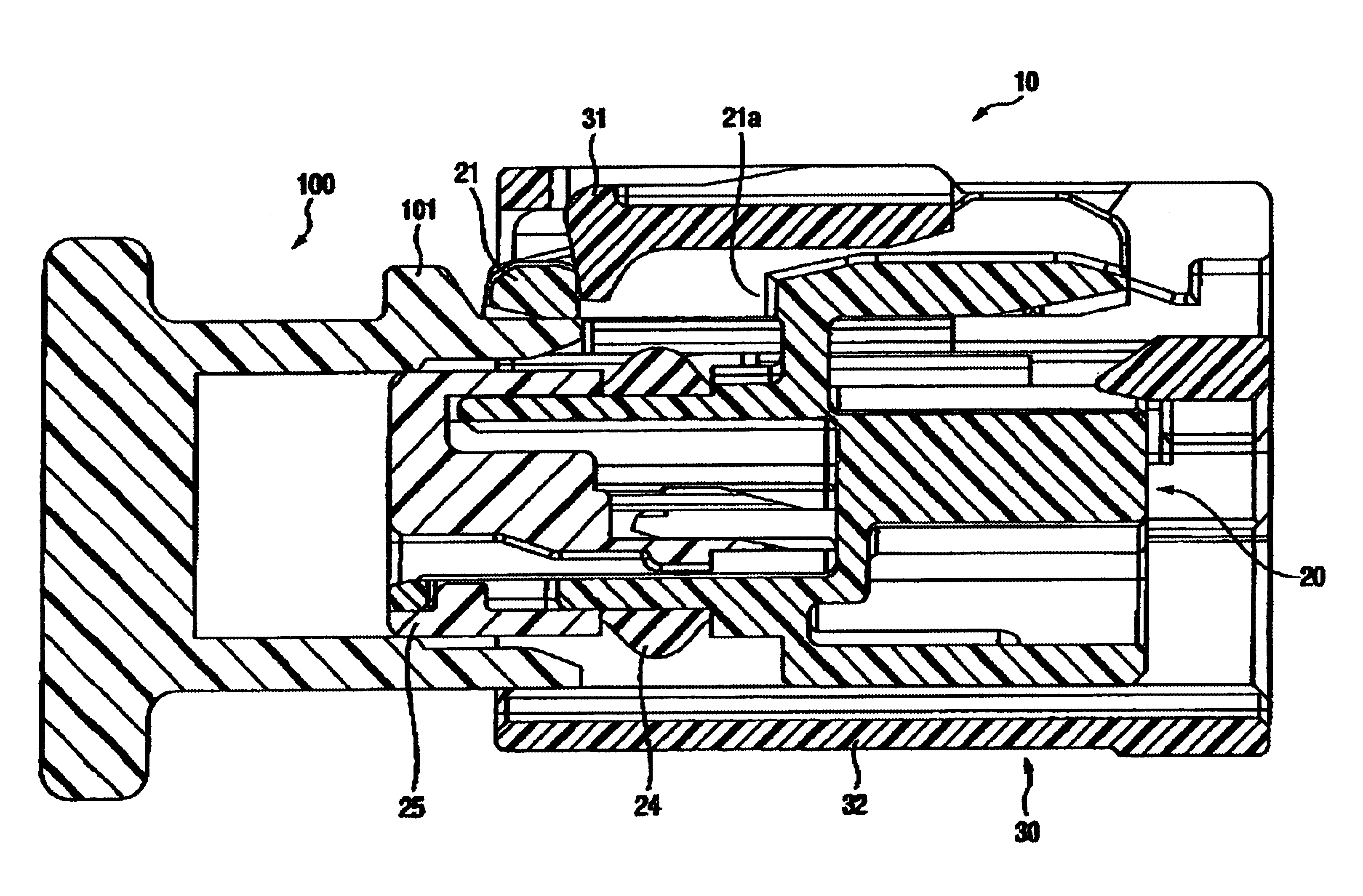
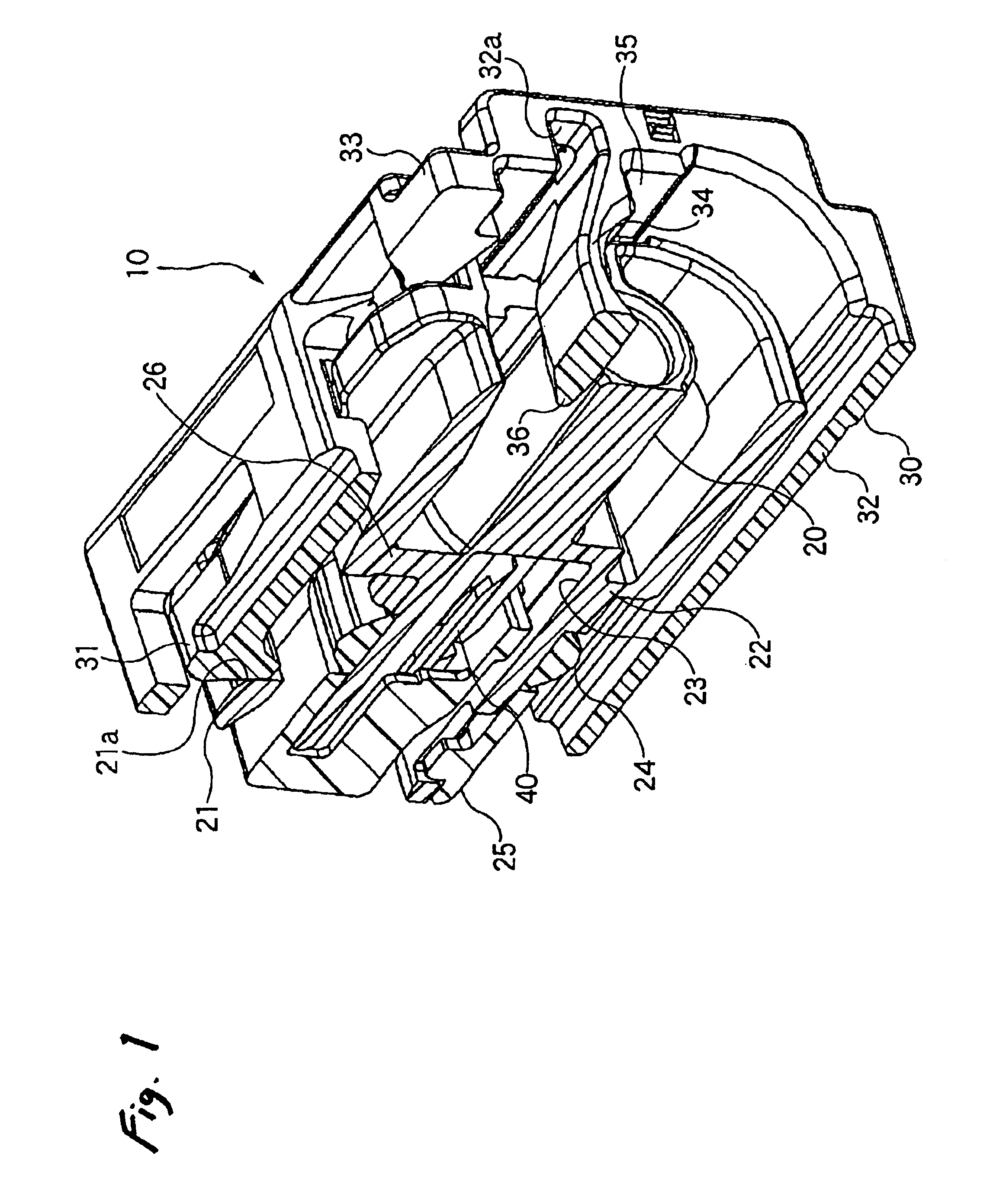
Contact for electrical connector
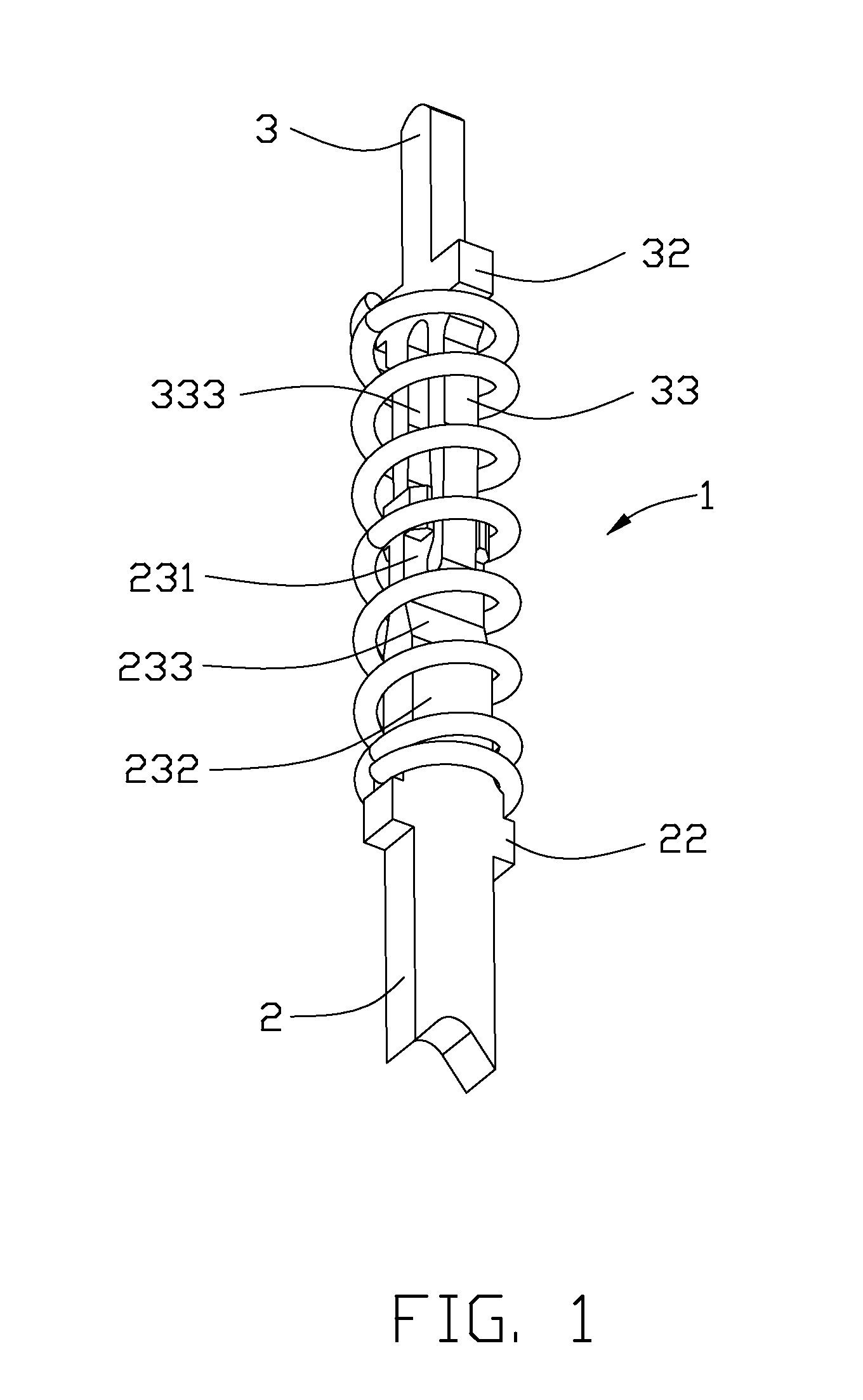
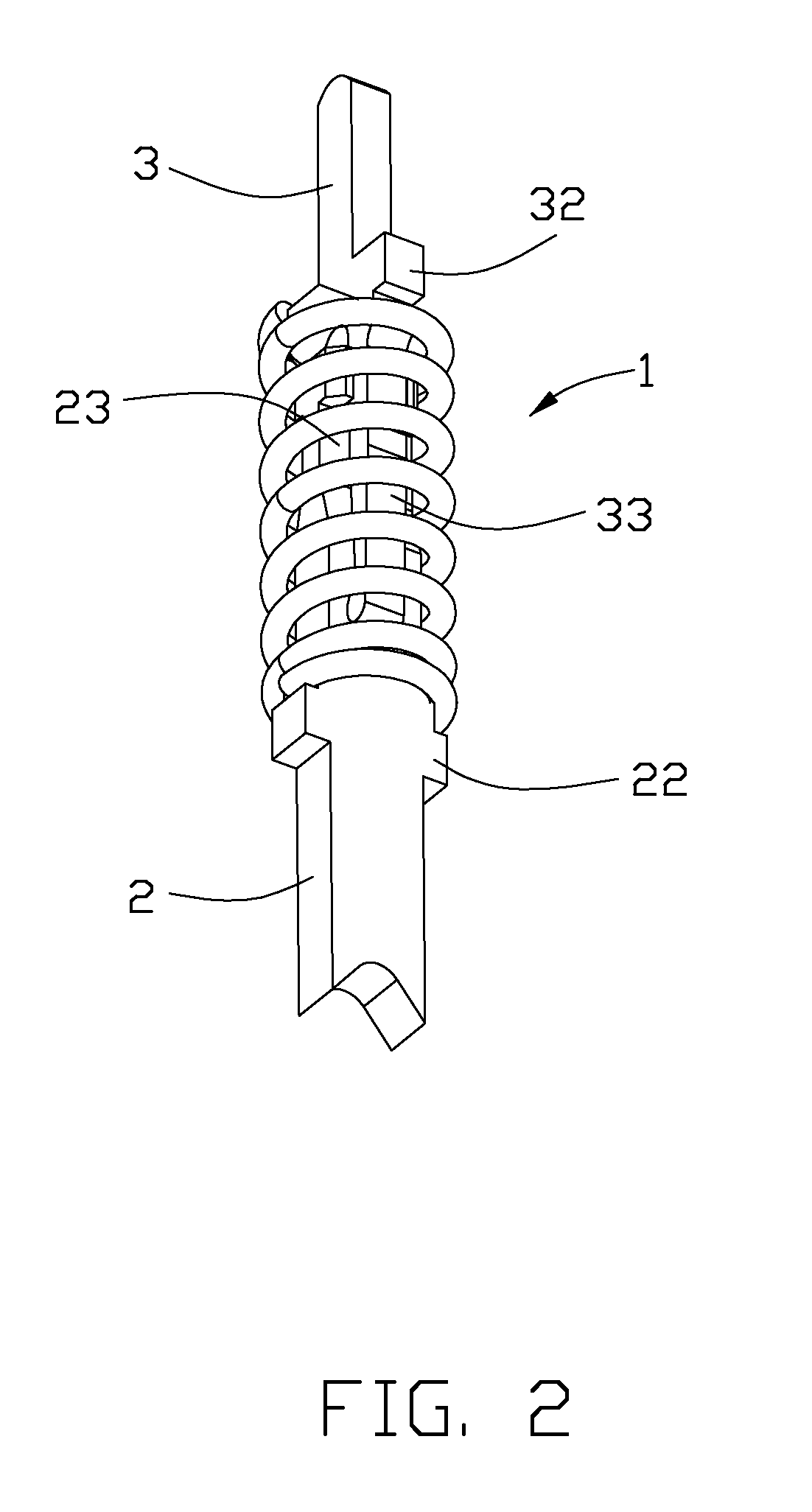
ActiveUS8157601B2Simple configurationReliable engagementCoupling contact membersClamped/spring connectionsElectrical connectorBiomedical engineering
A contact (1) includes a first contact pin (2), a second contact pin (3) and a spring (4). The first contact pin includes a mating end (26), a body (23) having a first body portion (232) and a second body portion (231), a pair of ear portions (24) and a pair of first blocking portions (22). The first body portion has a thickness greater than that of the second body portion. The second contact pin includes a pair of second blocking portions (32), and a pair of resilient beams (33) slidable between a latched position and an unlatched position. The resilient beams are confined by the ear portions. The resilient beams clasp the first body portion in the latched position. The spring is compressible between the pair of first blocking portions and the pair of second blocking portions.
Owner:HON HAI PRECISION IND CO LTD
Land grid array connector assembly with reliable block
InactiveUS6929495B2Reliable engagementEngagement/disengagement of coupling partsComponent plug-in assemblagesElectrical connectionLand grid array
An LGA connector assembly (1) includes an insulative housing (10), a number of terminals (12) received in the housing, and a metal clip (40) mounted on the housing. The clip is disposed on the housing to press an LGA chip upon the terminals and has two opposite slant sides (42). The housing includes a first sidewall and a second sidewall opposite to the first sidewall. A pair of arcuately spaced protrusions is extended from an outside of the second sidewall. A pair of trapeziform-configuration blocks is formed on an edge of the corresponding protrusions. When the clip presses the LGA chip onto the terminals, the blocks can prevent the clip driving the LGA chip move upwardly relative to the housing, thereby ensuring reliable electrical connection between the LGA chip and the connector assembly.
Owner:HON HAI PRECISION IND CO LTD
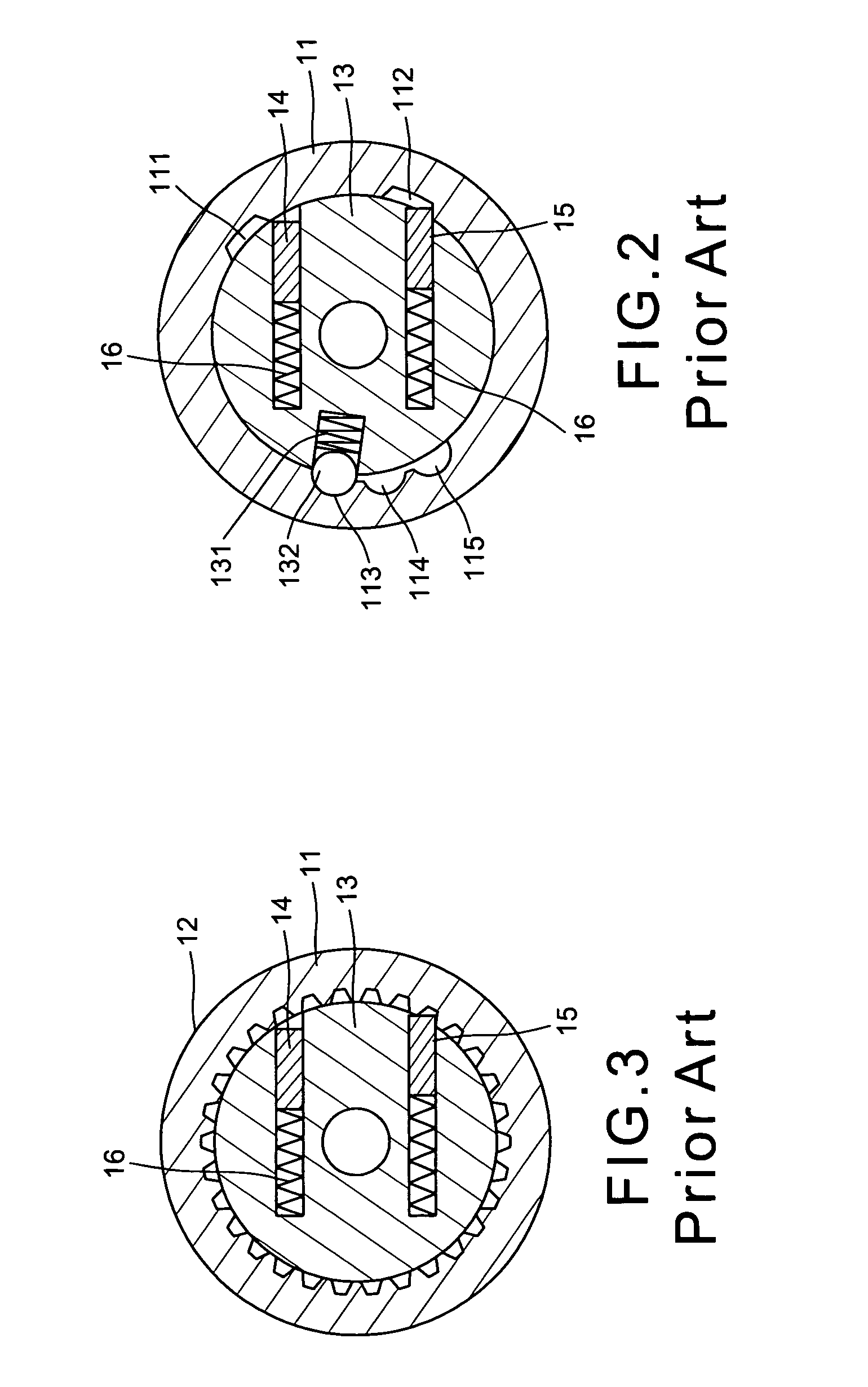
Toothed wheel gearing (variants) and a planetary toothed mechanism based thereon (variants)
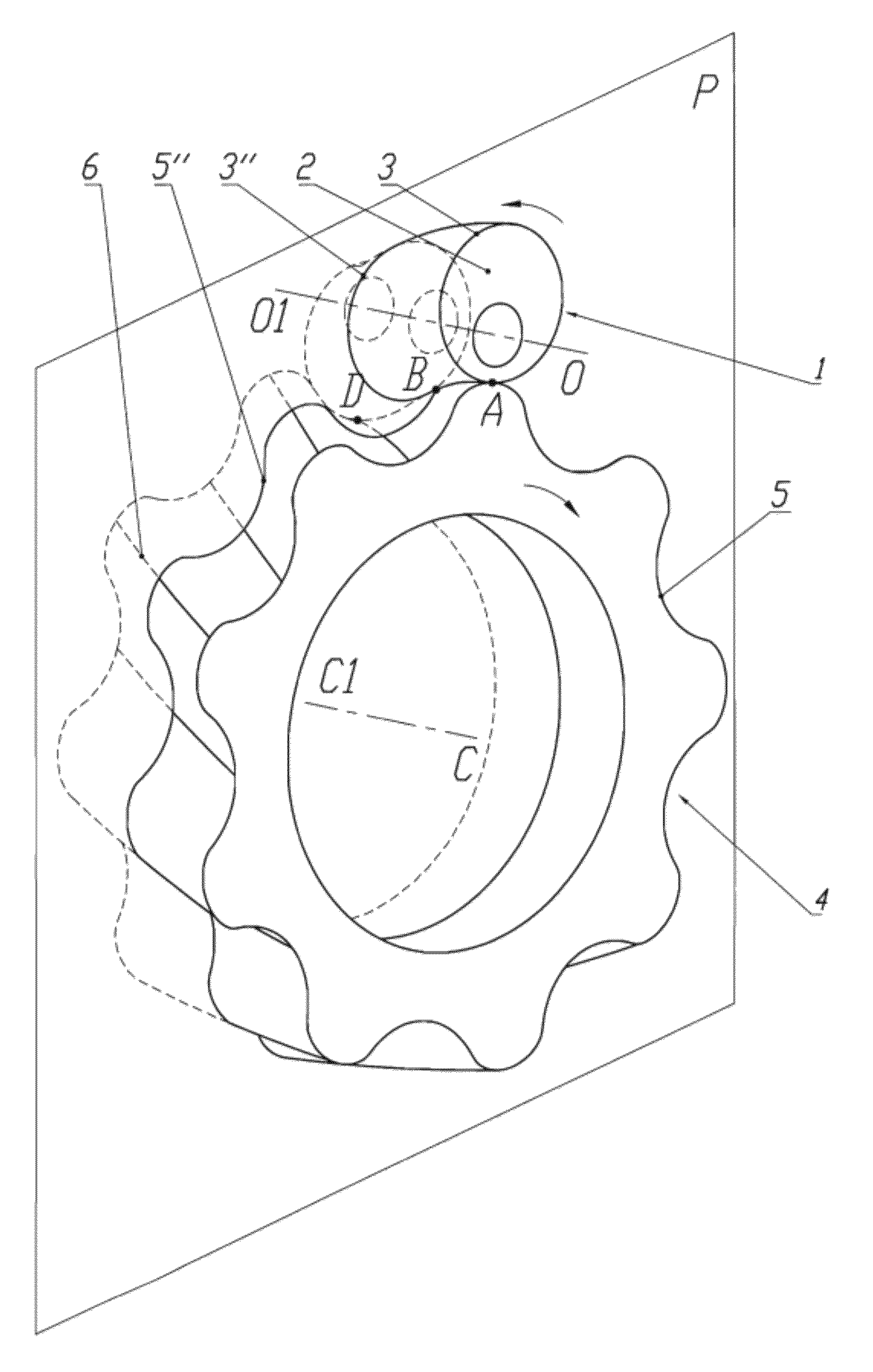
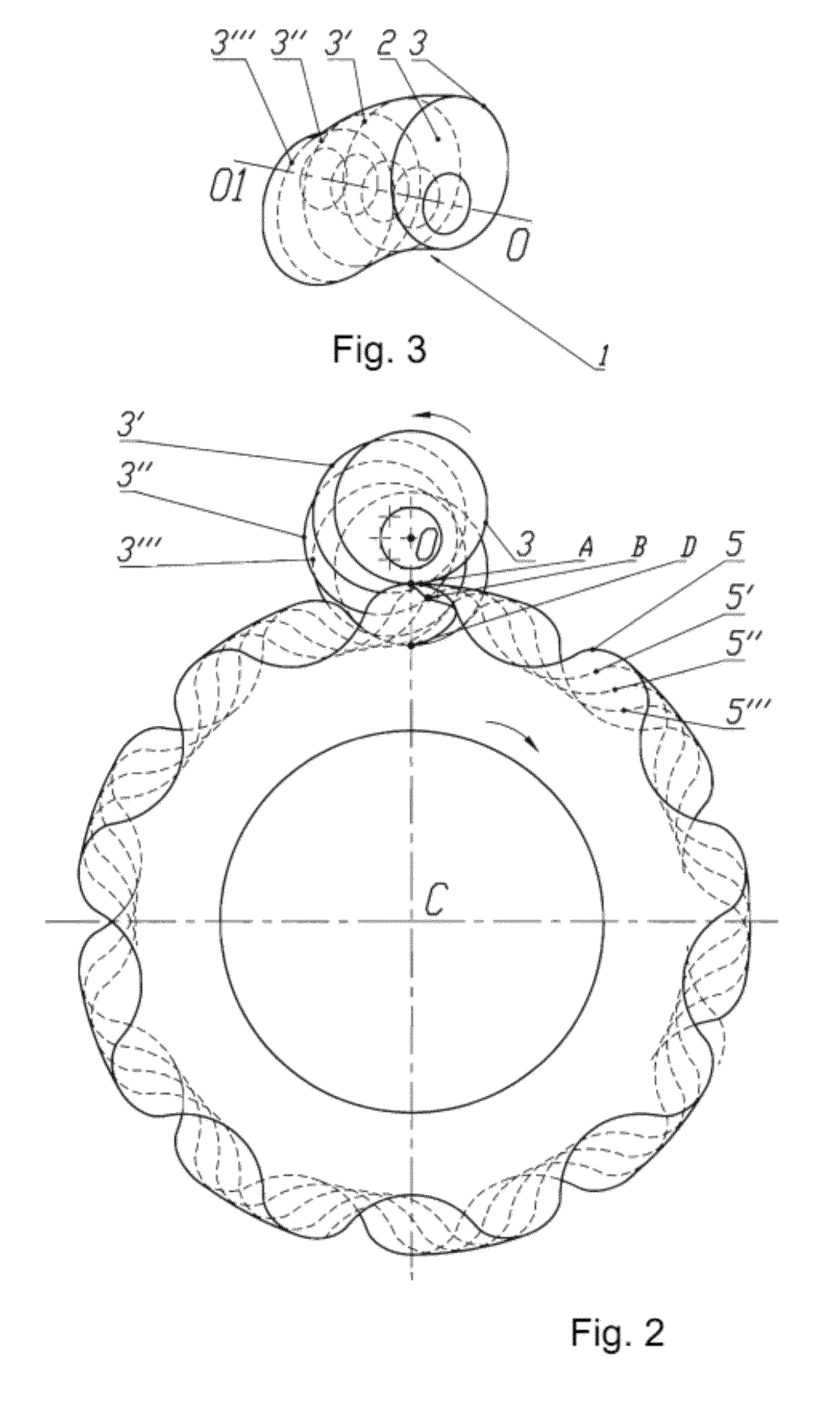
InactiveUS8157691B2Reliable engagementIncrease gear ratioToothed gearingsGearing detailsCycloidAxial load
The engagement of gearwheels with curvilinear teeth is intended to create small-sized mechanical gears of rotational motion with big gear ratio in one stage. The smaller gearwheel—pinion (16)—has one tooth, having in its face section the shape of circumference (3), eccentrically shifted with respect to the axis OO1 of the gearwheel (16). The curvilinear helical tooth of the gearwheel (16) (helical eccentric) is generated by sequential shifting of the circumference 3 along the axis OO1 and its continuous turning around the axis. The greater gearwheel (17) has helical teeth, generated by turning of the cycloidal curve (5), the teeth are conjugated with the helical surface of the pinion (16). The engagement has a continuous line of contact along the whole length of the tooth, where in each section a circular pin tooth and a cycloid are engaged, having minimum losses for friction. In order to eliminate axial loads, occurring in engagement of helical teeth, the gearwheels (16) and (17) are made herring-bone. The similar eccentrically cycloidal engagement can be implemented by assembled gearwheels. On the basis of the engagement one can design cylindrical gearboxes with parallel shafts, bevel gearboxes with intersecting shafts, and also planetary gears according to David and James schemes, possessing the increased load carrying capacity at smaller overall dimensions.
Owner:CLOSED TECH MARKET
Connector
ActiveUS20060046581A1Easy to insertReliable engagementCoupling device detailsEngineeringMechanical engineering
A connector in which a housing and a retainer are coupled with sufficient strength when the connector is reduced in scale. In this connector, provisional anchoring pieces and main anchoring pieces are provided at two end portions of an inner peripheral wall of an insertion hole formed in the housing, with lengths along a direction that intersects a direction of insertion of the retainer into the insertion hole. At outer sides of two end portions of the retainer, anchorage pawls, which are formed to be engageable with the main anchoring pieces and the provisional anchoring pieces, are provided with lengths along the intersecting direction. With this structure, when the retainer is inserted into the insertion hole of the housing, the anchorage pawls engage, at two end portions of the connector, with the provisional anchoring pieces and with the main anchoring pieces.
Owner:KK TOKAI RIKA DENKI SEISAKUSHO
Multiple seal storage and transport container
ActiveUS7063212B2Increase resistancePrevent leakagePackaging corrosive chemicalsOther accessoriesAviationCross-linked polyethylene
A container suitable for storing and transporting hazardous materials such as alkali wet cell batteries in an aviation environment includes a container body and a hinged lid made of cross linked polyethylene, latches, and a triple tongue-in-groove seal around the rim of the container opening. The triple tongue-in-groove seal includes two smaller tongue-in-groove seals with a larger tongue-in-groove seal disposed therebetween. The larger tongue-in-groove structure provides structural strength to help prevent the lid from skewing when the container suffers stress such as an impact at a corner, and also helps to guide the lid and base into proper alignment when the lid is being closed. The smaller tongue-in-groove seals provide double redundant sealing to help contain hazardous materials inside the container without making the rim of the container excessively wide.
Owner:BILL THOMAS ASSOCS
Ratchet coupling means for a driving tool
Owner:HUANG DANIEL
Dashboard support
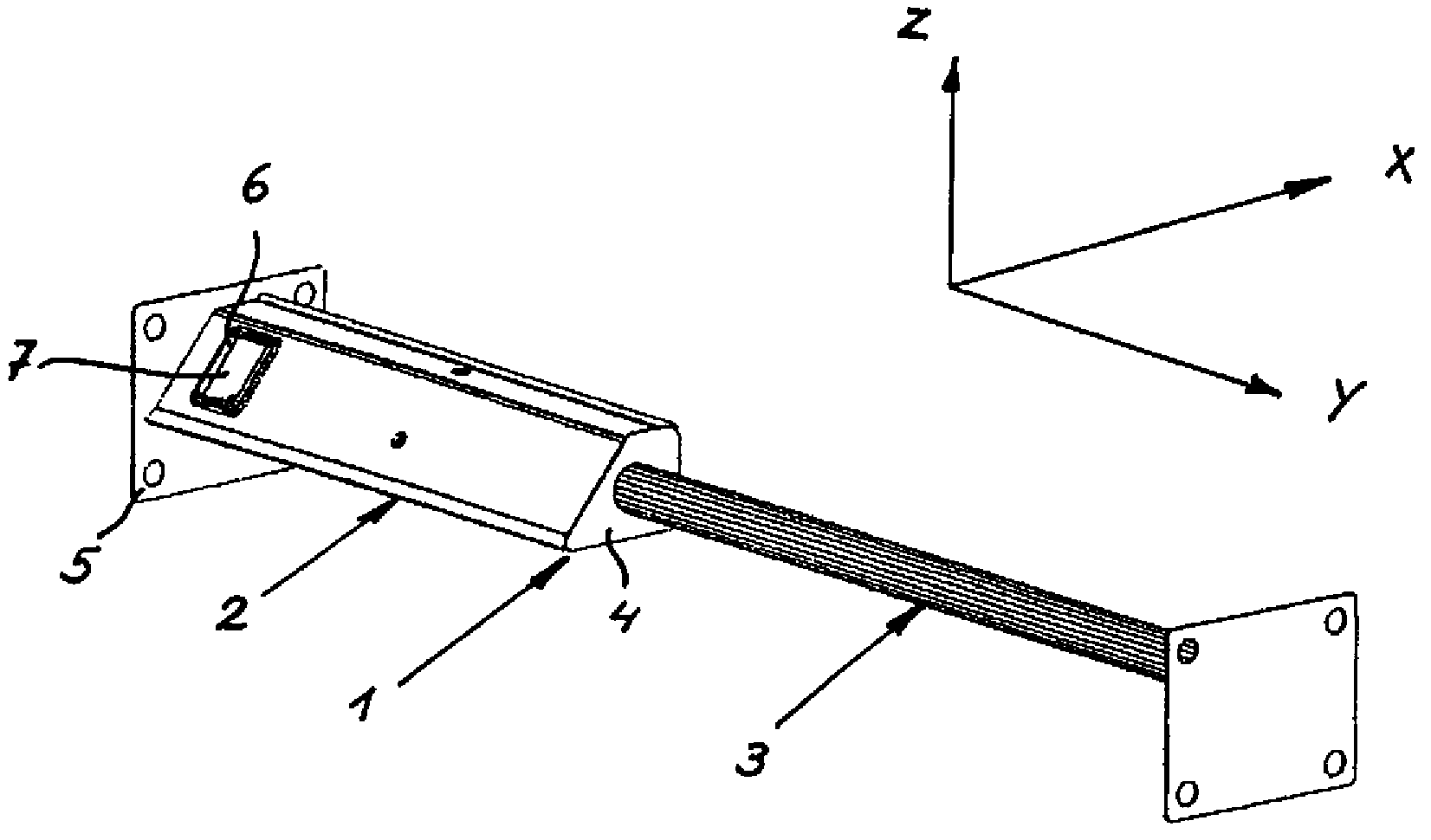
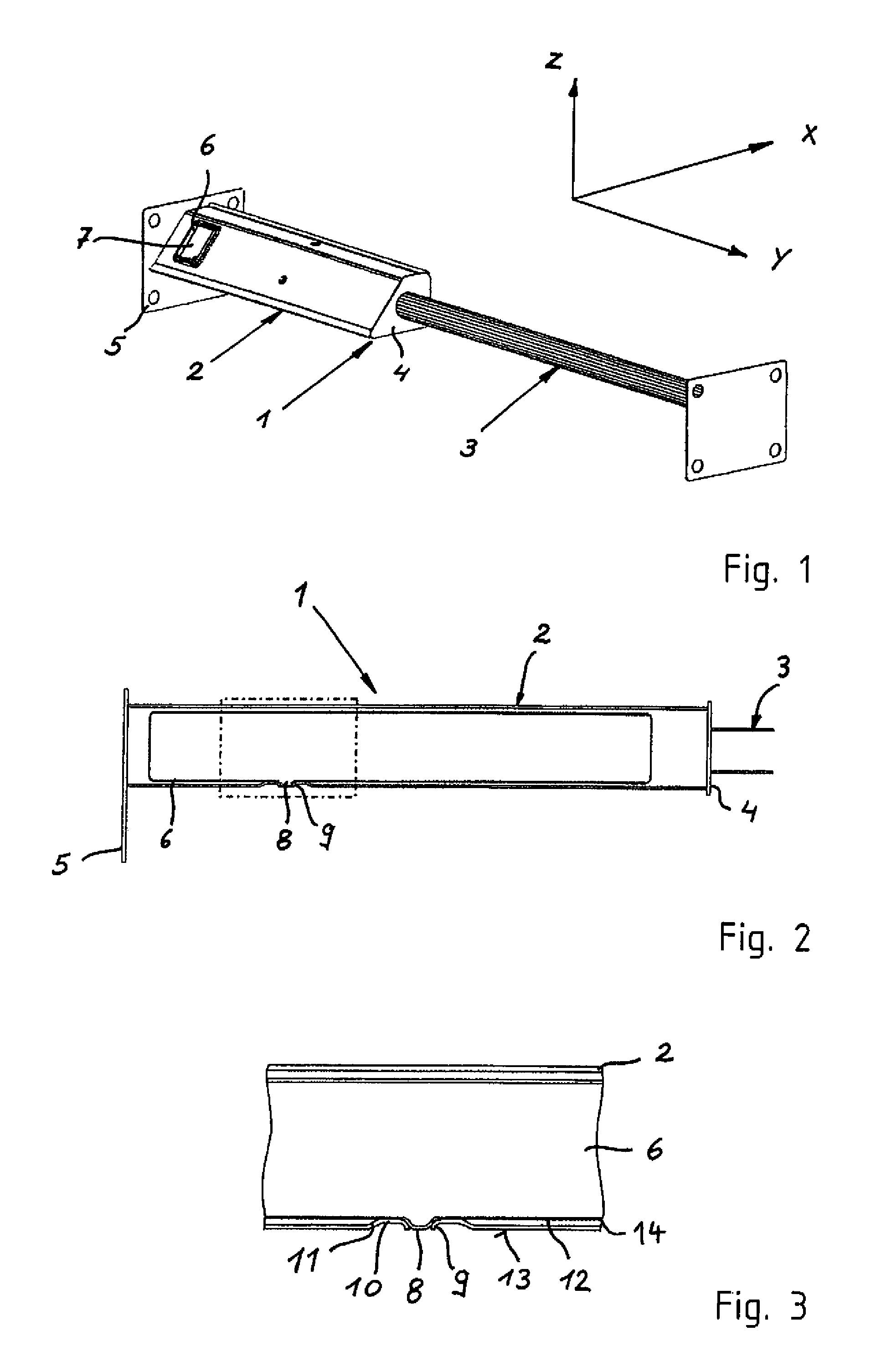
InactiveUS7810873B2Save materialSave weightVehicle seatsInstrument arrangements/adaptationsMobile vehicleDashboard
Owner:BENTELER AUTOMOBILTECHNIK GMBH
Features
- R&D
- Intellectual Property
- Life Sciences
- Materials
- Tech Scout
Why Patsnap Eureka
- Unparalleled Data Quality
- Higher Quality Content
- 60% Fewer Hallucinations
Social media
Patsnap Eureka Blog
Learn More Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.
© 2025 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com