Patents
Literature
Hiro is an intelligent assistant for R&D personnel, combined with Patent DNA, to facilitate innovative research.
45results about How to "Achieving precise measurements" patented technology
Efficacy Topic
Property
Owner
Technical Advancement
Application Domain
Technology Topic
Technology Field Word
Patent Country/Region
Patent Type
Patent Status
Application Year
Inventor
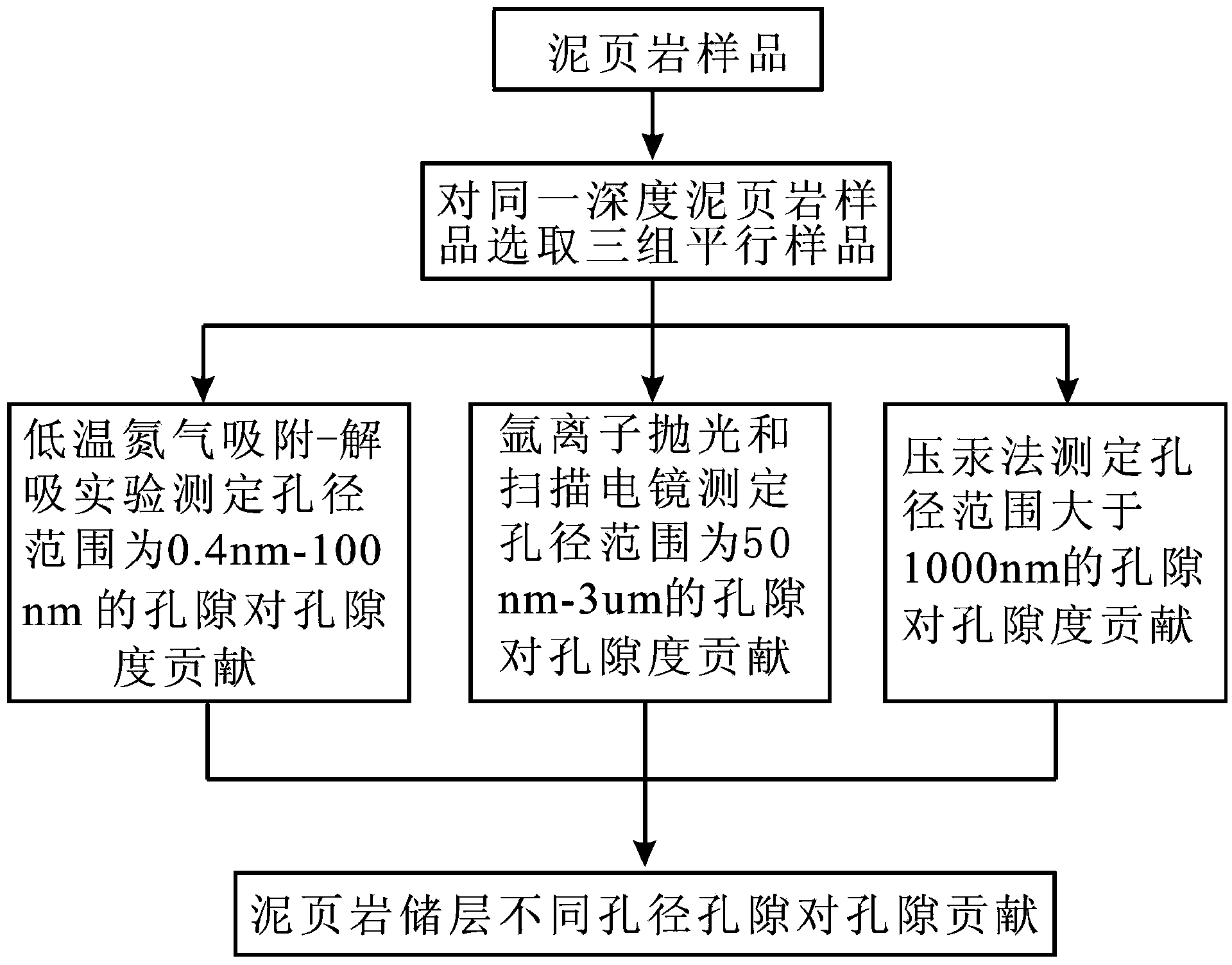
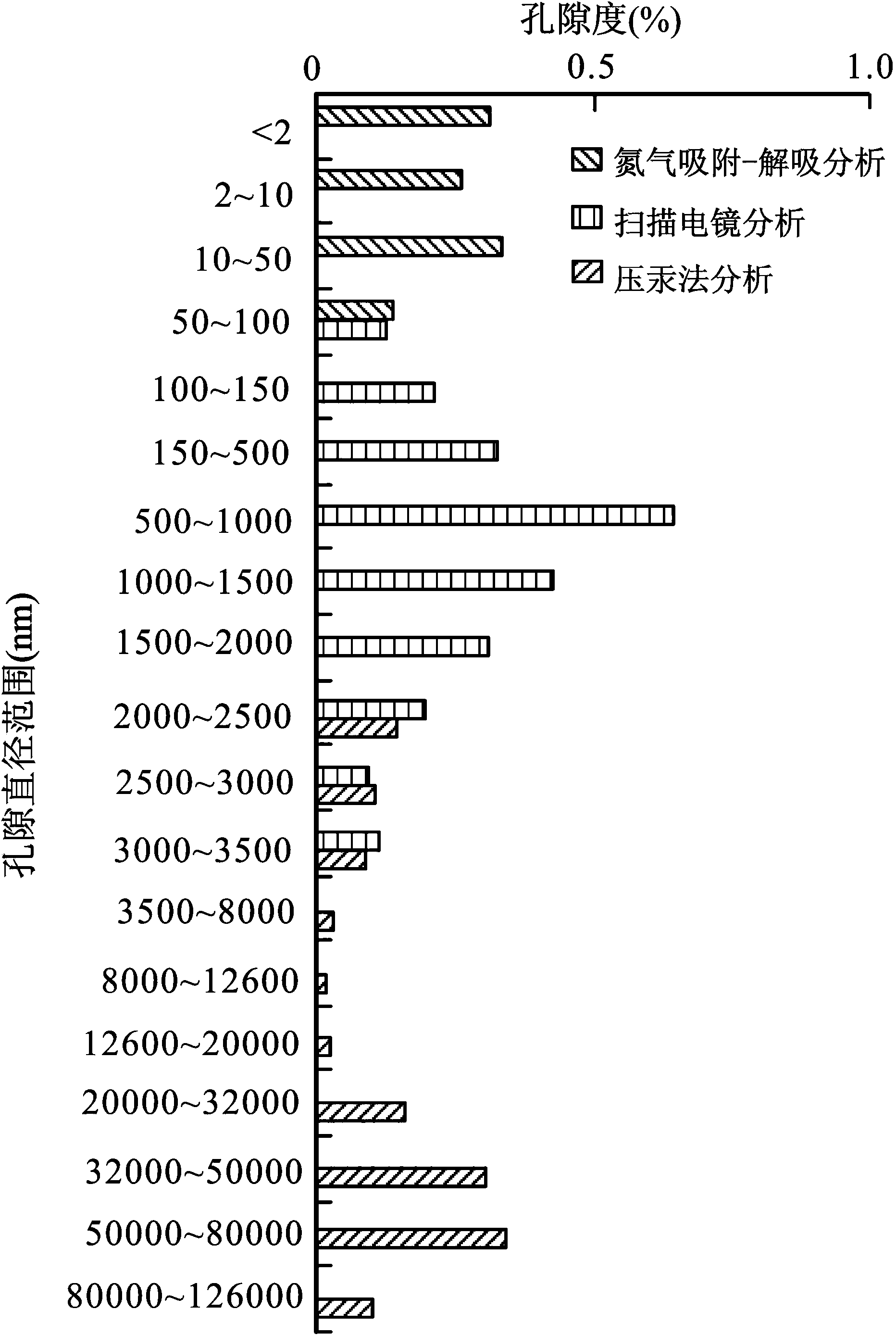
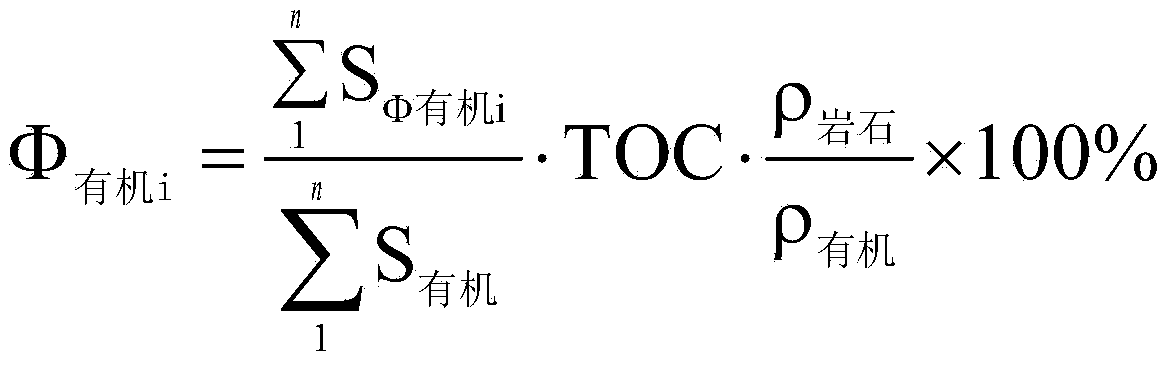
Method for determining contribution of pores with different apertures in shale reservoir stratum to porosity
InactiveCN103512838AAchieving precise measurementsEasy to operatePermeability/surface area analysisPorosityAnalysis data
The invention discloses a method for determining contribution of pores with different apertures in a shale reservoir stratum to porosity, and belongs to the technical field of petroleum, geology and mining exploration and development. The method comprises the following steps: selecting three groups of parallel samples from shale reservoir stratum samples at the same depth; carrying out a low-temperature nitrogen adsorption-desorption experiment on the first group of samples to determine contribution of the pores with aperture ranging from 0.4-100 nm to the porosity; carrying out argon ion polishing and electron scanning microscope examination on the second group of samples to determine the contribution of the pores with aperture ranging from 50 nm to 3 microns to the porosity; carrying out mercury intrusion analysis on the third group of samples to determine the contribution of the pores with aperture greater than 1000 nm to the porosity; synthesizing the analytic data of the three groups of parallel samples, and obtaining the determination results of contribution of the pores with different apertures in the shale reservoir stratum to the porosity. The method can determine the contribution of the pores with different apertures in the shale reservoir stratum to the porosity, and overcomes the defect of the conventional gas adsorption method and the mercury intrusion method for determining the contribution of the pores with different apertures in the shale reservoir stratum to the porosity.
Owner:CHINA UNIV OF PETROLEUM (EAST CHINA)
Fiber Bragg grating-based extensible distributed sensing system
InactiveCN102661755AAchieving precise measurementsLow costConverting sensor output opticallyFiberGrating
The invention relates to a fiber Bragg grating-based extensible distributed sensing system. The system is technically characterized by comprising a broadband light source, an optical loop device, a plurality of fiber Bragg grating sensors with different grating periods, an extensible light-wave demultiplexing separator, an optical etalon, a plurality of light sensing signal detectors and a signal processing system, wherein the output of the broadband light source is connected to an optical fiber with a distributed fiber Bragg grating through the optical loop device; light reflected from the fiber Bragg grating is output to the input port of the extensible light-wave demultiplexing separator from a port of the optical loop device, and is output to the optical etalon through each output port of the extensible light-wave demultiplexing separator; the light sensing signal detectors receive a light beams output from the optical etalon; and the signal processing system performs real-time signal processing on each sensing signal. The system is reasonable in design; and the invention has the characteristics that the system is low in cost, high in real-time performance, easy to extend and produce and the like.
Owner:GP PHOTONICS INC
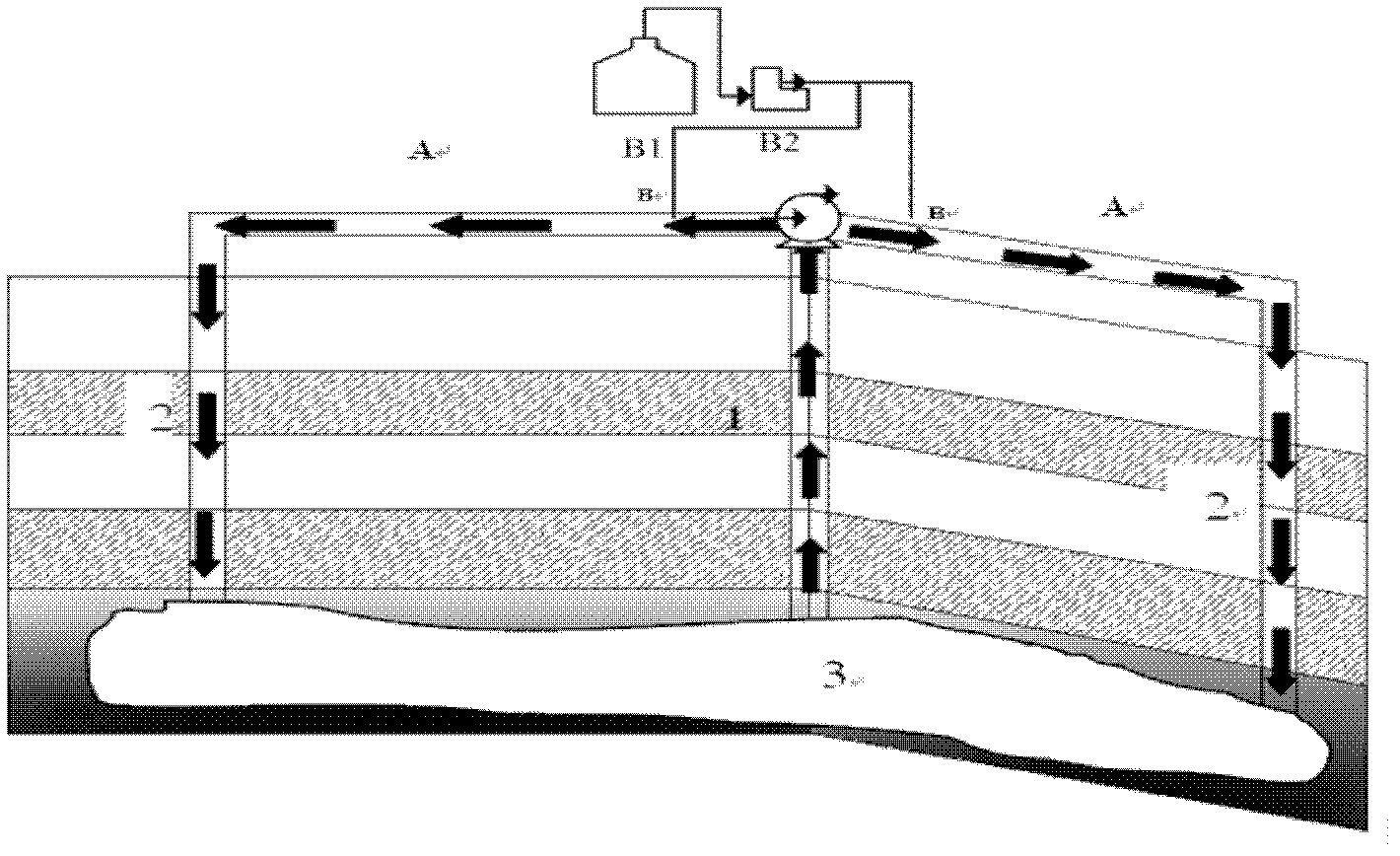
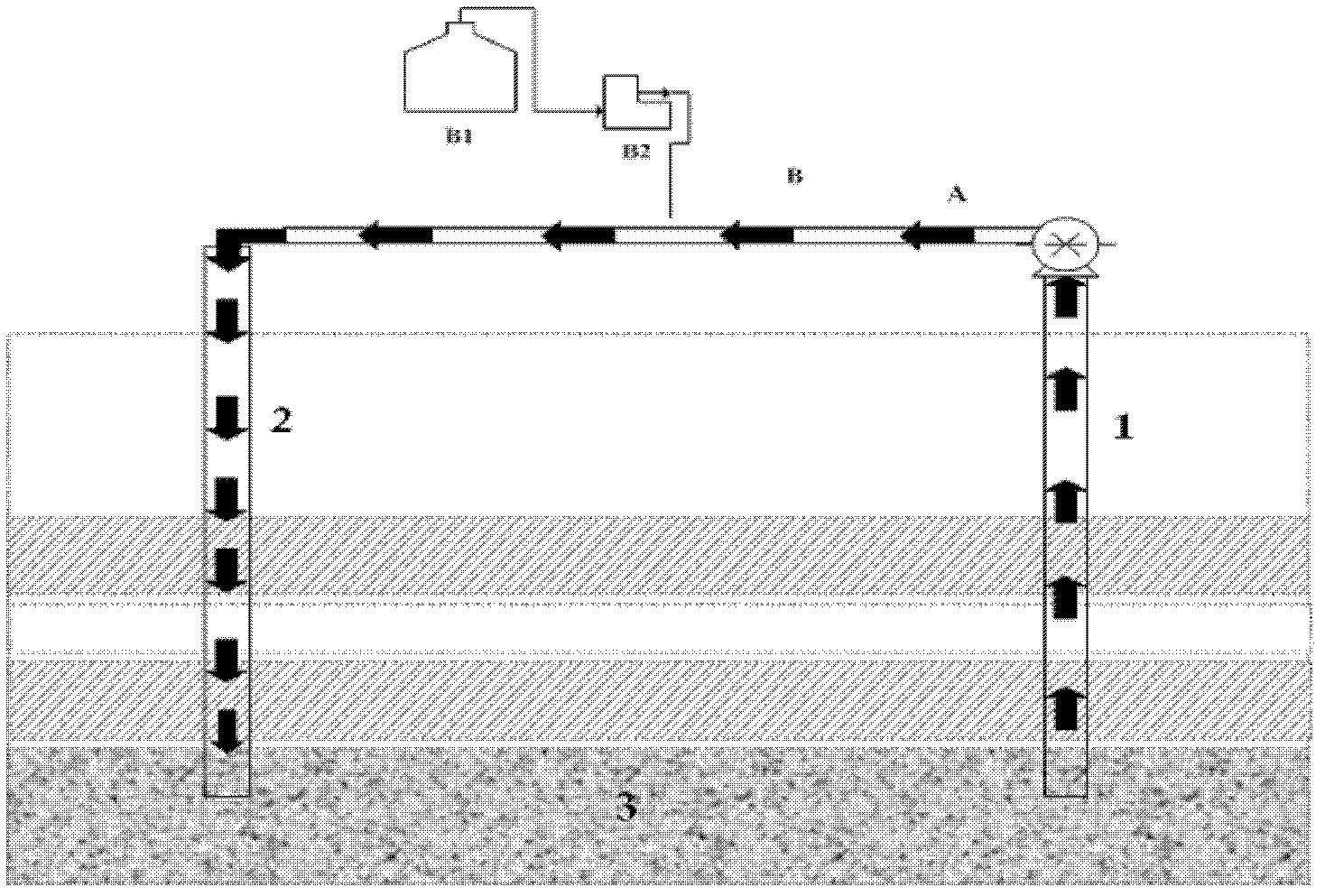
Underground cavity volume detection method and underground cavity volume detection device
ActiveCN102607663AAccurate measurementShort cycleContainer/cavity capacity measurementEnvironmental geologyCombustion
The invention belongs to the fields of geology and detection and relates to an underground cavity volume method and an underground cavity volume detection device. Particularly, the underground cavity volume detection method includes the following steps of 1) filling water into an underground cavity or keeping the underground cavity full of water; 2) adding detectable reagent of known quantity of A0mg into the underground cavity; 3) dispersing the detectable reagent in the step 2) in the water uniformly; 4) detecting concentration C' mg / L of the detectable reagent in the water; and 5) computing the volume V' of the water filled in the underground cavity according to the following formula, and obtaining the volume V of the underground: V=V'=A0 / C' / 1000m3. The invention further discloses the underground cavity volume detection device. The underground cavity volume method is capable of accurately measuring the volume of the underground cavity, particularly an empty combustion area and is short in cycle, free of risk, fine in repeatability and simple in process.
Owner:ENN SCI & TECH DEV
Detecting method and its device for double light beam-two angle super short pulse laser pumping
InactiveCN1945342AAchieving precise measurementsResistance/reactance/impedenceMaterial analysis by optical meansBeam splitterOptoelectronics
The present invention relates to ultrashort pulse laser testing technology, and is especially pumping and detecting method and device of double beam and two angle ultrashort pulse laser. The pulse laser beam the ultrashort pulse laser emits is split into two beams in the beam splitter, includes one as the pumping laser beam and one as the detecting laser beam. The detecting laser beam is delayed in a delay line and split in a beam splitter into two beams incoming to the surface of the sample in different angle, two photo detectors receive the reflected signals independently, the signals are acquired with one phase-lock amplifier and one computer, and the data are processed in the computer to obtain the optical characteristic curve of the sample. The present invention can measure both dielectric constant and light beam polarizing value of material, and realize precise determination of physical parameters in the conversion process in the time resolution of femtosecond level.
Owner:JIANGSU UNIV
Laser gas detection method with automatic linearity correction function
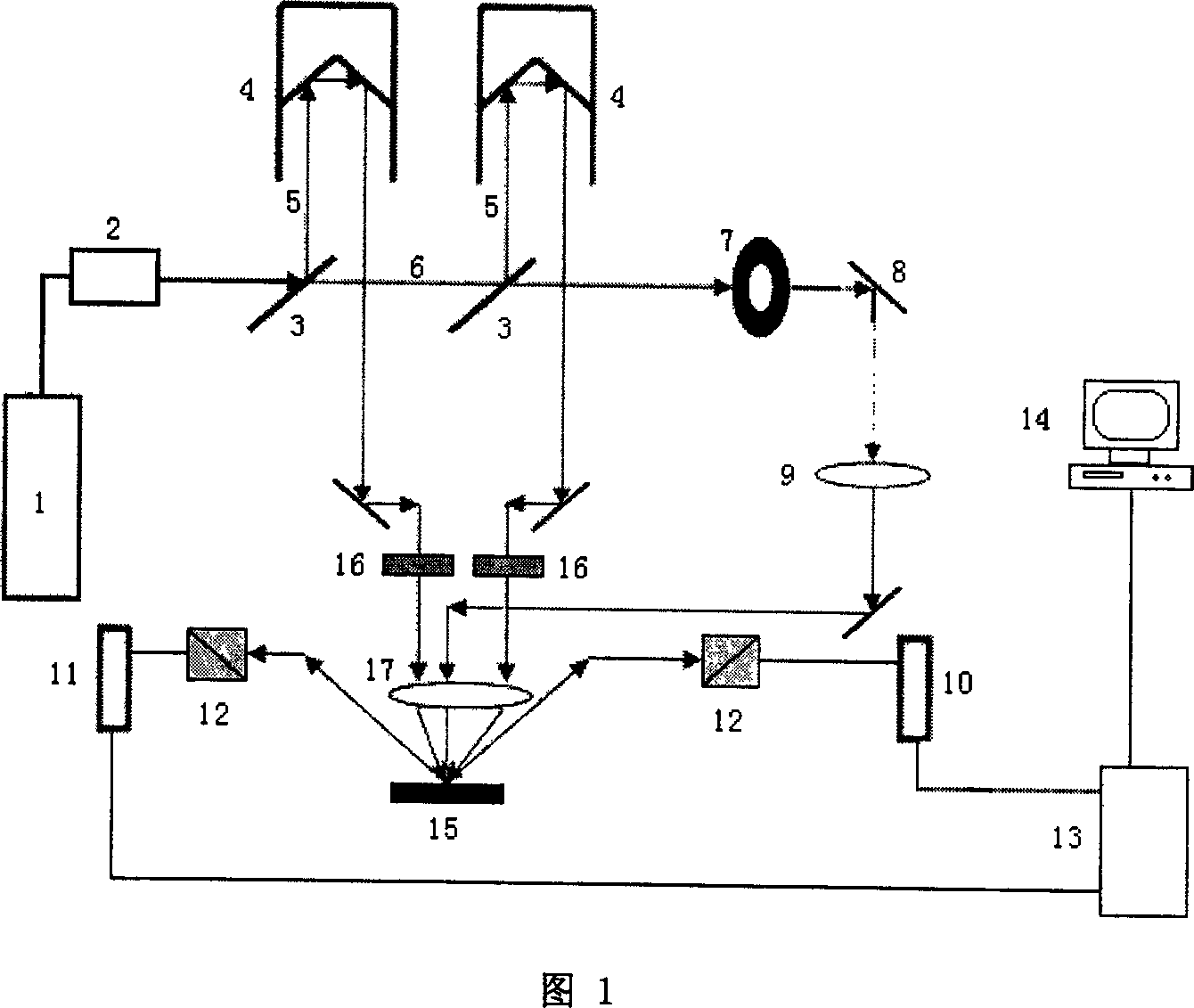
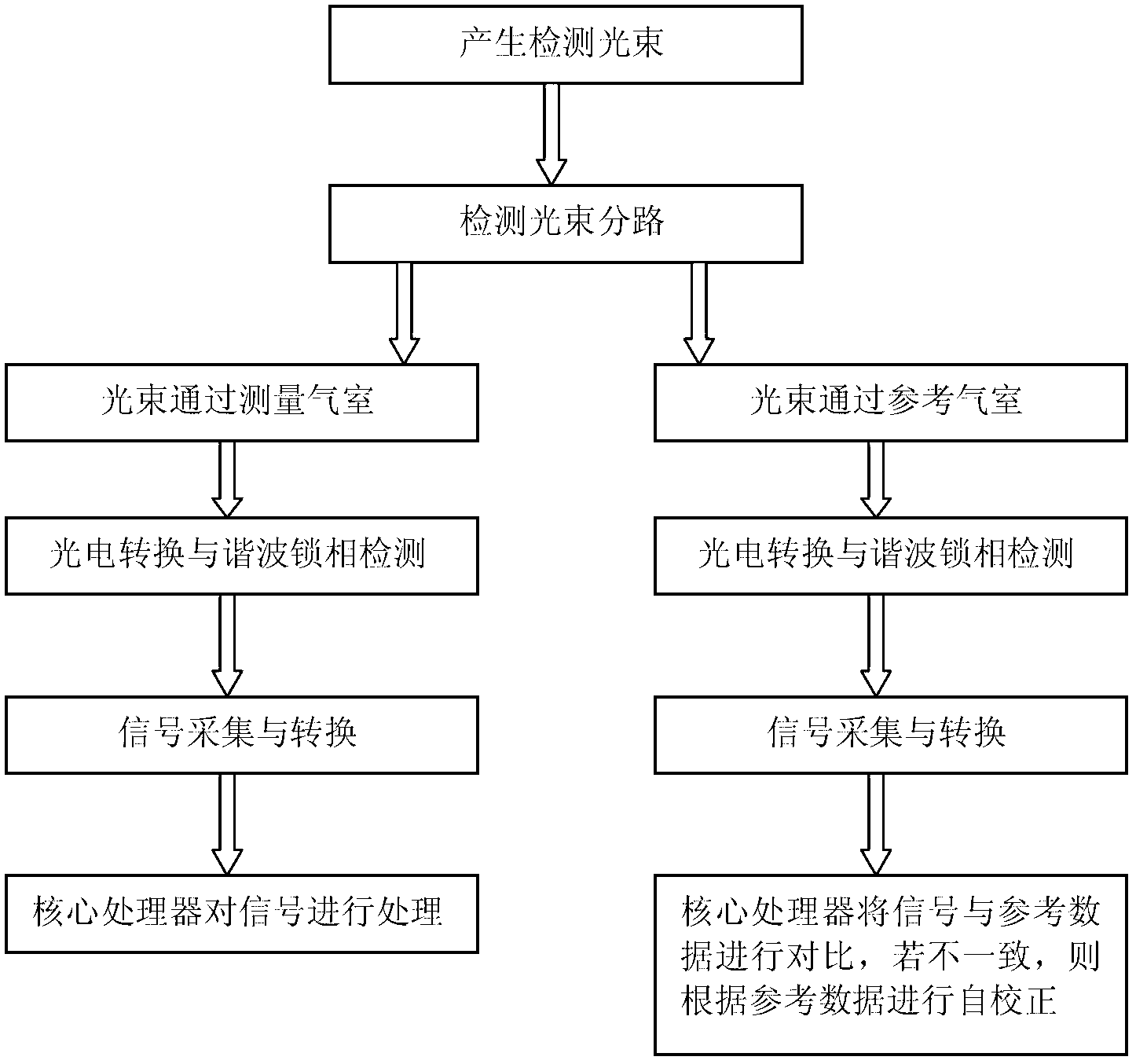
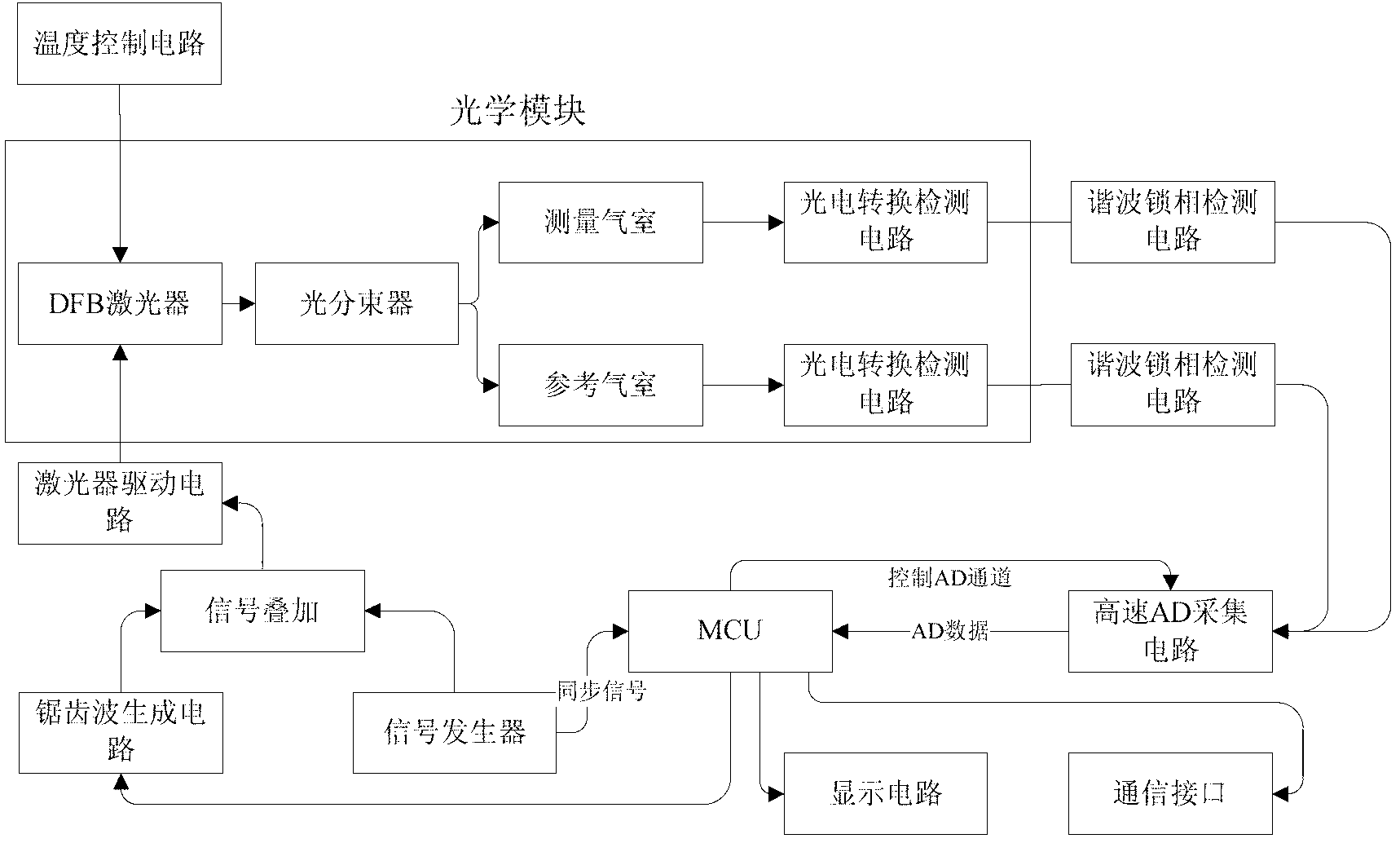
ActiveCN103196852AAchieving precise measurementsGuaranteed stabilityColor/spectral properties measurementsCorrection algorithmOpto electronic
The invention discloses a laser gas detection method with an automatic linearity correction function, and belongs to the field of mining industry gas concentration measurement. The method comprises the following steps of: generating a detection light beam, branching the detection light beam, enabling the branched light beams to pass through a measuring air chamber and a reference air chamber, carrying out photovoltaic conversion and harmonic wave phase locking detection, then carrying out collecting and converting and finally sending the treated light beams into a core processor; for a signal generated by the light beam passing through the reference air chamber, comparing a detected gas concentration value and a central wavelength position with two reference data values recorded during calibration at the first time by the core processor, and if the values are different, starting a linearity correction algorithm, and correcting the device; and for a signal generated by the light beam through the measuring air chamber, displaying and sending by the core processor according to a detected gas concentration value. The method provided by the invention can accurately measure gas concentration in a mine and can realize the automatic correction in a detection process, thus the underground maintaining times for workers are reduced.
Owner:CHINA COAL TECH & ENG GRP CHONGQING RES INST CO LTD
High-precision temperature probe on basis of cavity filled micro-structure optical fiber interferometers
ActiveCN109655176ACompact structureAchieving precise measurementsThermometers using physical/chemical changesMicro structureLight signal
The invention discloses a high-precision temperature probe on the basis of cavity filled micro-structure optical fiber interferometers. The high-precision temperature probe mainly comprises a light source, an object lens, a semi-transparent and semi-reflective mirror, an optical fiber structure and a detector. The optical fiber structure comprises an optical fiber core, optical fiber cladding, a temperature-sensitive chamber, a first reflective coating and a second reflective coating; emergent light emitted by the light source can be transmitted through the object lens into the optical fiber core via the semi-transparent and semi-reflective mirror, and one part of the light is reflected to the first reflective coating when the light source reaches the surfaces of the temperature-sensitivechamber, and then returns on an original path to be transmitted into the optical fiber core; the other part of the light is directly transmitted into the temperature-sensitive chamber, and then returns on an original path to be transmitted into the optical fiber core after the other part of the light reaches the second reflective coating, and two channels of returned light signals can be reflectedto the detector via the semi-transparent and semi-reflective mirror. The high-precision temperature probe has the advantages that the high-precision temperature probe can be applied to accurately monitoring the temperatures in narrow and small spaces and high-corrosion environments, and different sensitive materials can be selected according to the actual requirements, so that different parameters can be monitored in real time.
Owner:NORTHEASTERN UNIV
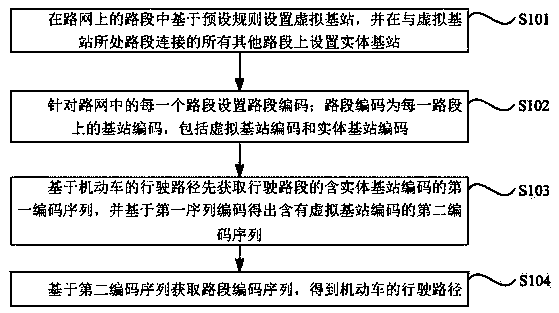
Accurate determination method of vehicle driving path in road network
InactiveCN102568211AAchieving precise measurementsReduce the number of layoutsRoad vehicles traffic controlRoad networksComputerized system
The invention relates to an accurate determination method of a vehicle driving path in a road network. An entity base station and a computer system are included. And the method comprises the following steps that: (1), numbering is carried out on all road sections in a whole road network with unique codes in the computer system; (2), a virtual base station is laid at any road section of the road network and entity base stations are laid at any other road sections, wherein the any other road sections are connected at two ends of the road section; and according to the above-mentioned laying rule, laying of the whole road network is completed; and (3), an entity base station number that is determined by the entity base station and a vehicle passes by during the driving is introduced into the computer system; and according to the laying rule of the entity base stations and the virtual base stations, it is concluded that at least one virtual base station is arranged between two adjacent entity base stations and thus a whole vehicle driving path is accurately reckoned. According to the invention, the used system has a simple structure; the laying number of the entity base stations is lowered; and whole investment cost and maintenance cost are reduced; therefore, the provided method is a reliable accurate determination method of a vehicle driving path in a road network.
Owner:GANSU WANHUA JINHUI INFORMATION TECH
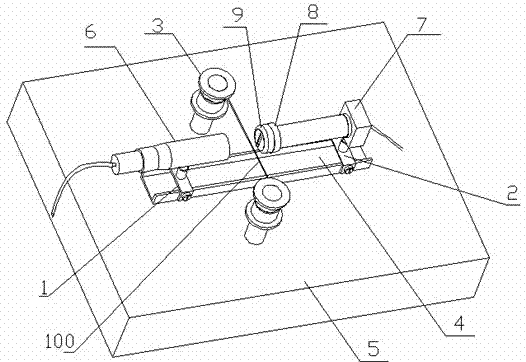
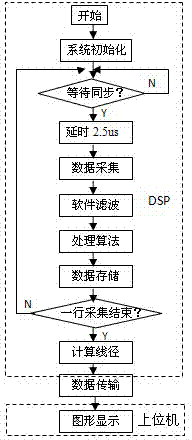
Digital signal processor (DSP) based linear array charge coupled device (CCD) online detection system for absorbable suture line diameter
InactiveCN104121860AAchieving precise measurementsImprove uniformityUsing optical meansClosed loopAbsorbable suture
The invention relates to a digital signal processor (DSP) based linear array charge coupled device (CCD) online detection system for absorbable suture line diameter. The DSP based linear array CCD online detection system for absorbable suture line diameter comprises structural members and a system circuit. The structural members include a light source support, a CCD support, guide wheels and a guide rail. The guide wheels and the guide rail are fixed to the surface of a platform of a wet spinning machine. A linear array CCD comprises a linear array CCD module and a casing; a plug rod B is arranged at the bottom of the casing and is in close fit with a central slot of a holder B. A telecentric lens is mounted at the front end of the linear array CCD and provided with a shading device. The shading device consists of a lens hood and a shade piece. The system circuit consists of an optical imaging module, a linear array CCD drive module, a high-speed analog / digital (A / D) data acquisition and DSP digital signal processing module. Compared with the conventional measurement method, the DSP based linear array CCD online detection method for the absorbable suture line diameter has the advantages that the line diameter can be detected in real time accurately during production and twisting of absorbable sutures, a DSP controller output end provides detection data for a spinneret mechanism and a tension control system to realize closed-loop control of spinneret pressure and stretch tension, thus the produced sutures have good uniformity.
Owner:TIANJIN POLYTECHNIC UNIV
Method for detecting trace elements in pyrite
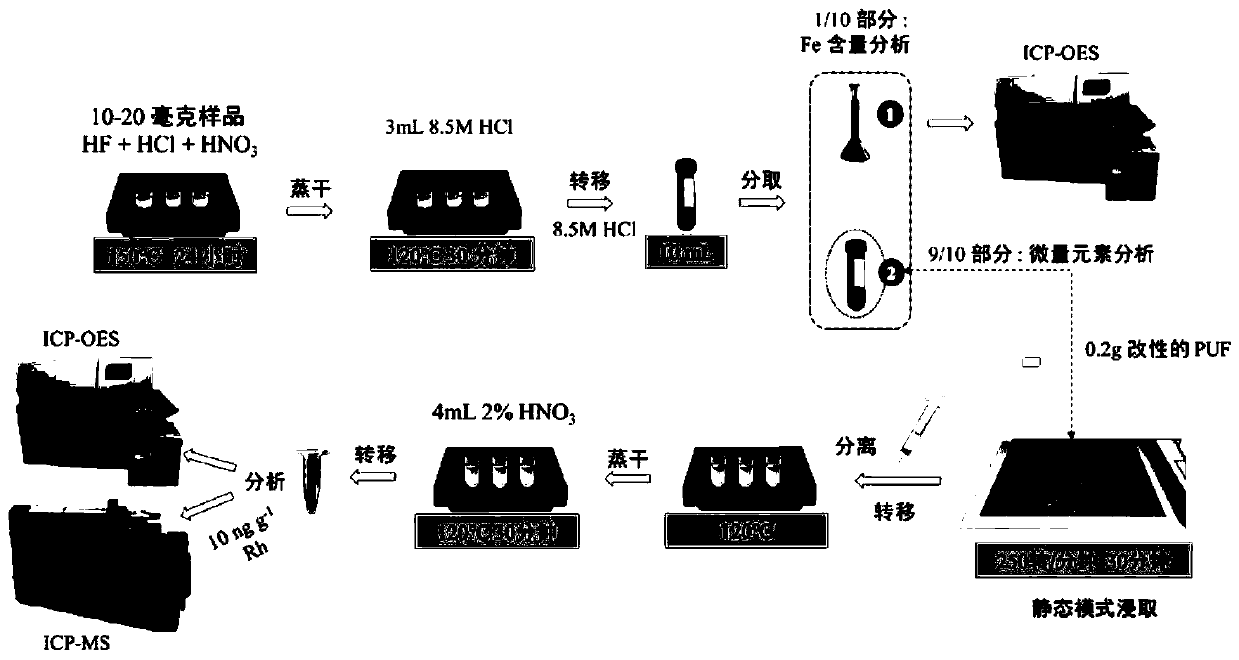
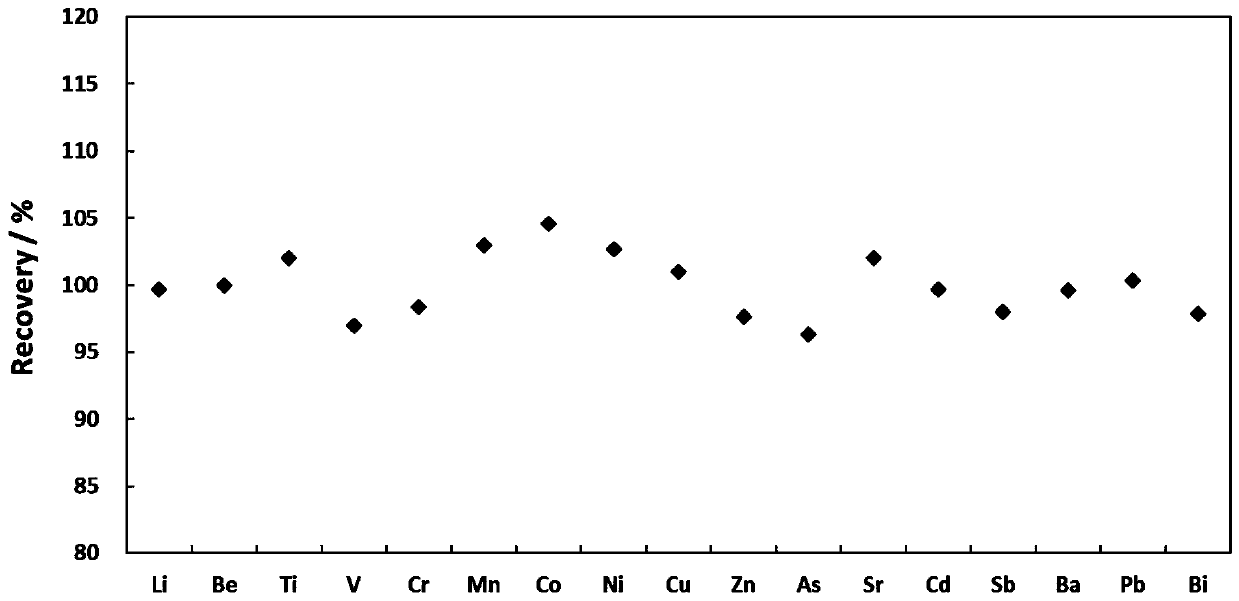
ActiveCN111413189AAchieving precise measurementsImprove adsorption capacityPreparing sample for investigationMaterial analysis by electric/magnetic meansSpecific adsorptionPyrite
The invention discloses a method for detecting trace elements in pyrite, which comprises the following steps of adsorbing an iron matrix in pyrite by using modified polyurethane foam, determining theadsorption efficiency of the modified polyurethane foam to the iron matrix by ICP-OES, and detecting the content of the trace elements by ICP-MS and ICP-OES. The modified polyurethane foam provided bythe invention is polyurethane foam modified by isothiocyanate and / or mercaptan, has high adsorption rate on an iron matrix in the pyrite, is beneficial to recovery of trace elements in the pyrite, and enables the recovery rate of 17 trace elements in the pyrite to be 95-103%. The modified polyurethane foam provided by the invention has a specific adsorption effect on an iron matrix, and is high in adsorption capacity and resistant to acid, so that the purpose of rapidly, accurately and accurately detecting trace elements in pyrite is achieved.
Owner:INST OF GEOLOGY & GEOPHYSICS CHINESE ACAD OF SCI
Improved hydrostatic pressure testing device and testing method
ActiveCN109341931AEasy to measureAccurate measurementFluid pressure measurement using elastically-deformable gaugesEducational modelsWater storageHydrostatic pressure
The invention discloses an improved hydrostatic pressure testing device and a testing method, and the device comprises a water storage device, a control mechanism, a leveling device, a pressure bearing device, and a measuring transmission device. The water storage device is used for supporting the whole device and temporarily storing the water for experiment. The control mechanism is used for controlling the water stored in the support to flow between the tank and the support. The leveling device is used for setting the tank to be in a horizontal state. The pressure bearing device is used forconverting the hydrostatic pressure into the rotational torque of the bearing body. The measuring transmission device is used for converting the rotational torque of the pressure bearing device into adeformation of the torsion spring, by which the torque of the torsion spring is obtained, thereby obtaining the value of the hydrostatic pressure, and further obtaining a quantitative relationship between the hydrostatic pressure and the water depth. The improved hydrostatic pressure testing device and the testing method are simple in structure and clear in principle, therefore, the accurate measurement of hydrostatic pressure, the law of hydrostatic pressure in different water depths, and the hydrostatic pressure action point can be obtained through the technical design of the invention.
Owner:HOHAI UNIV
Method for determining fatty acid in rice by using gas chromatography-mass spectrometry
ActiveCN110736801AImprove accuracyAvoid interferenceComponent separationGas liquid chromatographicButylated hydroxytoluene
The invention discloses a method for determining fatty acid in rice by using the gas chromatography-mass spectrometry. The method comprises the following steps: (1) preparation of a sample to be determined: pulverizing a rice sample, sequentially adding n-hexane, an acetyl chloride-methanol solution and butylated hydroxytoluene, mixing, and heating to perform fatty acid extraction and methyl esterification treatment; cooling to room temperature, adding a sodium carbonate solution, uniformly mixing, centrifuging, taking supernatant, and filtering to obtain a sample to be detected; and (2) determination of fatty acid components: performing quantitative or qualitative determination on 37 kinds of fatty acids of the sample obtained to be detected in the step (1) by adopting a gas chromatograph-mass spectrometer. According to the present invention, through the common cooperation of the extraction method and the determination method, the accurate qualitative and quantitative determination of37 kinds of fatty acids in rice is achieved, the difficult problem that the fatty acid components in rice cannot be accurately determined in the prior art is solved, and the determination of a variety of fatty acids can be achieved on the basis of the assurance of the determination accuracy of the fatty acid components.
Owner:山东国仓健生物科技有限公司
Method for rapidly assaying activity of DNA polymerase
PendingCN112176040AEasy to operateGood accuracy and repeatabilityMicrobiological testing/measurementBiological material analysisOligonucleotideEnzyme
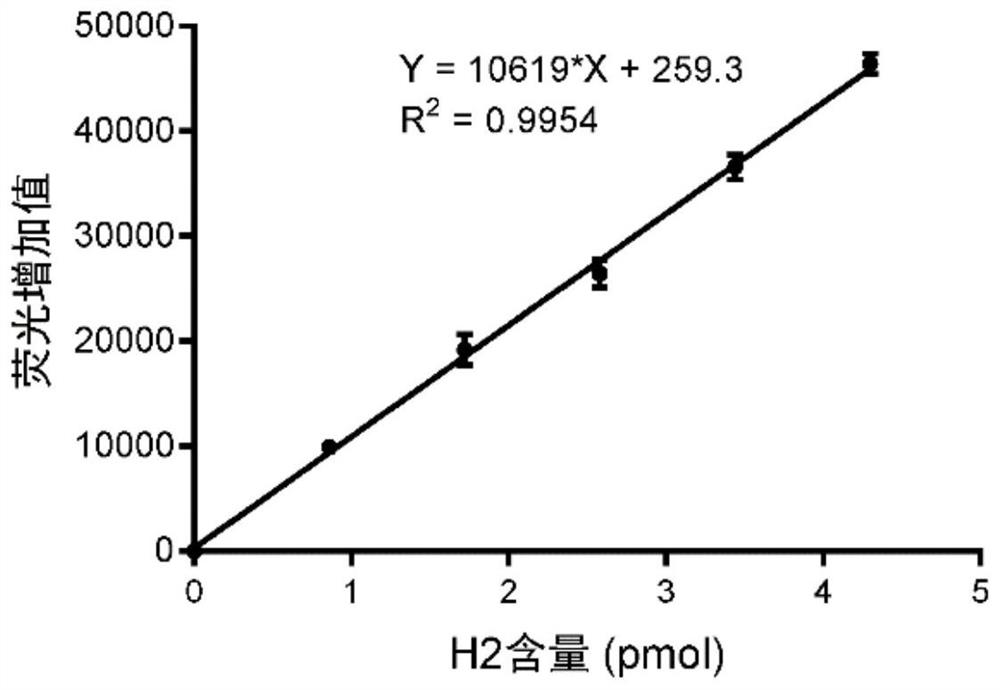
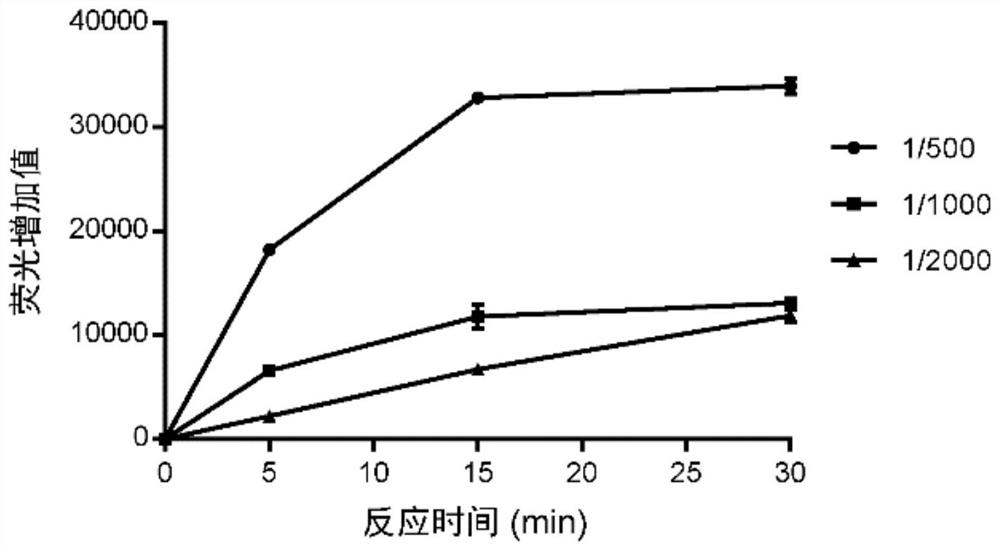
The invention discloses a method for rapidly assaying activity of DNA polymerase. The method comprises the steps: (1) drawing a standard curve and calculating an equation of linear regression: mixingtwo kinds of oligonucleotide probes H1 and H2 by different proportions, measuring fluorescence values, drawing the standard curve, and calculating the equation of linear regression; (2) carrying out aDNA polymerase activity detecting reaction under isothermal conditions: employing a working solution only containing H1 obtained in the step (1) as a substrate, adding a dNTP mixed solution and magnesium ions, and preparing a DNA polymerase activity detecting reaction system by employing a reaction buffer corresponding to the DNA polymerase; (3) determining a fluorescence value of a product afterstopping the reaction: forcefully stopping the reaction after an isothermal reaction, cooling the temperature of a system to room temperature, then, assaying the content of the product, and recordinga fluorescence added value in 30min; and (4) calculating enzymatic activity: substituting the fluorescence added value into the equation of linear regression corresponding to the standard curve obtained in the step (1) to obtain the content of the product in the system, and calculating the activity of raw enzyme liquid. Independent of isotopes, the operation is simple, and the accuracy and repeatability are good.
Owner:JIANGSU MARINE RESOURCES DEV RES INST LIAN YUNGANG +1
Soil moisture content detection method based on TDR (time domain reflectometry)
InactiveCN107478681AAchieving precise measurementsMaterial analysis by electric/magnetic meansMaterial weighingTime-domain reflectometerTime domain
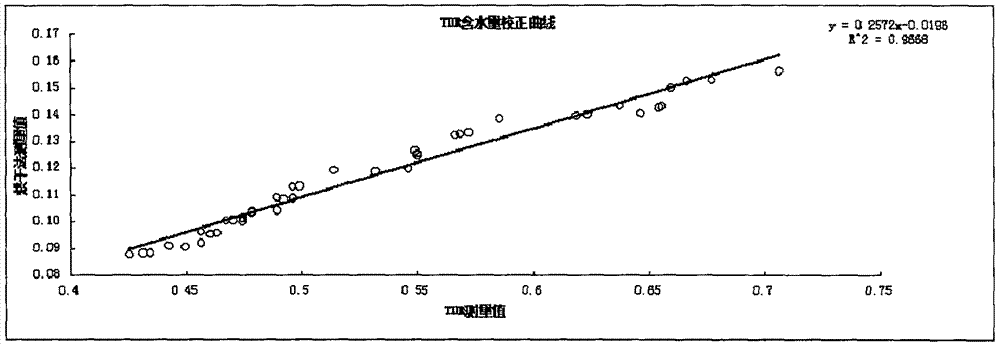
The invention discloses a soil moisture content detection method based on a TDR (time domain reflectometry). A collected soil sample is spread and aired in a shade place, the aired soil sample is manually broken off with hands, impurities are removed, and the soil sample passes through a 2 mm soil sieve and put into a round PVC barrel; marks are made in the round PVC barrel at the interval of 5 mm, six scale marks are 30 m in total, and then, soil is compacted for each scale mark layer by layer until the soil is 30 cm high; after the soil column is filled and compacted, the soil is saturated after moisture enters the soil, a container is weighed on an electronic balance, bottom moisture is required to be toweled off before weighing, and numerical values are recorded; after weighing, the TDR is inserted in the soil column, a probe inserting point is the center of the soil column, then measurement is performed once every 3-5 h, and weighing is performed during measurement; TDR data are corrected by the following linear function: y=0.2769+0.0554 (R2=0.9668), wherein x is the moisture content measured by the TDR while y is the actual moisture content of the soil.
Owner:NINGXIA UNIVERSITY
Unidirectional tensile high-temperature mechanical property test system and method for sheet material
ActiveCN110672427AHeating evenlyElongation effectCoil arrangementsMaterial strength using tensile/compressive forcesControl systemMechanical property
The present invention discloses a unidirectional tensile high-temperature mechanical property test system and method for a sheet material, which realizes the determination of the basic mechanical properties of a unidirectional tensile test on the sheet material at a high temperature. The system comprises a tensile test device and two heating devices symmetrically arranged on the tensile test device; the tensile test device comprises a workbench, two clamps arranged on the workbench, and a tensile test piece clamped between the two clamps; the heating device comprises a PLC control system, an induction heating power supply, an induction coil, an iron plate and an iron plate support frame; the iron plate support frame is arranged on the workbench; the iron plate is fixedly arranged on the iron plate support frame; one side of the iron plate is parallel to the test piece, and the other side is parallel to the induction coil; the induction heating power supply is connected with the induction coil; and the PLC control system is connected with the induction heating power supply, and is configured to control the output power of the induction heating power supply to realize the automatic adjustment control of the temperature of the tensile test piece.
Owner:SHANDONG UNIV
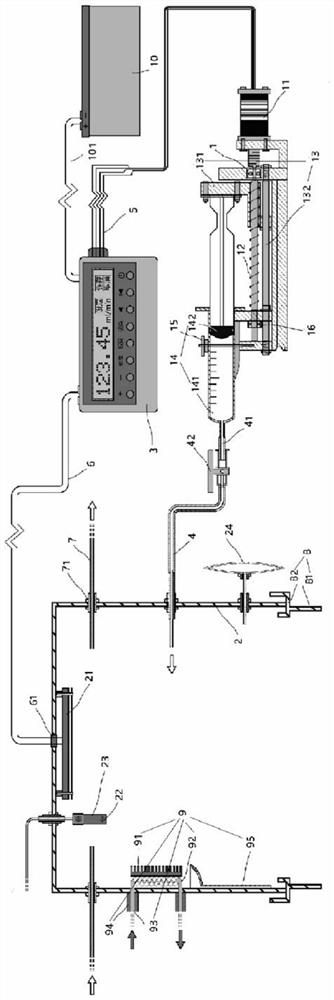
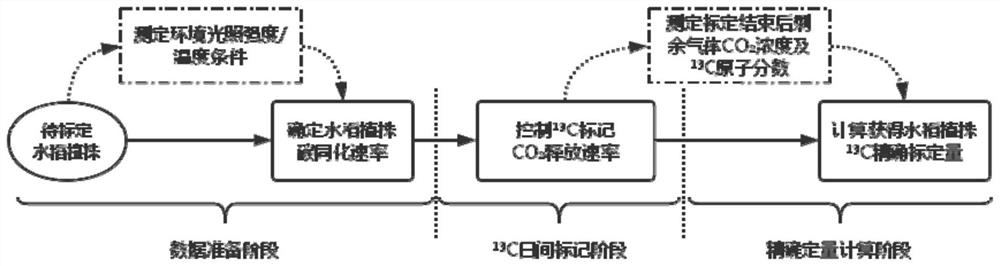
Rice plant carbon isotope field in-situ calibration method
PendingCN112180032AEasy speed controlEasy to controlTesting plants/treesRice plantElectric machinery
The invention provides a rice plant carbon isotope field in-situ calibration system, which comprises the following components: a carbon marker release device, which comprises a motor, a screw, a sliding member and an injector, wherein the motor is in transmission connection with the screw and is used for driving the screw to rotate, the sliding part is connected with the screw rod and the injectorand is used for sliding under the driving of the screw rod so as to extrude and release the carbon marker in the injector; a marking box, wherein the marking box is hollow, an opening is formed in the bottom of the marking box, and the marking box is communicated with the injector through a carbon marker release tube; and a motor controller connected with the motor through a first signal line andused for sending a signal for driving the screw to rotate to the motor. The invention further provides a rice plant carbon isotope field in-situ calibration method. According to the rice plant carbonisotope field in-situ calibration method, 13C in-situ calibration is conducted on rice plants under the field in-situ condition, the process is controllable, measurement is accurate, human interference is reduced, and the 13C marking cost is reduced.
Owner:SHANGHAI ACAD OF AGRI SCI
Method for measuring total amount of 2-phenyl-2-propanol in plastic and plastic products
The invention belongs to the technical field of chemical detection methods and relates to a method for measuring the total amount of 2-phenyl-2-propanol in plastic and plastic products. The method includes the following steps that a 2-phenyl-2-propanol standard stock solution is prepared; a 2-phenyl-2-propanol standard work solution is prepared; a work curve of the 2-phenyl-2-propanol standard work solution is prepared; extraction is carried out, plastic or plastic products to be measured are frozen and smashed into powder, dissolved in a toluene-acetone solution and placed in a microwave extraction tank, extraction liquid is transferred into a volumetric flask after microwave extraction, the extraction tank is flushed through a toluene-acetone solution, the solutions are combined, and volume is measured through a toluene-acetone solution; measurement is carried out through a gas chromatography-mass spectrometer under the selected analysis condition; the total amount of the 2-phenyl-2-propanol in the plastic and the plastic products is precisely measured. Operation is easy, accuracy is high, reproducibility is good, and actual detection requirements can be met.
Owner:INSPECTION & QUARANTINE TECH CENT SHANDONG ENTRY EXIT INSPECTION & QUARANTINE BUREAU
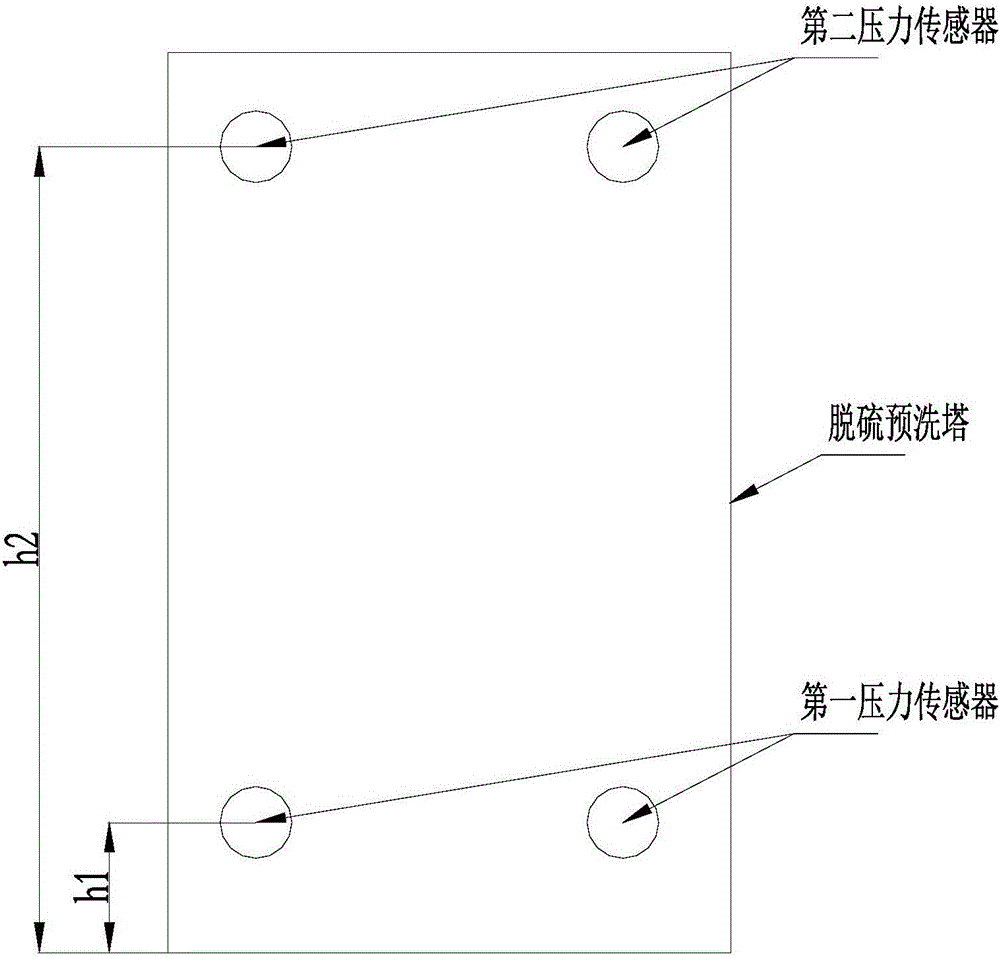
Method and system for measuring density of desulfurization slurry in power plant desulfurization pre-washing tower
InactiveCN105973750ASimple measuring deviceHigh measurement accuracySpecific gravity by measuring pressure differencesEngineeringAccuracy and precision
The invention relates to a method for measuring density of desulfurization slurry in a power plant desulfurization pre-washing tower. The method includes: measuring slurry pressures of the pre-washing tower at different horizontal heights, subtracting the measurements to obtain a slurry pressure difference P corresponding to a difference h of the different horizontal height, and acquiring density Rho of the desulfurization slurry in the pre-washing tower according to the formula: Rho = P / (gh). Also related to is a system for measuring density of desulfurization slurry in a power plant desulfurization pre-washing tower, and the density of desulfurization slurry is measured precisely using the method. The density of desulfurization slurry in the desulfurization pre-washing tower can be measured precisely through the system and method, measuring equipment is simplified, and measuring precision is improved; meanwhile, the system is simple in structure, the method is simple, and the system and method have significant effect and are suitable for popularization and use.
Owner:DATANG HANCHENG NO 2 POWER GENERATION
Accurate temperature measurement method for sand casting based on temperature measurement unit positioning
ActiveCN110044507AAchieving precise measurementsAchieve precise positioningThermometer detailsThermometers using electric/magnetic elementsCasting moldSand casting
The invention discloses a temperature measurement method for sand casting based on temperature measurement unit positioning. The method comprises steps of (1) manufacturing a temperature measurement unit by using a limiting block, a thermal couple and modeling sand, wherein (1-1), the limiting block comprises a positioning groove, the thermal couple is inserted into the positioning groove, modeling sand is used for filling and shaping on the outer surface of the limiting block, so as to form a sand block on the outer surface of the limiting block, the modeling sand is hardened, so as to obtaina temperature measurement unit precursor, (1-2), the limiting block is extracted out of the temperature measurement unit precursor, the position of the previous limiting block is filled with modelingsand, so as to form a temperature measurement side, the modeling sand is hardened, so as to obtain a temperature measurement unit; (2) making a casting mold by using the temperature measurement unitand the modeling sand; and (3) performing sand casting by using the casting mold, and measuring temperature of a to-be-measured temperature interface by using the thermal couple during sand casting. In the method, the temperature measurement unit is made by virtue of the limiting block and the sand block, the temperature measurement thermal couple in modeling sand can be positioned accurately, sothat temperature field change can be measured accurately in sand casting.
Owner:TSINGHUA UNIV
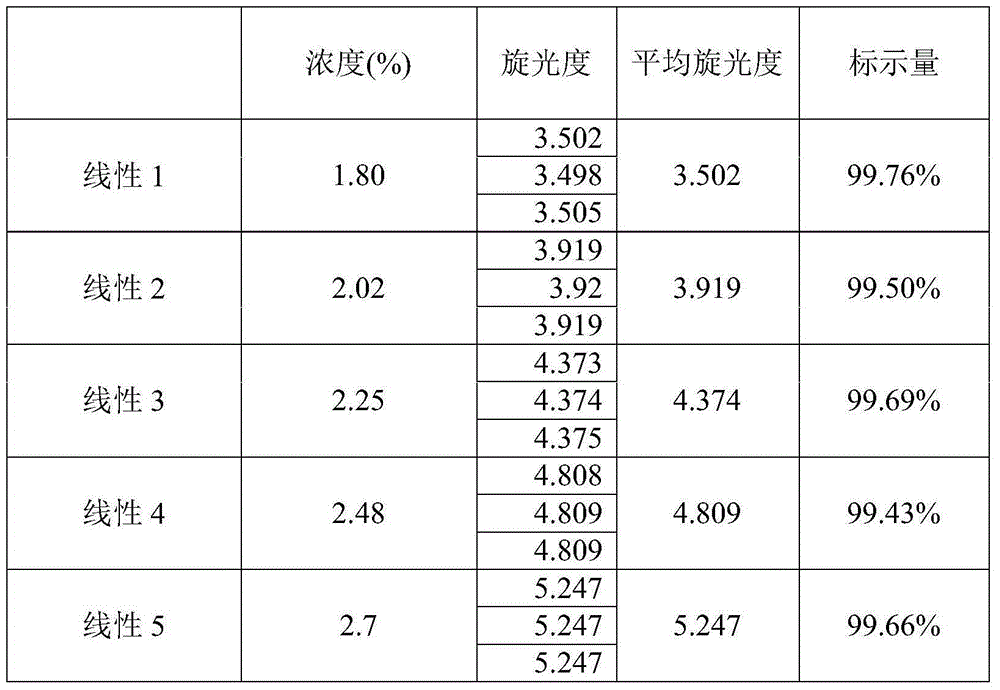
Method for determination of content of icodextrin in icodextrin peritoneal dialysis solution
InactiveCN106525727AAchieving precise measurementsThe determination method is simple and fastPolarisation-affecting propertiesSodium lactateDistilled water
The invention belongs to the technical field of medical testing, and relates to a method for determination of content of icodextrin in an icodextrin peritoneal dialysis solution, and the method comprises the following steps: taking 3ml of a to-be-measured icodextrin peritoneal dialysis solution sample into a 10ml measuring flask, adding distilled water to dilute to a scale; and measuring and calculating on the basis of a 0.134% sodium lactate solution as a blank according to a rotation determination method to obtain the content of the icodextrin in the icodextrin peritoneal dialysis solution, accurate determination of the content of the icodextrin in the icodextrin peritoneal dialysis solution can be achieved, and the method is simple and quick, can save manpower and material resources, is helpful for reduction of test cost, and improves product quality and safety.
Owner:HUAREN PHARMACEUTICAL CO LTD
Two-dimensional Hankel matrix multi-scale SVD transformation method
ActiveCN109901021AEliminate distractionsRealize accurate detectionFault locationTravel modeDecomposition
The embodiments of the invention disclose a two-dimensional Hankel matrix multi-scale SVD transformation method. The method includes the following steps that: S1, three-phase traveling wave data are collected, and modulus transformation is performed on the three-phase traveling wave data, and linear traveling wave mode signals are obtained, and the linear traveling mode signals are analyzed; S2, Xtransformation is performed on the traveling wave linear mode signals, and an approximate component Pj and a detail component Qj under the decomposition scale of a j-th layer are obtained; S3, a composite matrix Cj is constructed based on the S2, SVD transformation is performed on the Cj, a singular entropy corresponding to the decomposition layer is calculated, and a singular entropy increment deltaEj relative to an upper layer is calculated; if it is judged that the deltaEj is larger than a certain value epsilon, the approximate component Pj is assigned to a one-dimensional matrix X, and the step S2 is repeated, an optimal decomposition layer number r and detailed components Q1, Q2, ..., and Qr which are obtained after the decomposition of the first r layers are outputted; S4, dynamic threshold noise reduction processing is performed on a series of matrices Qi (i=1, 2, ..., and r) which are obtained after the r layers are decomposed, so that matrices which are represented by a symbol in the descriptions of the invention are obtained; and S5, transformation is performed on traveling wave signals X', and head wave and reflected wave time points are determined according to a singular point position in a traveling wave signal approximate component Qr which is obtained according to the decomposition of an r-th layer; and S6, a fault location interval is determined according to the head wave time of each monitor, and therefore, a fault point can be accurately located.
Owner:珠海妙微科技有限公司
Motor vehicle driving path obtaining method and system
InactiveCN110248312AAchieving precise measurementsAccurate and reliable measurement resultsParticular environment based servicesLocation information based serviceRoad networksEngineering
The invention discloses a motor vehicle driving path obtaining method, which is applied to the technical field of driving path detection, and comprises the following steps of setting a virtual base station in road sections on a road network based on a preset rule, and setting entity base stations on all other road sections connected with the road section where the virtual base station is located; setting a road section code for each road section in the road network, wherein the road section codes are base station codes on each road section and comprise virtual base station codes and entity base station codes; acquiring a first coding sequence containing an entity base station code on a driving road section based on a driving path of a motor vehicle, and obtaining a second coding sequence containing a virtual base station code based on the first sequence code; and obtaining a road section coding sequence based on the second coding sequence to obtain a driving path of the motor vehicle. The invention also discloses a system for acquiring the driving path of the motor vehicle. The method has the advantages that the number of base stations can be remarkably reduced while the running track of the motor vehicle can be accurately obtained, and therefore the cost needed by path detection is reduced.
Owner:GANSU WANHUA JINHUI INFORMATION TECH
Characteristic testing system of semiconductor laser
ActiveCN102109571BRealize measurementAchieving precise measurementsLaser detailsSemiconductor lasersPower semiconductor deviceTemperature control
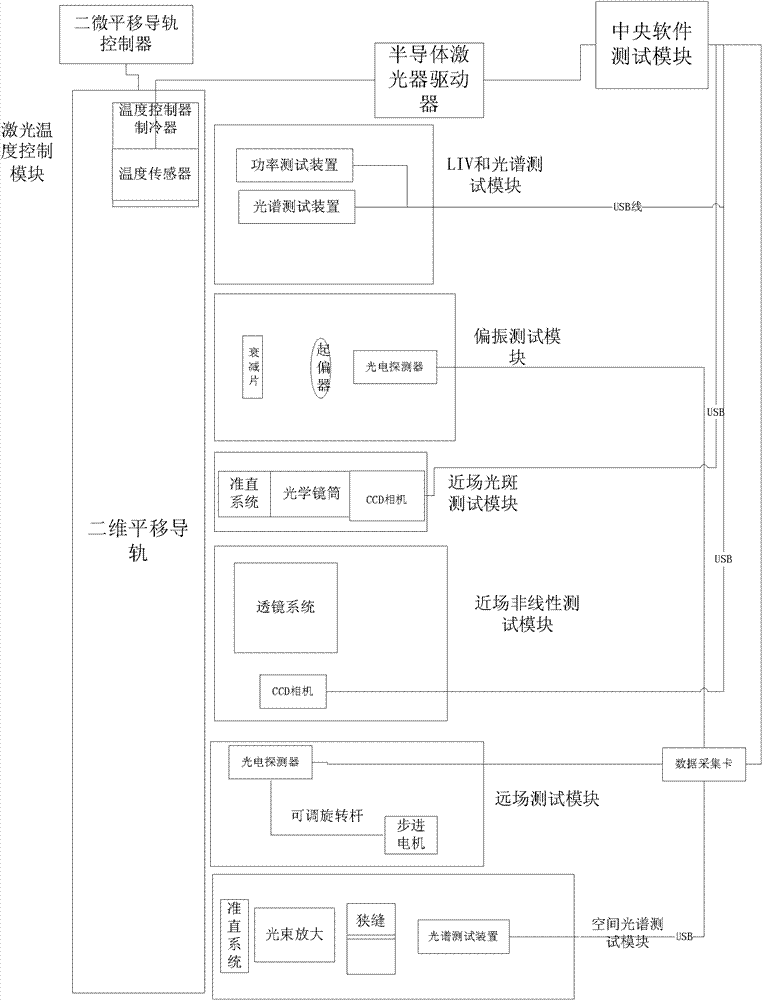
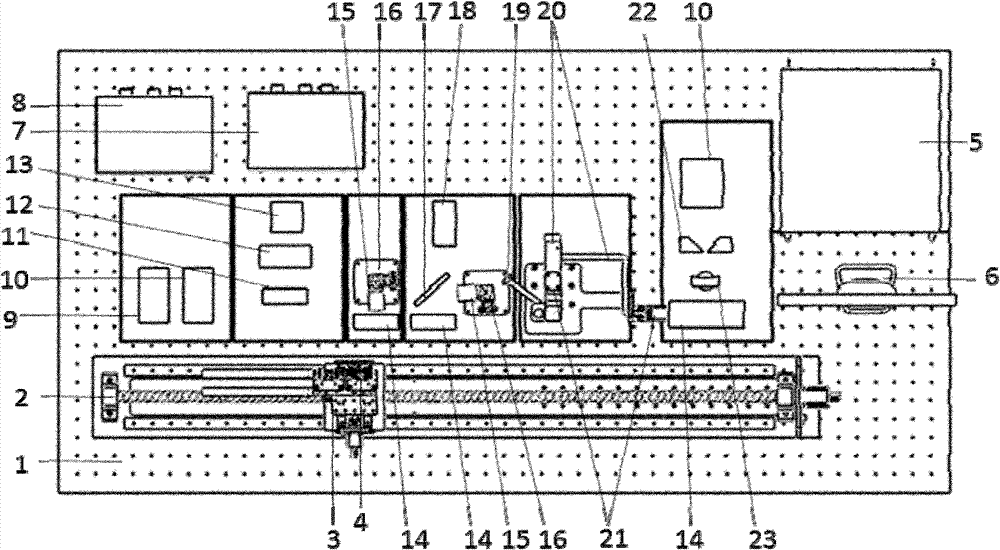
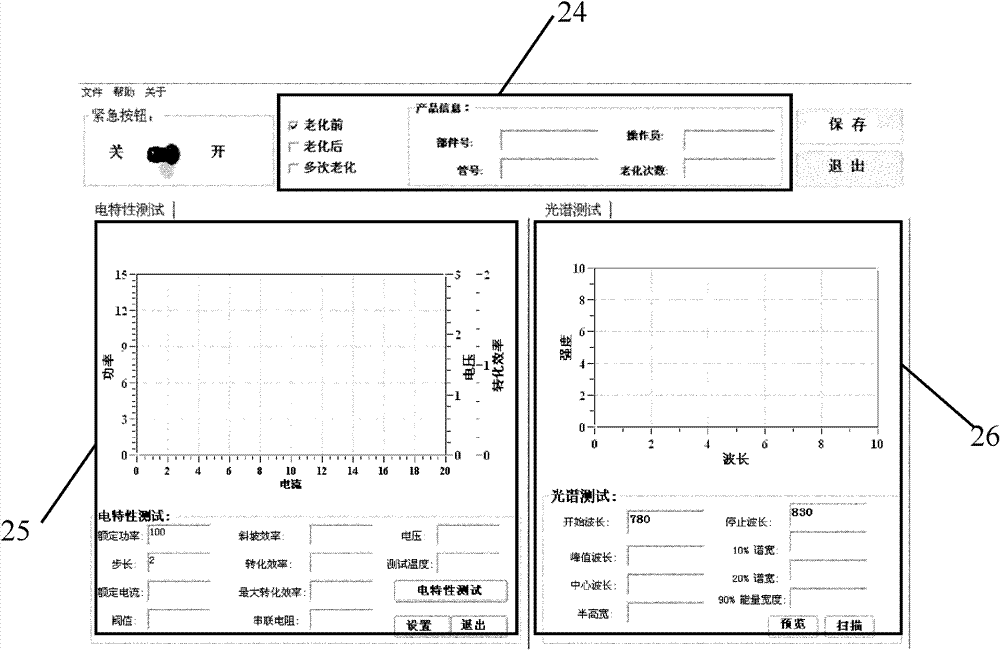
The invention discloses a characteristic testing system of a semiconductor laser, comprising a semiconductor laser driver, three-dimensional parallel guide rails, a laser temperature control module, an LIV (power-current-voltage) and spectrum test module, a polarization test module, a near-field light spot test module, a near-field nonlinear test module, a far-field test module, a space spectrum test module and a center software processing module. Working modules can be selected according to needs simultaneously. The characteristic testing system is used for high-accuracy and automation test of the semiconductor laser, especially for a high-power semiconductor laser, so that the cost for detection and manufacture of the semiconductor laser is reduced.
Owner:FOCUSLIGHT TECH
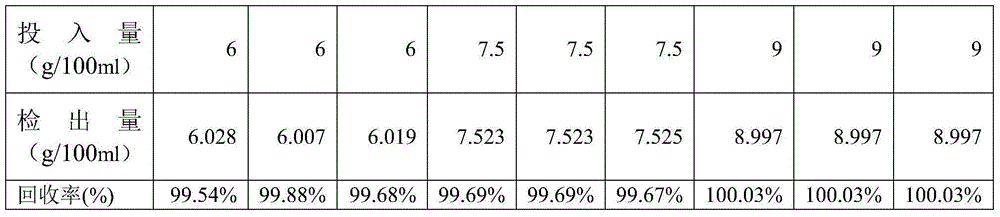
Dibutyl tin oxide detection method
InactiveCN101241111ASimple methodImprove detection accuracyComponent separationPreparing sample for investigationDibutyltin oxideSolvent
The present invention relates to a method for detecting content of dibutyltin oxide (DBTO) by chromatography exactly. Wherein, the dibutytin oxide and excessive 1-8 C alkyl derivatives grignard reagent are reacted under ultrasonic action, residual grignard reagent is destroyed by excessive acid, organic non-polar solvent is added to extract to obtain dibutyltin alkyl substitution which is insoluble in water and has lower boiling point, content of dibutyltin alkyl substitution is detected by organic phase chromatography. The dibutyltin oxide precision detection can be realized by equivalent conversion of dibutyltin alkyl substitution which can be analyzed by chromatography. The present invention is simple, feasible and the detection precision is high, the problem that no method in present can detect exactly the dibutyltin oxide content is solved.
Owner:JIANGSU YOKE TECH
Quantum weak measurement-based complex magneto-optical angle measurement system and method
ActiveCN113567351AEnables separate measurementsHigh sensitivityPolarisation-affecting propertiesMagnetic field measurement using magneto-optic devicesPerpendicular polarizationParticle physics
The invention discloses a quantum weak measurement-based complex magneto-optical angle measurement system and method. The measurement system comprises a light emitting device used for providing a light beam, a polarization state preparation device used for preparing plane polarization light, an electromagnetic field generation device used for providing a magnetic field, a phase adjusting device used for enabling two mutually perpendicular polarization components of the light beam to generate a pi / 2 phase difference, a time delay device used for adjusting the phase difference of two mutually perpendicular polarization components of the light beam, a polarization state detector used for projecting the light beam to a determined polarization state, and a photoelectric detector used for detecting and processing spectral distribution. By means of the measurement system, parameter separation can be conducted on a magneto-optical rotation angle and a magneto-optical ellipsometry, so that high-precision measurement of the magneto-optical rotation angle and the magneto-optical ellipsometry is achieved.
Owner:SICHUAN UNIV
Transmission and test system for signal-wavelength 3Gbps space high-speed light
InactiveCN102664676BAchieving precise measurementsSmooth transmissionFibre transmissionFree-space transmissionArea networkFlight vehicle
The invention discloses a transmission and test system for signal-wavelength 3Gbps space high-speed light. The system comprises an emitting end, a receiving end and double bidimensional adjustable overlaying light parallel adjusting devices for beacon light and signal light. The system is high in speed and safe, can be in seamless butted joint with the conventional optical fiber net and can be used for the condition that the high speed data transmission is required but an optical fiber system is difficult to pave, or can be used among area networks with high speed data rates, particularly be used among buildings, communication base stations, as well as between an aircraft and the ground, between an aircraft and an aircraft and among fighting units needing of fast networking; and be used for temporary interlinkage of disaster rescue.
Owner:XI'AN INST OF OPTICS & FINE MECHANICS - CHINESE ACAD OF SCI
Precise Temperature Measurement Method for Sand Casting Based on Positioning of Temperature Measurement Unit
ActiveCN110044507BAchieving precise measurementsAchieve precise positioningThermometer detailsThermometers using electric/magnetic elementsSoil scienceEngineering
The invention discloses a sand casting temperature measurement method based on the positioning of a temperature measurement unit. The method includes: (1) using a limiting block, a thermocouple and molding sand to make a temperature measuring unit: (1-1) the limiting block includes a positioning groove, by inserting the thermocouple into the positioning groove, and using the molding sand on the outer surface of the limiting block Carry out sand filling molding to form sand blocks on the outer surface of the limiting block; harden the molding sand to obtain the precursor of the temperature measuring unit; (1-2) take the limiting block out of the precursor of the temperature measuring unit, Fill the position of the block with molding sand to form a temperature measuring surface; harden the molding sand to obtain a temperature measuring unit; (2) use the temperature measuring unit and molding sand to make a mold; (3) use the mold for sand casting, the process of sand casting In this method, the temperature of the interface to be measured is measured by a thermocouple. In this method, the temperature measuring unit is made by means of the limiting block and the sand block, and the temperature measuring thermocouple in the molding sand can be precisely positioned, so as to realize the accurate measurement of the temperature field change in the sand casting process.
Owner:TSINGHUA UNIV
A stabilizing device that facilitates the measurement of the fuel injection quantity of the fuel injector
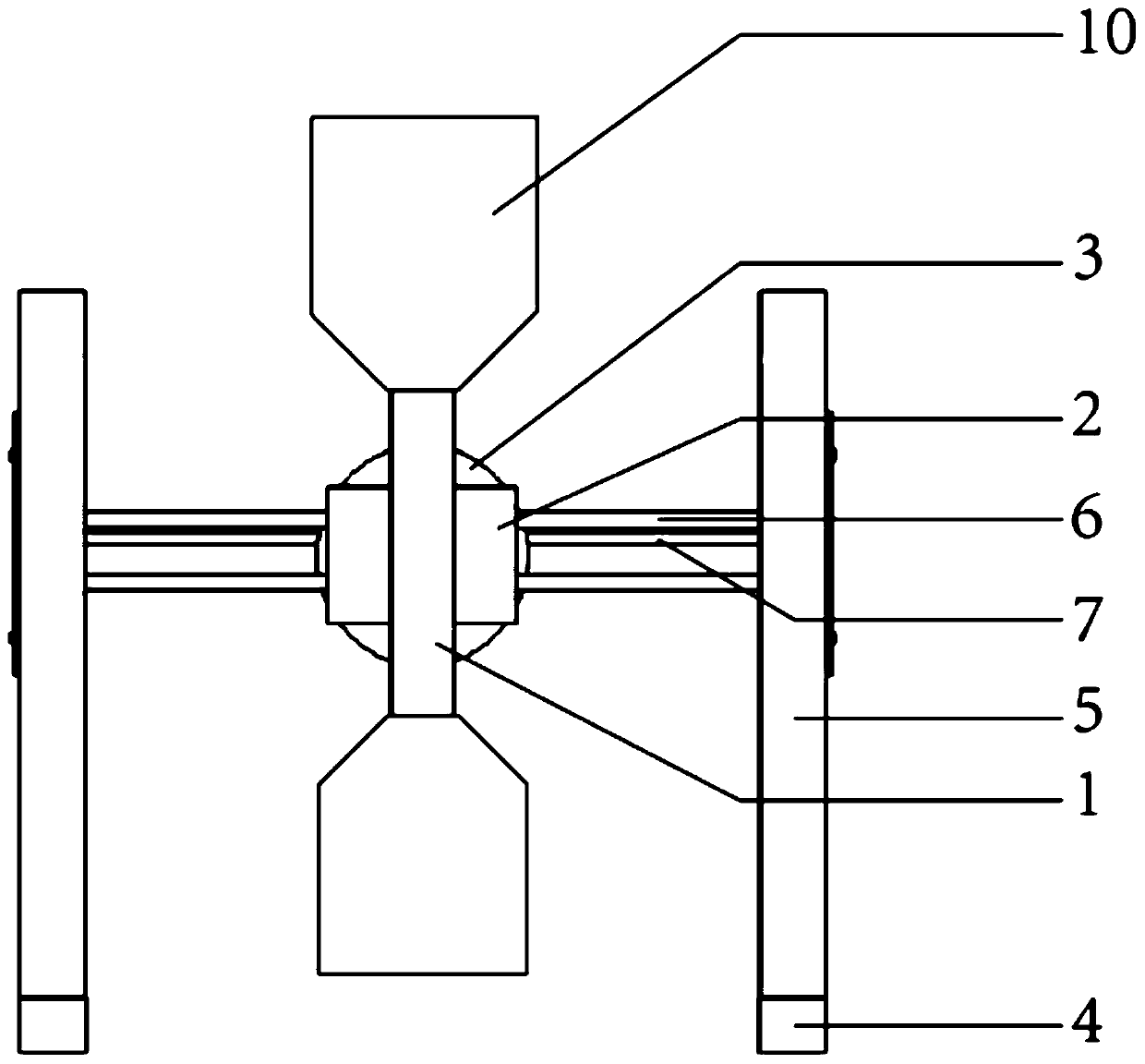
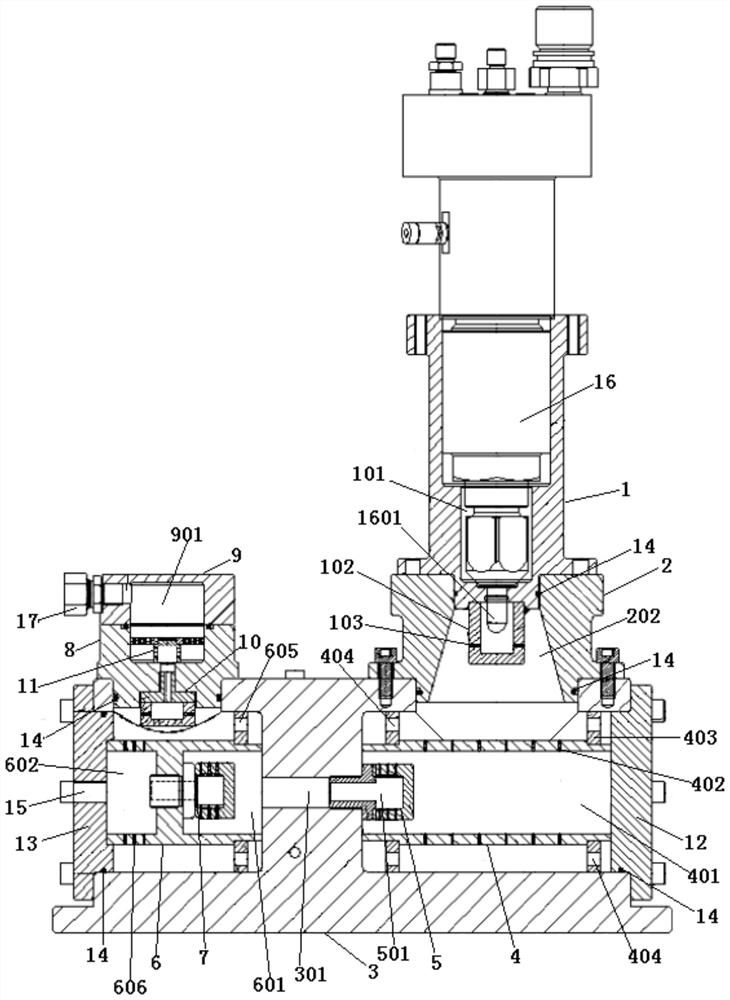
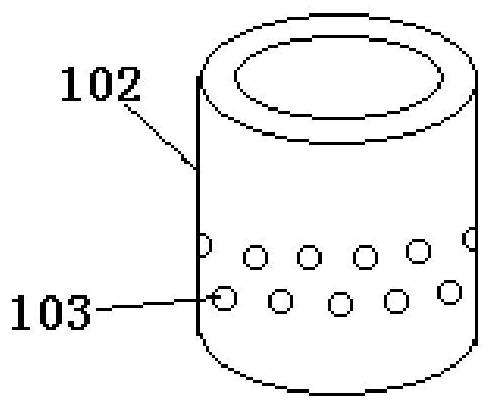
ActiveCN113153601BRelieve pressureAchieving precise measurementsEngine testingFuel injection apparatusEngineeringControl theory
Owner:CHONGQING HONGJIANG MACHINERY CO LTD
Teaching experiment system control module for mechanics of material
InactiveCN100365530CControl printer printingControl printingElectric signal transmission systemsComputer controlLaboratory deviceEngineering
Owner:山东省机电学校
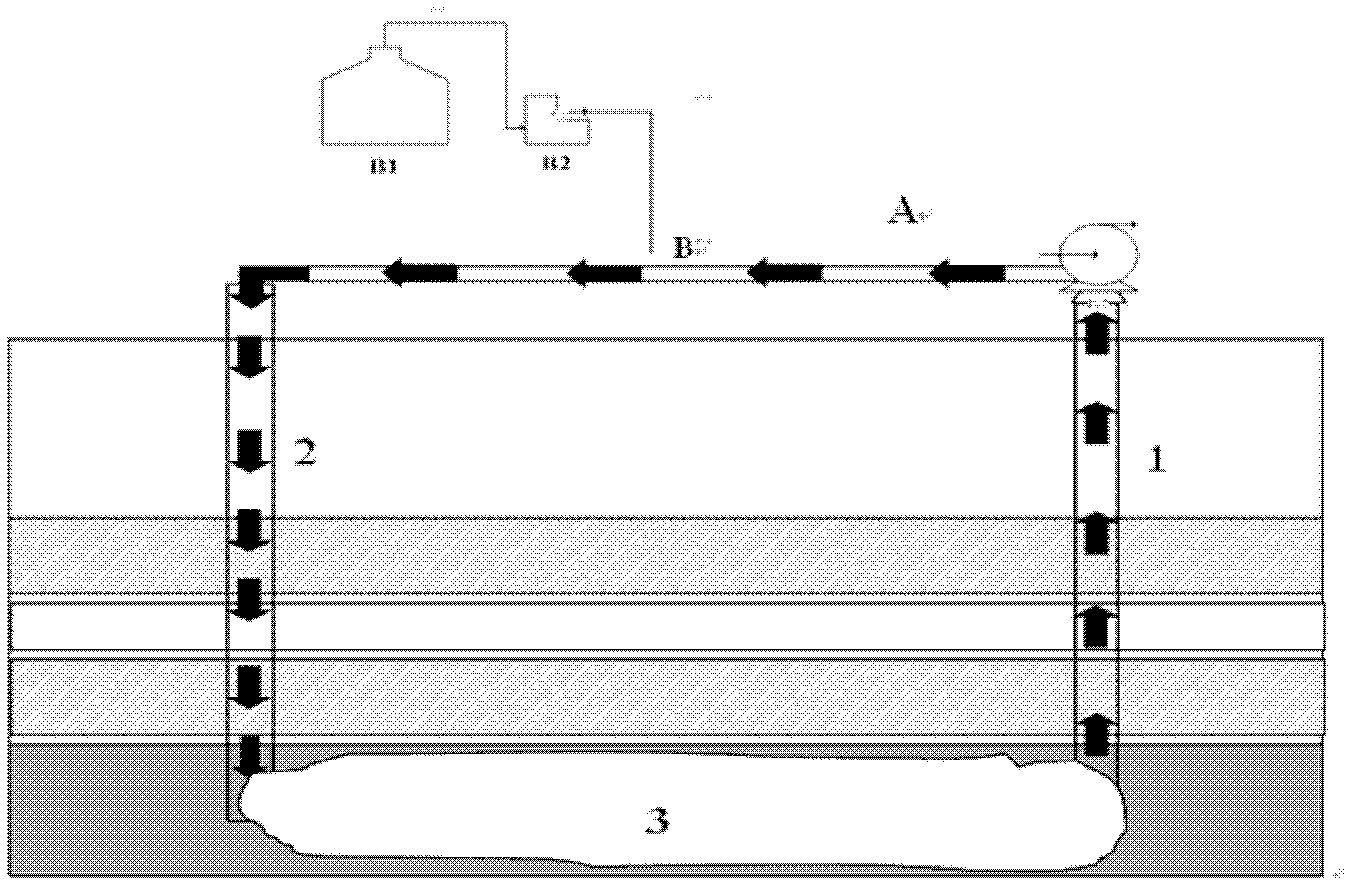
Underground cavity volume detection method and underground cavity volume detection device
ActiveCN102607663BAccurate measurementShort cycleContainer/cavity capacity measurementCombustionRepeatability
The invention belongs to the field of geology and detection, and relates to a method and a device for detecting the volume of an underground cavity. Specifically, the method for detecting the volume of an underground cavity includes the following steps: 1) filling the underground cavity with water or the underground cavity is already filled with water; Known amount is the detectable reagent of A0 mg; 3) make the detectable reagent in step 2) evenly distributed in water; 4) detect the concentration C' mg / L of the detectable reagent in water then; 5) according to the following formula Calculate the volume V' of the water filled in the underground cavity to obtain the volume V of the underground cavity: V=V'=A0 / C' / 1000m3. The invention also relates to a device for detecting underground cavities. The method of the invention can accurately measure the volume of the underground cavity, especially the burn-out area, and has the advantages of short period, no risk, good repeatability and simple process.
Owner:ENN SCI & TECH DEV
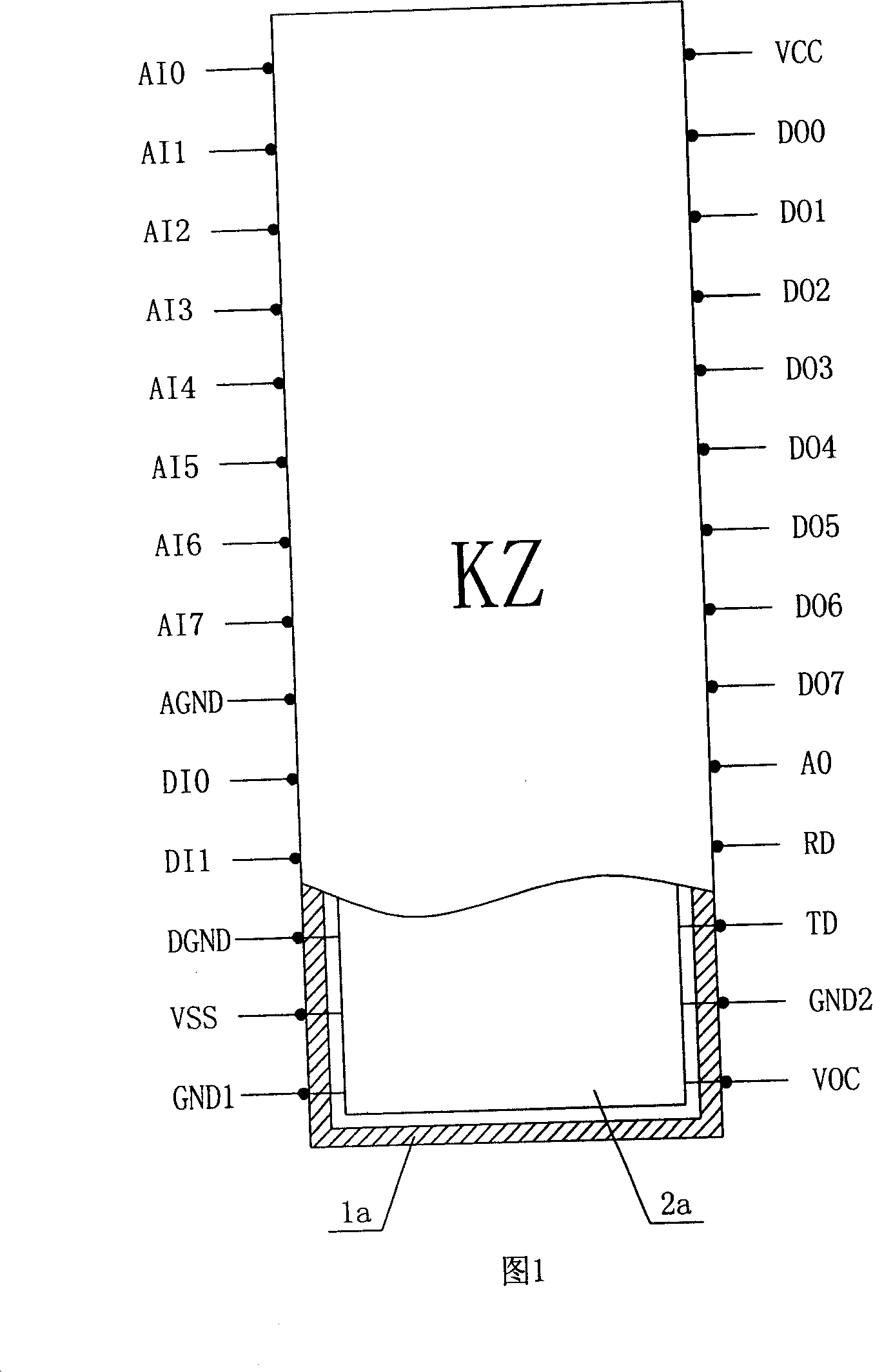
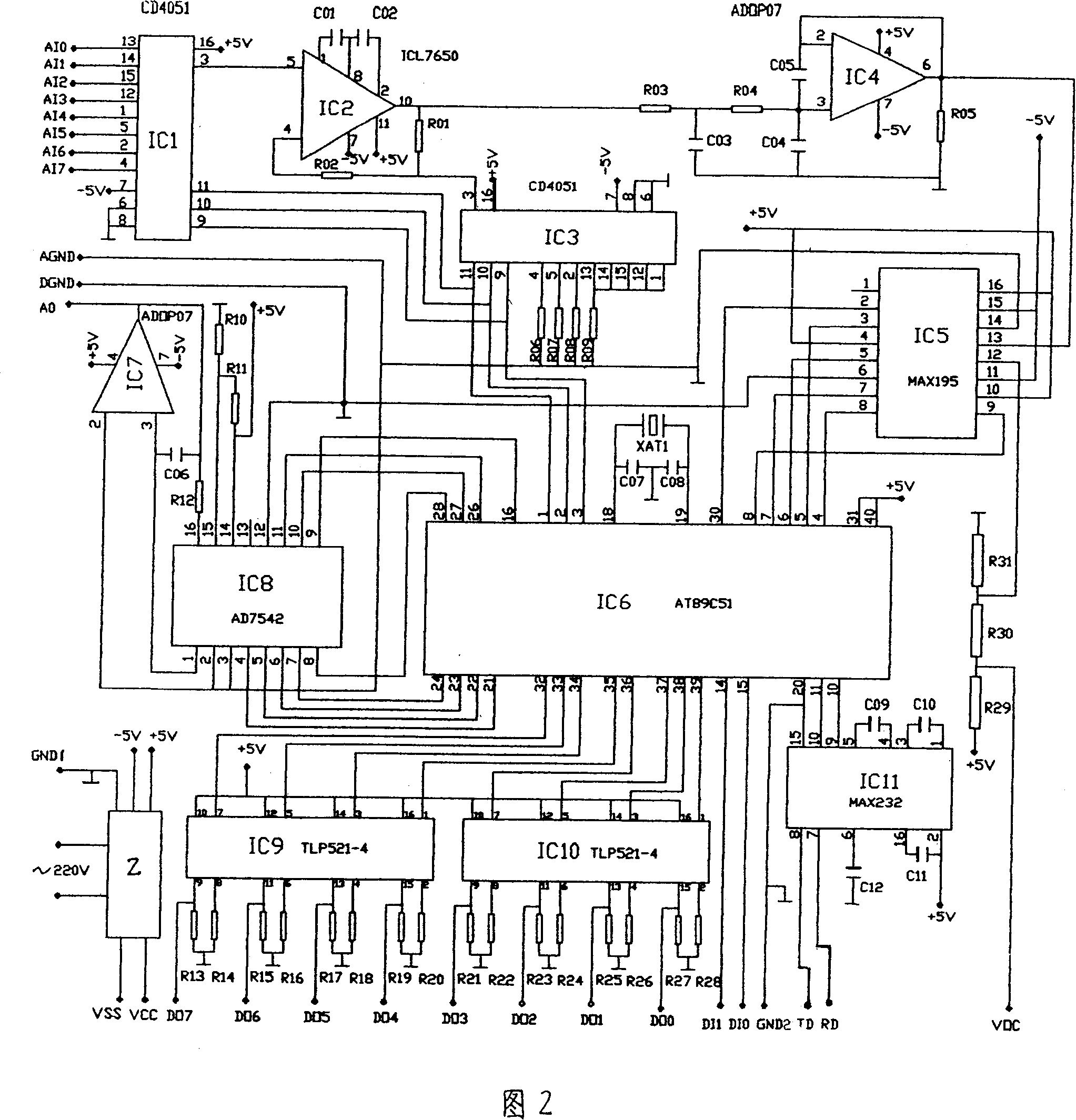
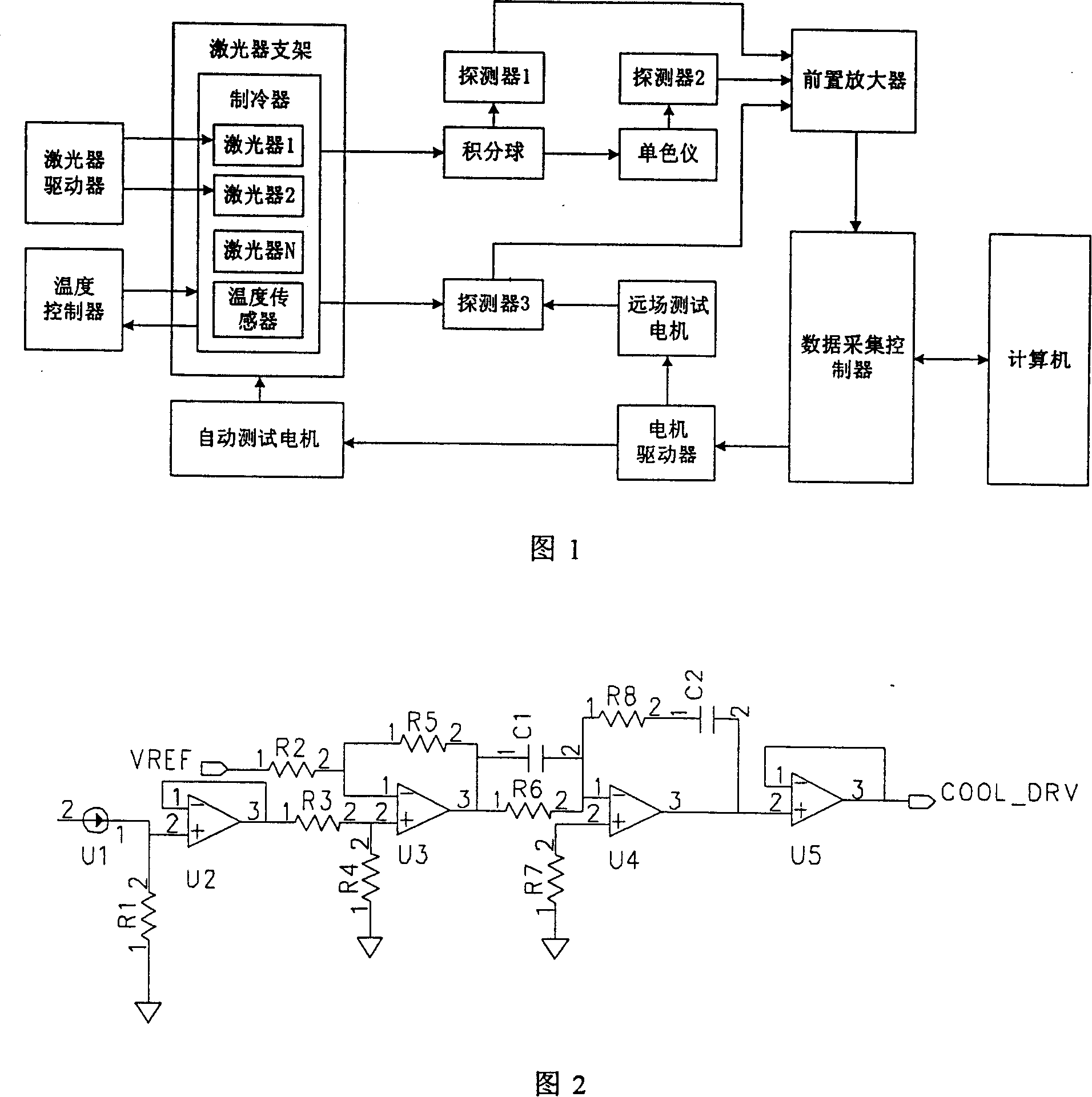
Semiconductor laser measuring device parameter
InactiveCN100371725CRealize automatic continuous measurementAchieving precise measurementsLaser detailsIndividual semiconductor device testingMeasurement deviceData acquisition
This invention relates to a test device for parameters of semiconductor lasers including: a laser driver connected with the laser, a temperature control system connected with a laser frame, a motor for mounting cards automatically connected with the frame, an integrating sphere connected with the laser to be tested, a detector connected with the integrating sphere, the laser to be tested and a monochromator, a monochromator connected with the integrating sphere and a data collection controller, a far-field test motor connected with the detector, a preamplifier connected with the data collection controller, a data collection controller connected with a computer and a computer connected with the controller, which can test various parameters of semiconductor lasers.
Owner:左昉 +3
Features
- R&D
- Intellectual Property
- Life Sciences
- Materials
- Tech Scout
Why Patsnap Eureka
- Unparalleled Data Quality
- Higher Quality Content
- 60% Fewer Hallucinations
Social media
Patsnap Eureka Blog
Learn More Browse by: Latest US Patents, China's latest patents, Technical Efficacy Thesaurus, Application Domain, Technology Topic, Popular Technical Reports.
© 2025 PatSnap. All rights reserved.Legal|Privacy policy|Modern Slavery Act Transparency Statement|Sitemap|About US| Contact US: help@patsnap.com